Delta shock as free piston in pressureless Euler flows

Abstract

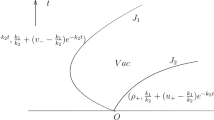
We establish the equivalence of free piston and delta shock, for the one-space-dimensional pressureless compressible Euler equations. The delta shock appearing in the singular Riemann problem is exactly the piston that may move freely forward or backward in a straight tube, driven by the pressureless Euler flows on two sides of it in the tube. This result not only helps to understand the physics of the somewhat mysterious delta shocks, but also provides a way to reduce the fluid–solid interaction problem, which consists of several initial-boundary value problems coupled with moving boundaries, to a simpler Cauchy problem. We show the equivalence from three different perspectives. The first one is from the sticky particles, and derives the ordinary differential equation (ODE) of the trajectory of the piston by a straightforward application of conservation law of momentum, which is physically simple and clear. The second one is to study a coupled initial-boundary value problem of pressureless Euler equations, with the piston as a moving boundary following the Newton’s second law. It depends on a concept of Radon measure solutions of initial-boundary value problems of the compressible Euler equations which enables us to calculate the force on the piston given by the flow. The last one is to solve directly the singular Riemann problem and obtain the ODE of delta shock by the generalized Rankine–Hugoniot conditions. All the three methods lead to the same ODE.

Appendix A: Solvability of piston’s trajectories

For convenience of readers, in this “Appendix”, we list the results on the six cases of the free piston problem. We omit the proof, since the details are the same as those in [15, pp. 67–72].

1.1 Appendix A.1: Case 1: $u_2<u_0<u_{1}$

Theorem 4

Suppose that $u_2<u_0<u_{1}$, then the free piston problem (1)–(5) admits a global solution, in which the trajectory of the piston satisfies (14). The velocity of the piston $x'_ {1}(t)\in (u_2,u_1)$ for any $t>0$, and it converges to a constant as $t\rightarrow +\infty$. There are concentration on both sides of the piston for all the time. Particularly, we have

(1) If  $\rho _{1}\ne \rho _{2}$,

$$\begin{aligned} x_{1}(t)=&\,\dfrac{-\sqrt{\rho _{1}\rho _{2}(u_{1}-u_{2})^{2}t^{2}+2m_{0} [\rho _{1}(u_{1}-u_{0})-\rho _{2}(u_{2}-u_{0})]t+m_{0}^{2}}}{\rho _{1}-\rho _{2}} \end{aligned}$$

(38)

$$\begin{aligned}&+\dfrac{(\rho _{1}u_{1}-\rho _{2}u_{2})t+m_{0}}{\rho _{1}-\rho _{2}}, \end{aligned}$$

(39)

$$\begin{aligned} \lim _{t\rightarrow +\infty } x_{1}'(t)=&\,\dfrac{-\sqrt{\rho _{1}\rho _{2}}(u_{1}-u_{2})+\rho _{1}u_{1} -\rho _{2}u_{2}}{\rho _{1}-\rho _{2}}=\frac{\sqrt{\rho }_1u_1+\sqrt{\rho _2}u_2}{\sqrt{\rho }_1+\sqrt{\rho _2}}; \end{aligned}$$

(40)

(2) If $\rho _{1}=\rho _{2}$,

$$\begin{aligned} x_{1}(t)=&\,\frac{u_1+u_2}{2}t+\frac{m_0^2(u_1+u_2 -2u_0)}{2\rho _1(u_1-u_2)[\rho _{1}(u_{1}-u_{2})t+m_{0}]} -\frac{m_0(u_1+u_2-2u_0)}{2\rho _1(u_1-u_2)}, \end{aligned}$$

(41)

$$\begin{aligned} \lim _{t\rightarrow +\infty } x_{1}'(t)=&\,\dfrac{u_{1}+u_{2}}{2}. \end{aligned}$$

(42)

1.2 Appendix A.2: Cases 2 and 3: $u_{1}>u_0$, $u_{2}>u_0$

For Case 3, we know the physical picture is as follows: there is a moment $t_1$, such that if $0< t<t_1$, there is a vacuum near the right side of the piston; as the flow on the left accelerates, the piston, it chases the constant moving flow $U_2=(\rho _2,u_2)^\top$ on the right, and at $t_1$ the piston meets $U_2$ and then concentration on the right side of the piston occurs; what happens next is the same as in Case 1. For Case 2, the story is a little bit different: the piston could never catch up with the right state $U_2$, and there is always vacuum near its right side. The following theorem verifies this.

Theorem 5

For the free piston problem (1)–(5), suppose that $u_{1}>u_0$, $u_{2}>u_0$, then it admits a global solution.

More specifically, for Case 2: $u_0<u_{1}<u_{2}$, there is always vacuum near the right surface of the piston, namely the piston will never catch up with the right-side moving flows with state $(\rho _2, u_2)^{\top }$, and the trajectory of the left surface of the piston is

$$\begin{aligned} x_{1}(t)=\dfrac{-\sqrt{2m_{0}\rho _{1}(u_{1}-u_{0})t+m_{0}^{2}}}{\rho _{1}}+u_{1}t +\dfrac{m_{0}}{\rho _{1}}. \end{aligned}$$

(43)

The limiting velocity of the piston is

$$\begin{aligned} \lim _{t\rightarrow +\infty }x_{1}'(t)=u_{1}. \end{aligned}$$

(44)

For Case 3: $u_0<u_{2}<u_{1}$, $x_1(t)$ is given by

$$\begin{aligned} x_{1}(t)={\left\{ \begin{array}{ll} \dfrac{-\sqrt{2m_{0}\rho _{1}(u_{1}-u_{0})t+m_{0}^{2}}}{\rho _{1}}+u_{1}t +\dfrac{m_{0}}{\rho _{1}}, &{}\text {if }0\le t\le t_{1},\\ \tilde{x}_1(t-t_1)+u_2t_1,&{} \text {if }t_1<t, \end{array}\right. } \end{aligned}$$

(45)

where $\displaystyle t_1\doteq \frac{2m_0(u_2-u_0)}{\rho _1(u_1-u_2)^2}$, and $\tilde{x}_1(\cdot )$ is the function given by (38) if $\rho _1\ne \rho _2$, and by (41) if $\rho _1= \rho _2$, while in both of which, $m_0$ is replaced by $\alpha (t_1)$ defined in (36) and $u_0$ is replaced by $\displaystyle \frac{2u_1u_2-u_0u_2-u_0u_1}{u_1+u_2-2u_0}$. The limiting velocity of the piston is given by (40) and (42), respectively.

1.3 Appendix A.3: The other cases

As discussed in Sect. 2.3, Case 4 and Case 5 ($u_{1}<u_0$, $u_{2}<u_0$) are in essence the same as Cases 2 and 3 studied above. So we will not repeat the results any more. For the last Case 6, namely $u_{1}\le u_0\le u_{2}$, the pressureless gas moves uniformly away from the piston, and there are vacuum near the piston and the piston’s trajectory is given by $x_1(t)=u_0t,\ \forall \, t\ge 0$. The solution for this case is trivial.