Skip to main content
SpringerLink
Log in

Large deformation shape uncertainty quantification in acoustic scattering

  • Published:
Advances in Computational Mathematics Aims and scope Submit manuscript

Abstract

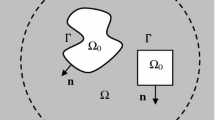
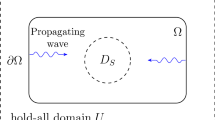
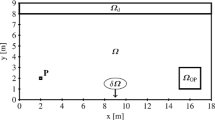
We address shape uncertainty quantification for the two-dimensional Helmholtz transmission problem, where the shape of the scatterer is the only source of uncertainty. In the framework of the so-called deterministic approach, we provide a high-dimensional parametrization for the interface. Each domain configuration is mapped to a nominal configuration, obtaining a problem on a fixed domain with stochastic coefficients. To compute surrogate models and statistics of quantities of interest, we apply an adaptive, anisotropic Smolyak algorithm, which allows to attain high convergence rates that are independent of the number of dimensions activated in the parameter space. We also develop a regularity theory with respect to the spatial variable, with norm bounds that are independent of the parametric dimension. The techniques and theory presented in this paper can be easily generalized to any elliptic problem on a stochastic domain.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Allaire, G., Jouve, F., Toader, A.-M.: A level-set method for shape optimization. C.R. Math. 334, 1125–1130 (2002)

    Article  MathSciNet  Google Scholar 

  2. Babuška, I., Nobile, F., Tempone, R.: A stochastic collocation method for elliptic partial differential equations with random input data. SIAM Rev. 52, 317–355 (2010)

    Article  MathSciNet  Google Scholar 

  3. Berenger, J.-P.: A perfectly matched layer for the absorption of electromagnetic waves. J. Comput. Phys. 114, 185–200 (1994)

    Article  MathSciNet  Google Scholar 

  4. Bieri, M., Andreev, R., Schwab, C.: Sparse tensor discretization of elliptic SPDEs. SIAM J. Sci. Comput. 31, 4281–4304 (2009)

    Article  MathSciNet  Google Scholar 

  5. Brenner, S.C., Scott, R.: The mathematical theory of finite element methods, vol. 15 Springer Science & Business Media (2008)

  6. Calvi, J.-P., Manh, P.: Lagrange interpolation at real projections of Leja sequences for the unit disk. Proc. Am. Math. Soc. 140, 4271–4284 (2012)

    Article  MathSciNet  Google Scholar 

  7. Canuto, C., Kozubek, T.: A fictitious domain approach to the numerical solution of PDEs in stochastic domains. Numerische mathematik 107, 257–293 (2007)

    Article  MathSciNet  Google Scholar 

  8. Castrillon-Candas, J.E., Nobile, F., Tempone, R. F.: Analytic regularity and collocation approximation for PDEs with random domain deformations. Comput. Math. Appl. 71, 1173–1197 (2016)

    Article  MathSciNet  Google Scholar 

  9. Chkifa, A., Cohen, A., Schwab, C.: High-dimensional adaptive sparse polynomial interpolation and applications to parametric PDEs. Found. Comput. Math. 14, 601–633 (2014)

    Article  MathSciNet  Google Scholar 

  10. Chkifa, A.: Breaking the curse of dimensionality in sparse polynomial approximation of parametric PDEs. Journal de Mathé,matiques Pures et Appliquées 103, 400–428 (2015)

    Article  MathSciNet  Google Scholar 

  11. Chkifa, M.A.: On the Lebesgue constant of Leja sequences for the complex unit disk and of their real projection. J. Approx. Theory 166, 176–200 (2013)

    Article  MathSciNet  Google Scholar 

  12. Cohen, A., DeVore, R., Schwab, C.: Convergence rates of best N-term Galerkin approximations for a class of elliptic sPDEs. Found. Comput. Math. 10, 615–646 (2010)

    Article  MathSciNet  Google Scholar 

  13. Cohen, A.: Analytic regularity and polynomial approximation of parametric and stochastic elliptic PDEs. Anal. Appl. 9, 11–47 (2011)

    Article  MathSciNet  Google Scholar 

  14. Cohen, A., Schwab, C., Zech, J.: Shape Holomorphy of the stationary Navier-Stokes Equations, Report 2016-45, Seminar for Applied Mathematics, ETH Zürich, Switzerland. to appear in SIAM J. Math. Analysis (2016)

  15. Collino, F., Monk, P.: The perfectly matched layer in curvilinear coordinates. SIAM J. Sci. Comput. 19, 2061–2090 (1998)

    Article  MathSciNet  Google Scholar 

  16. Colton, D., Kress, R.: Inverse acoustic and electromagnetic scattering theory, vol. 93 Springer Science & Business Media (2012)

  17. Dambrine, M., Harbrecht, H., Puig, B.: Computing quantities of interest for random domains with second order shape sensitivity analysis, ESAIM. Math. Model. Numer. Anal. 49, 1285–1302 (2015)

    Article  MathSciNet  Google Scholar 

  18. Dick, J., Gantner, R.N., Le Gia, Q.T., Schwab, C.: Multilevel higher-order quasi-Monte Carlo Bayesian estimation. Math. Models Methods Appl. Sci. 27, 953–995 (2017)

    Article  MathSciNet  Google Scholar 

  19. Eigel, M., Gittelson, C.J., Schwab, C., Zander, E.: Adaptive stochastic Galerkin FEM. Comput. Methods Appl. Mech. Eng. 270, 247–269 (2014)

    Article  MathSciNet  Google Scholar 

  20. Gantner, R.N.: A generic C++ library for multilevel Quasi-Monte Carlo. In: Proceedings of the Platform for Advanced Scientific Computing Conference, PASC ’16, pp. 11:1–11:12. ACM, New York (2016)

  21. Gantner, R.N., Peters, M.D.: Higher order Quasi-Monte Carlo for Bayesian shape inversion, Report 2016-45, Seminar for Applied Mathematics, ETH Zürich, Switzerland (2016)

  22. Gantner R.N., Schwab, C.: Computational higher order Quasi-Monte Carlo integration. In: Cools R., Nuyens, D. (eds.) Monte Carlo and Quasi-Monte Carlo Methods: MCQMC, Leuven, Belgium, April 2014, pp. 271–288. Springer International Publishing, Cham (2016)

    Google Scholar 

  23. Gerstner, T., Griebel, M.: Dimension–adaptive tensor–product quadrature. Computing 71, 65–87 (2003)

    Article  MathSciNet  Google Scholar 

  24. Ghanem, R.G., Spanos, P.D.: Stochastic finite elements: a spectral approach Courier Corporation (2003)

  25. Gilbarg, D., Trudinger, N.S.: Elliptic partial differential equations of second order, vol. 224 Springer Science & Business Media (2001)

  26. Griebel, M., Oettershagen, J.: On tensor product approximation of analytic functions. J. Appr. Theory 207, 348–379 (2016)

    Article  MathSciNet  Google Scholar 

  27. Harbrecht, H., Li, J.: First order second moment analysis for stochastic interface problems based on low-rank approximation. ESAIM: Math. Model. Numer. Anal. 47, 1533–1552 (2013)

    Article  MathSciNet  Google Scholar 

  28. Harbrecht, H., Peters, M., Siebenmorgen, M.: Analysis of the domain mapping method for elliptic diffusion problems on random domains. Numer. Math. 134, 823–856 (2016)

    Article  MathSciNet  Google Scholar 

  29. Harbrecht, H., Schneider, R., Schwab, C.: Sparse second moment analysis for elliptic problems in stochastic domains. Numer. Math. 109, 385–414 (2008)

    Article  MathSciNet  Google Scholar 

  30. Hiptmair, R., Sargheini, S.: Scatterers on the Substrate: Far field formulas, Report 2015-02, Seminar for Applied Mathematics, ETH Zürich, Switzerland (2015)

  31. Horn, R.A., Johnson, C.R.: Topics in matrix analysis. Cambridge University Press, Cambridge (1991)

    Book  Google Scholar 

  32. Jerez-Hanckes, C., Schwab, C., Zech, J.: Electromagnetic wave scattering by random surfaces: Shape holomorphy. Math. Mod. Meth. Appl. Sci. 27, 2229–2259 (2017)

    Article  MathSciNet  Google Scholar 

  33. Lassas, M., Somersalo, E.: On the existence and convergence of the solution of PML equations. Computing 60, 229–241 (1998)

    Article  MathSciNet  Google Scholar 

  34. Li, J., Melenk, J.M., Wohlmuth, B., Zou, J.: Optimal a priori estimates for higher order finite elements for elliptic interface problems. Appl. Numer. Math. 60, 19–37 (2010)

    Article  MathSciNet  Google Scholar 

  35. Li, R., Tang, T., Zhang, P.: Moving Mesh Methods in Multiple Dimensions Based on Harmonic Maps. J. Comput. Phys. 170, 562–588 (2001)

    Article  MathSciNet  Google Scholar 

  36. McLean, W.C.H.: Strongly elliptic systems and boundary integral equations. Cambridge University Press, Cambridge (2000)

    MATH  Google Scholar 

  37. Monk, P., Süli, E.: The adaptive computation of far-field patterns by a posteriori error estimation of linear functionals. SIAM J. Numer. Anal. 36, 251–274 (1998)

    Article  MathSciNet  Google Scholar 

  38. Nédélec, J.-C.: Acoustic and electromagnetic equations: integral representations for harmonic problems, vol. 144 Springer Science & Business Media (2001)

  39. Nouy, A., Clement, A., Schoefs, F., Moës, N.: An extended stochastic finite element method for solving stochastic partial differential equations on random domains. Comput. Methods Appl. Mech. Eng. 197, 4663–4682 (2008)

    Article  MathSciNet  Google Scholar 

  40. Osher S., Fedkiw, R.P.: Level set methods: An overview and some recent results. J. Comput. Phys. 169, 463–502 (2001)

    Article  MathSciNet  Google Scholar 

  41. Paganini, A., Scarabosio, L., Hiptmair, R., Tsukerman, I.: Trefftz approximations: a new framework for nonreflecting boundary conditions. IEEE Trans. Magn. PP, 1–1 (2015)

    Google Scholar 

  42. Scarabosio, L.: Shape uncertainty quantification for scattering transmission problems, PhD thesis, ETH Zürich, 2016. Diss. No. 23574. http://e-collection.library.ethz.ch/eserv/eth:49652/eth-49652-02.pdf

  43. Schillings, C., Schwab, C.: Sparse, adaptive Smolyak quadratures for Bayesian inverse problems. Inverse Prob. 29, 065011 (2013)

    Article  MathSciNet  Google Scholar 

  44. Schillings, C., Schwab, C.: Sparsity in Bayesian inversion of parametric operator equations. Inverse Prob. 30, 065007 (2014)

    Article  MathSciNet  Google Scholar 

  45. Schwab, C., Gittelson, C.J.: Sparse tensor discretizations of high-dimensional parametric and stochastic PDEs. Acta Numer. 20, 291–467 (2011)

    Article  MathSciNet  Google Scholar 

  46. Tartakovsky, D.M., Xiu, D.: Stochastic analysis of transport in tubes with rough walls. J. Comput. Phys. 217, 248–259 (2006)

    Article  MathSciNet  Google Scholar 

  47. Wiener, N.: The homogeneous chaos. Amer. J. Math. 60(4), 897–936 (1938)

    Article  MathSciNet  Google Scholar 

  48. Xiu, D., Hesthaven, J.S.: High-order collocation methods for differential equations with random inputs. SIAM J. Sci. Comput. 27, 1118–1139 (2005)

    Article  MathSciNet  Google Scholar 

  49. Xiu, D., Tartakovsky, D.M.: Numerical methods for differential equations in random domains. SIAM J. Sci. Comput. 28, 1167–1185 (2006)

    Article  MathSciNet  Google Scholar 

  50. Zech, J., Schwab, C.: Convergence rates of high dimensional Smolyak quadrature, Report 2017-27, Seminar for Applied Mathematics, ETH Zürich, Switzerland (2017)

Download references

Acknowledgments

Research supported by ERC under Grant AdG247277 and by ETH under CHIRP Grant CH1-02 11-1.

Author information

Authors and Affiliations

  1. Seminar for Applied Mathematics, D-MATH, ETH Zurich, Zurich, Switzerland

    R. Hiptmair & Ch. Schwab

  2. Department of Mathematics, Chair of Numerical Mathematics, Technical University of Munich, Munich, Germany

    L. Scarabosio

  3. Institut für Mathematik, Fakultät für Wirtschaftsinformatik & Wirtschaftsmathematik, Universität Mannheim, Mannheim, Germany

    C. Schillings

Authors
  1. R. Hiptmair
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. L. Scarabosio
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. C. Schillings
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ch. Schwab
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to L. Scarabosio.

Additional information

Communicated by: Ivan Graham

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hiptmair, R., Scarabosio, L., Schillings, C. et al. Large deformation shape uncertainty quantification in acoustic scattering. Adv Comput Math 44, 1475–1518 (2018). https://doi.org/10.1007/s10444-018-9594-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10444-018-9594-8

Keywords

Mathematics Subject Classification (2010)

Search

Navigation