Abstract
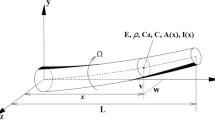
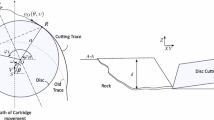
This paper constructs a theoretical framework to investigate the stress and deformation state within the body of a roller disc cutter from the cutter characteristics (radii of the shaft and disc and the length of the shaft), the characteristics of the cut (depth of cut), the mechanical properties of the constructive material (Poisson’s ratio and Young modulus) and the boundary conditions involved during the disc cutting process. To pursue the objectives, mathematical formulations and calculations required for the analysis of elastic variables induced in the disc and shaft parts of a roller disc cutter subjected to general cutting forces are investigated. Assuming elastic material behaviour, the disc cutter in contact with its shaft is considered as a partially loaded annulus, and the shaft inside the disc as a laterally loaded cylinder subjected to stresses corresponding to different cutting configurations. The Airy stress function, Michell’s method and Papkovich–Neuber solutions are employed in two- and three-dimensional elastic domains using a double Fourier series technique.



















Similar content being viewed by others
References
Hudson JA, Harrison JP (1997) Engineering rock mechanics: an introduction to the principles. Pergamon, Oxford
Maidl B, Schmid L, Ritz W, Herrenknecht M (2008) Hardrock tunnel boring machines. Wiley, Berlin
Guan Z (1997) Mechanics of rock/tool/machine interaction in disc rock cutting. PhD thesis, The University of Queensland, School of Engineering, Brisbane, Australia
Alehossein H, Boland JN, Chen L, Lim W, Korinets A, Hood M (1995) Prediction of rock failure in rock indentation by a rock cutter. Technical Report. CMTE-HDRK, MM3–95/2, Division of Exploration and Mining, CSIRO, Australia, August 1995
Alehossein H, Hood M (1996) State of the art review of rock models for disc roller cutters. In: 2nd North American rock mechanics symposium (NARMS) on rock mechanics, tools and techniques. Balkema Publications, Montreal, pp 693–700
Roxborough FF, Phillips HR (1975) Rock excavation by disc cutter. Int J Rock Mech Min Sci Geomech Abstr 12(12):361–366
Cho JW, Jeon S, Yu SH, Chang SH (2010) Optimum spacing of TBM disc cutters: a numerical simulation using the three-dimensional dynamic fracturing method. Tunn Undergr Space Technol 25(3):230–244
Zhu LD, Jijiang W, Liu CG, Liu WW, Shuai X (2013) Research on simulation process of rock broken by TBM hob. Adv Mater Res 712–715:633–636
Moon T, Oh J (2012) A study of optimal rock-cutting conditions for hard rock TBM using the discrete element method. Rock Mech Rock Eng 45:837–849
Yu B, Khair AW (2007) Numerical modeling of rock ridge breakage in rotary cutting. In: ARMA General Meeting, Vancouver
Cook NGW, Hood M, Tsai F (1984) Observations of crack growth in hard rock loaded by an indenter. Int J Rock Mech Min Sci Geomech Abstr 21(2):97–107
Pang SS, Goldsmith W, Hood M (1989) A force-indentation model for brittle rocks. Rock Mech Rock Eng 22(2):127–148
Huang H, Damjanac B, Detournay E (1997) Numerical modeling of normal wedge indentation in rocks with lateral confinement. Int J Mech Min Sci 34(3–4): Paper No. 064
Alehossein H, Detournay E, Huang H (2000) An analytical model for the indentation of rocks by blunt tools. Rock Mech Rock Eng 33(4):267–284
Chen LH, Labuz JF (2006) Indentation of rock by wedge-shaped tools. Int J Rock Mech Min Sci 43(7):1023–1033
Eubanks RA, Sternberg E (1956) On the completeness of the Boussinesq–Papkovich stress functions. J Ration Mech Anal 5(5):735–746
Sadd MH (2009) Elasticity: theory, applications, and numerics, 2nd edn. Elsevier, Academic Press, Boston
Barber JR (2010) Elasticity, 2nd edn. Springer, Berlin
Timoshenko SP, Goodier JN (1969) Theory of elasticity, 3rd edn. McGraw-Hill, New York
Little RW (1973) Elasticity. Civil engineering and engineering mechanics series. Prentice Hall, Englewood Cliffs
Serati M, Alehossein H, Williams DJ (2013) 3D elastic solutions for laterally loaded discs: generalised Brazilian and point load tests. Rock Mech Rock Eng 47(4):1087–1101
Michell JH (1899) On the direct determination of stress in an elastic solid, with application to the theory of plates. Proc Lond Math Soc 31(1):100–124
Serati M, Alehossein H, Williams DJ (2012) Elastic stress analysis of partially loaded hollow discs. Int J Eng Sci 53:19–37
Youngdahl CK (1969) On the completeness of a set of stress functions appropriate to the solution of elasticity problems in general cylindrical coordinates. Int J Eng Sci 7(1):61–79
Steven GP (1977) A non-axisymmetric cylindrical contact problem. Int J Eng Sci 15(2):95–103
Chiu YP, Weinstein AS, Zorowski CF (1964) Elastic behaviour of a cylinder subjected to a biaxially symmetric normal surface loading. Q J Mech Appl Math 17(2):199–215
Yao JC (1969) Long cylindrical tube subjected to two diametrically opposite loads. Aeronaut Q 20:365–381
Abdulaliyev Z, Ataoglu S (2009) Effect of Poisson’s ratio on three-dimensional stress distribution. J Appl Mech 76(1):014506
Alehossein H, Serati M (2011) FEM numerical study of wedge roller discs on hard rock cutting. Technical Report. CSIRO Internal Report, Brisbane
Serati M, Alehossein H, Williams DJ (2012) Analytical and numerical study of hard rock cutting with roller disc cutters. In: IMECE 2012: ASME international mechanical engineering congress and exposition, Huston, 9–15 Nov 2012, pp 1947–1954
Simmonds JG (1994) A brief on tensor analysis, 2nd edn. Springer, New York
Shima H, Nakayama T (2010) Higher mathematics for physics and engineering, 7th edn. Springer, Berlin
Lai WM, Rubin D, Krempl E (2009) Introduction to continuum mechanics, 4th edn. Butterworth-Heinemann/Elsevier, Philadelphia
Arfken GB, Weber HJ, Harris FE (2011) Mathematical methods for physicists: a comprehensive guide, 7th edn. Elsevier Science, Cambridge
Alehossein H (1996) Fundamentals of engineering analysis—Advanced Graduate Course E9871. University of Queensland Press, Faculty of Engineering, Physical Sciences and Architecture
Moon P, Spencer DE (1988) Field theory handbook, including coordinate systems, differential equations, and their solutions, 2nd edn. Springer, New York
Boyde L, Ekpenyong A, Whyte G, Guck J (2013) Elastic theory for the deformation of a solid or layered spheroid under axisymmetric loading. Acta Mech 224(4):819–839
Acknowledgments
The first author wishes to acknowledge two scholarships, granted by CSIRO and UQ: (i) Minerals Down Under Flagship Scholarship of CSIRO (CESRE), and (ii) UQ Postgraduate Scholarship from the School of Civil Engineering at UQ.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix: Elastic field variables in partially loaded hollow discs with \(\alpha =\beta \)
Stress components for a particular case of a partially loaded annulus when \(\alpha =\beta \) discussed in [23] are further verified as follows:
where
Tensor calculus in general curvilinear coordinates
In a generalised curvilinear coordinate system, \(\text {Y}\) identified by its covariant and contravariant local basis vectors \(\varvec{e}_{i}\) and \(\varvec{e}^{i}\), respectively, the process of the divergence (\(\nabla \cdot \)), del (\(\nabla \)) and Laplacian (\(\nabla ^2\)) operators, operating possibly on either a scalar (\(\phi \)) or a vector (\(\varvec{u}\)), is guaranteed if they satisfy the following generic rules and relations: [31–35]:
where \(\delta ^{i}_{j}\) is the Kronecker delta, \(\Gamma \) is the familiar Christoffel symbol, \(g_{ij}\) are the components of the coordinate covariant metric tensor, \(g=\text {det}[g_{ij}]\), \(i,j,k,l,m=1,2,3\), and the Einstein’s summation convention is applied. However, it should be noted that \(\varvec{e}^i\) (or \(\varvec{e}_i\)) in Eqs. (76)–(83) does not generally result in basis vectors with unitary length, as occurs in Cartesian coordinates. Coordinate scale factors (\(h_i\)) are thus to be used to normalise both covariant and contravariant vector components independent of their length, so that \(\hat{\varvec{e}}\) is the normalised unit vector form of \(\varvec{e}\), viz. Therefore, the gradient Eq. (76) and divergence Eq. (78) can also take the following forms:
Solutions of Laplace’s equation in cylindrical polar coordinates
In order to find the general solution of Laplace’s relation in Eqs. (38) and (39), the method of separation of variables can be employed to reduce the resultant PDEs to ODEs [34, 36]. The Laplacian of a harmonic scalar \(\phi \) in cylindrical coordinates is obtained by solving the following linear partial differential equation:
It follows that \(\phi \) takes either the form
or
Here, \(I_m(\zeta _n r)\) and \(K_m(\zeta _n r)\) denote the modified Bessel function of the first and the second kinds of order m and the non-negative argument \(\zeta _n r\), respectively; \(J_m(\zeta _n r)\) is the Bessel function of the first kind; \(Y_m(\zeta _n r)\) is the Bessel function of the second kind; and \(A_{n}\), \(A_m,\ldots \) are the undetermined integral constants for each m and n. It should be pointed out that the terms \(K_m(\zeta _n r)\) and \(Y_m(\zeta _n r)\) are singular at the origin and must be discarded for bounded domains. However, for problems in which the boundary conditions are given by Fourier series, e.g. in the form of Eq. (52), the former general solution in Eq. (87) to the Laplace equation (86) is eventually accepted. For special cases of a solid cylinder with the origin included, this becomes
In the same manner and remembering that the Cartesian components \(\psi _x\), \(\psi _y\) and \(\psi _z\) of a harmonic vector \(\varvec{\psi }{(x,y,z)}\) are all harmonic [21, 25, 37], the Laplace operator on non-harmonic components, \(\psi _r\) and \(\psi _\theta \), in cylindrical coordinates is related to the appropriate Laplace equation on Cartesian components according to the geometric relationships between the two systems and the classical principles of conversion of a vector from rectangular to cylindrical coordinates. This means,
With Eqs. (90) and (91) in mind, acceptable trial functions of \(\psi _r\) and \(\psi _\theta \) can be investigated to satisfy the distribution of any prescribed field variables given for a problem. For instance, if the hoop stress (\(\sigma _{\theta \theta }\)) of a target problem has only \(\sin (\zeta _n z)\sin (m\theta )\) terms at \(r=r_0\), suitable choices of \(\psi _r\) and \(\psi _\theta \) would be
where \(f(r)_{mn}\), \(g(r)_{mn}\) are only functions of r for each m and n. The reason for selecting such trial functions is seen in Eq. (42), in which \(\psi _r\) and its first derivative with respect to r (\(\psi _{r,\,r}\)) and its second derivative with respect to \(\theta \) (\(\psi _{r,\,\theta \theta }\)) all participate in calculating the hoop stress. In contrast, only the first derivative of \(\psi _\theta \) with respect to \(\theta \) contributes to the hoop stress formulation. Consequently, if the trial functions of \(\psi _r\) and \(\psi _\theta \) defined in Eqs. (92) and (93) are substituted in Eq. (42), it can be readily checked that the same target trigonometric terms of \(\sin (\zeta _n z)\sin (m\theta )\) are obtained. Equation (90) therefore yields
Since \(\nabla ^2\psi _x=0\), the solution to Eq. (94) is recognised as various forms of modified Bessel’s equation leading to
where \(A^{\prime }_{mn}\), \(A^{\prime \prime }_{mn},\cdots \) are yet unknown arbitrary integration constants to be determined from a given boundary condition, and \(K(\zeta _nr)\) should be excluded for the case of a bounded problem. Substituting Eqs. (95) and (96) into Eqs. (92) and (93) gives
In a similar fashion, acceptable vector components \(\psi _r\) and \(\psi _\theta \) for bounded problems are determined in the general forms of Eqs. (99) and (100).
Rights and permissions
About this article
Cite this article
Serati, M., Alehossein, H. & Williams, D.J. Theoretical treatment of disc cutters subjected to general cutting forces. J Eng Math 100, 141–165 (2016). https://doi.org/10.1007/s10665-015-9839-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10665-015-9839-1
Keywords
- Double Fourier series technique
- Michell’s method
- Papkovich–Neuber functions
- Rock cutting
- Roller disc cutter