Hodograph transformation for crack-tip fields in hyperelastic sheets: higher order eigenmodes and asymptotic path-independent integrals

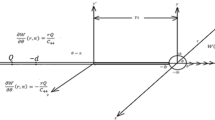
Abstract

Hodograph transformations can be used to linearize a nonlinear partial differential equation by judicious use of physical quantities (e.g. velocities or displacement gradients) as coordinate variables in the hodograph plane. This approach has been found useful for obtaining the leading order terms of eigenproblems that govern asymptotic singular crack fields in nonlinear materials. There is little work on the use of the hodograph transformation for obtaining higher order terms in the asymptotic expansion of the crack tip fields. In this paper, we develop a framework to obtain such higher order terms using the hodograph transformation. The method relies heavily on the representation of physical quantities of interest in terms of hodograph plane variables. We demonstrate the method via application to a generalized neo-Hookean material. In addition, asymptotic path-independent J-integrals are expressed in terms of either physical or hodograph variables and are used to compute the leading-order amplitude coefficients. A relationship between the asymptotic J-integrals and the energy release rate is established for a mixed crack mode. The asymptotic results are compared with numerical results from finite element computation and excellent agreement is obtained.

Appendix A: Solution of higher order eigenmodes of a GNH material

Here, we show that the first three order eigenmodes in Eq. (67) obtained by the hodograph transformation are the same as those in (Geubelle and Knauss 1994). We start with the first order eigenmode given by

$$\begin{aligned} f_1(\theta ;\,n)= & {} [n(w+k\, \text {cos}\theta )]^{\frac{1}{2}-\frac{1}{2n}}\nonumber \\&\sin \frac{\theta }{2}\left( 1-\frac{2k^{2}\, \cos ^{2}\, \frac{\theta }{2}}{1+w}\right) ^{\frac{1}{2}}\nonumber \\ \end{aligned}$$

(A.1)

where

$$\begin{aligned} k=1-\frac{1}{n},\, w=\sqrt{1-k^{2}\, \sin ^{2}\,\theta } \end{aligned}$$

(A.2)

To obtain Eq. (A.1) from $(m_{1} -1)C_{1} R^{m_{1} }\cos \vartheta$ in Eq. (65), we need to use Eq. (69) and following relations

$$\begin{aligned}&(w+k\, \cos \theta )(w-k\,\cos \theta )\nonumber \\&\quad =1-k^{2} =\frac{2n-1}{n^{2}}, \, (\hbox {Eq. }(A.2)) \nonumber \\ \end{aligned}$$

(A.3)

$$\begin{aligned}&\frac{1}{2}(w-\cos \theta )\nonumber \\&\quad =\sin ^{2}\frac{\theta }{2}\left( 1-\frac{2k^{2}\,\cos ^{2}\,\frac{\theta }{2}}{1+w} \right) , \, (\hbox {Eq. }(A.2)) \nonumber \\ \end{aligned}$$

(A.4)

and

$$\begin{aligned} {\cos ^2}\vartheta = \frac{1}{{2n}}\left( {1 - wn\cos \theta + nk\,{{\cos }^2}\theta } \right) , \, (\hbox {Eq. }(69)) \nonumber \\ \end{aligned}$$

(A.5)

Using these relations, we can further show

$$\begin{aligned}&(4{n^2} - 4n){\cos ^2}\vartheta + 1 \nonumber \\&\quad = {n^2}{(w - k\cos \theta )^2} \nonumber \\&\quad = \frac{{{{(2n - 1)}^2}}}{{{n^2}}}{(w + k\cos \theta )^{ - 2}}, \,(\hbox {Eqs. }(A.2) \hbox { and } (A.5)) \nonumber \\ \end{aligned}$$

(A.6)

and

$$\begin{aligned} {\cos ^2}\vartheta= & {} \frac{1}{2}\frac{n}{{2n - 1}}(w - k\cos \theta )(w - \cos \theta ),\nonumber \\&\,(\hbox {Eqs. }(A.2)\hbox { and } (A.5)) \end{aligned}$$

(A.7)

From the above relations, it can be shown that the square of the first order eigenmode of y becomes

$$\begin{aligned}&(m_{1} -1)^{2}C_{1}^{2} R^{2m_{1} }\cos ^{2}\vartheta \nonumber \\&\quad =4n^{2}C_{1}^{\tfrac{1}{n}} r^{2-\tfrac{1}{n}}\left[ {(4n^{2}-4n)\cos ^{2}\vartheta +1} \right] ^{-1+\tfrac{1}{2n}}\nonumber \\&\qquad \cos ^{2}\vartheta ,\, (\hbox {Eqs. }(64)\hbox { and }(69)) \nonumber \\&\quad =4n^{2}C_{1}^{\tfrac{1}{n}} r^{2-\tfrac{1}{n}}\left[ {\tfrac{(2n-1)^{2}}{n^{2}}(w+k\cos \theta )^{-2}} \right] ^{-1+\tfrac{1}{2n}}\nonumber \\&\qquad \cos ^{2}\vartheta ,\, (\hbox {Eq. }(A.6))\nonumber \\&\quad =4n^{2}C_{1}^{\tfrac{1}{n}} r^{2-\tfrac{1}{n}}\left( {\tfrac{2n-1}{n}} \right) ^{-2+\tfrac{1}{n}}\nonumber \\&\qquad \tfrac{2n-1}{n^{2}}(w+k\cos \theta )^{1-\tfrac{1}{n}}(w-k\cos \theta )^{-1}\nonumber \\&\qquad \cos ^{2}\vartheta ,\, (\hbox {Eq. }(A.3))\nonumber \\&\quad =4n^{2}C_{1}^{\tfrac{1}{n}} r^{2-\tfrac{1}{n}}\left( {\tfrac{2n-1}{n}} \right) ^{-2+\tfrac{1}{n}}\nonumber \\&n^{-1}n^{-1+\tfrac{1}{n}}[n(w+k\cos \theta )]^{1-\tfrac{1}{n}}\nonumber \\&\qquad \sin ^{2}\frac{\theta }{2}\left( {1-\frac{2k^{2} \cos ^{2}\tfrac{\theta }{2}}{1+w}} \right) ,\nonumber \\&\qquad (\hbox {Eqs. } (A.7)\hbox { and }(A.4))\nonumber \\&\quad =4C_{1}^{\tfrac{1}{n}} r^{2-\tfrac{1}{n}}\left( {2n-1} \right) ^{-2+\tfrac{1}{n}}n^{2}[n(w+k\cos \theta )]^{1-\tfrac{1}{n}}\nonumber \\&\qquad \sin ^{2}\frac{\theta }{2}\left( {1-\frac{2k^{2}\cos ^{2}\tfrac{\theta }{2}}{1+w}} \right) \nonumber \\&\quad = {p^2}{r^{2 - \frac{1}{n}}}f_1^2(\theta ;\;n) \end{aligned}$$

(A.8)

where

$$\begin{aligned} {p^2} = 4{n^2}\,C_1^{{\frac{1}{n}}}\left( {2n - 1} \right) ^{ - 2 + \frac{1 }{n}} \end{aligned}$$

(A.9)

It can be verified that $f_{1} (\theta ;\;n)$ is the same as the expression given in Eq. (A.1), and p reduces to $p=2C_{1}^{\tfrac{1}{2}}$ for $n=1$, i.e., Eq. (50).

To obtain the higher order eigenmodes, we can first show that

$$\begin{aligned}&(w - k\cos \theta )(1 - k{\sin ^2}\theta - w\cos \theta ) \nonumber \\&\quad = (1 + k)(w - \cos \theta ),\, (\hbox {Eq. }(A.2)) \end{aligned}$$

(A.10)

and

$$\begin{aligned} {\cos ^2}\zeta= & {} \frac{{n^2}}{2{{(2n - 1)}^2}}{(w - k\cos \theta )^2}\nonumber \\&(1 - k{\sin ^2}\theta - w\cos \theta )\nonumber \\= & {} \frac{{n^2}}{2{{(2n - 1)}^2}}(w - k\cos \theta )(1 + k)(w - \cos \theta ) \nonumber \\&(\hbox {Eq. }(A.10), (A.2)\hbox { and }(A.7)) \nonumber \\= & {} \frac{n}{2(2n - 1)}(w - k\cos \theta )(w - \cos \theta )\nonumber \\= & {} \cos ^{2}\, \vartheta \end{aligned}$$

(A.11)

where $\cos \zeta$ is an intermediate variable in the coordinate transformation (Knowles and Sternberg 1974; Geubelle and Knauss 1994). Thus, using Eqs. (A.6), (A.10) and (A.11), we obtain the second order eigenmode

$$\begin{aligned}&({m_2} - 1)C_2^{}{R^{{m_2}}}\cos 2\vartheta \nonumber \\&\quad = ({m_2} - 1)C_2^{}C_1^{{m_2}/2n}{r^{ - {m_2}/2n}}\nonumber \\&\qquad \left[ {{{(4}}{n^2} - 4n){{\cos }^2}\vartheta + 1} \right] ^{{m_2}/4n}(2\cos ^{2}\vartheta - 1)\nonumber \\&\quad = ({m_2} - 1)C_2^{}C_1^{{m_2}/2n}{r^{ - {m_2}/2n}}\nonumber \\&\qquad {\left[ {{\textstyle {{{{(2n - 1)}^2}} \over {{n^2}}}}{{(w + k\cos \theta )}^{ - 2}}} \right] ^{{m_2}/4n}}\nonumber \\&\qquad (2\cos ^{2}\zeta - 1)\nonumber \\&\quad = ({m_2} - 1)C_2^{}C_1^{{m_2}/2n}{(2n - 1)^{{m_2}/2n}}{r^{ - {m_2}/2n}}\nonumber \\&\quad {\left[ {n(w + k\cos \theta )} \right] ^{ - {m_2}/2n}}\nonumber \\&\qquad (2\cos ^{2}\zeta - 1)\nonumber \\&\quad = q{r^{ - {m_2}/2n}}{\left[ {n(w + k\cos \theta )} \right] ^{ - {m_2}/2n}}\nonumber \\&\qquad (2\cos ^{2}\zeta - 1) \end{aligned}$$

(A.12)

where

$$\begin{aligned} q = ({m_2} - 1)C_2^{}C_1^{{m_2}/2n}{(2n - 1)^{{m_2}/2n}} \end{aligned}$$

(A.13)

Equation (A.12) is the same as Eq. (4.22) in (Geubelle and Knauss 1994). In a similar way, we can show shat the third order eigenmode in the physical plane satisfies

$$\begin{aligned}&({m_3} - 1)C_3^{}{R^{{m_3}}}\cos 3\vartheta \nonumber \\&\quad = s \cdot {r^{ - {m_3}/2n}}{\left[ {n(w + k\cos \theta )} \right] ^{ - {m_3}/2n}}\nonumber \\&\quad (4\cos ^{3}\zeta - 3\cos \zeta ) \end{aligned}$$

(A.14)

where

$$\begin{aligned} s = ({m_3} - 1)C_3^{}C_1^{{m_3}/2n}{(2n - 1)^{{m_3}/2n}} \end{aligned}$$

(A.15)

Equation (A.14) is the same as Eq. (3.35) in (Geubelle and Knauss 1994). Thus, we show that all the three (and beyond, if desired) eigenmodes can be obtained by the hodograph transformation.