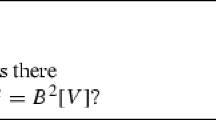Skip to main content
SpringerLink
Log in

The Common Face of some 0/1-Polytopes with NP-Complete Nonadjacency Relation

  • Published:
Journal of Mathematical Sciences Aims and scope Submit manuscript

Abstract

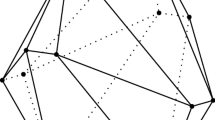
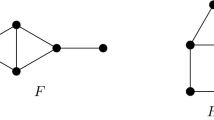
In this paper, we consider so-called double covering polytopes. In 1995, Matsui showed that the problem of checking nonadjacency on these polytopes is NP-complete. We show that double covering polytopes are faces of the following polytopes: knapsack polytopes, set covering polytopes, cubic subgraph polytopes, 3-SAT polytopes, partial order polytopes, traveling salesman polytopes, and some others.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. A. Y. Alfakih and K. G. Murty, “Adjacency on the constrained assignment problem,” Discrete Appl. Math., 87, No. 1, 269–274 (1998).

    Article  MATH  MathSciNet  Google Scholar 

  2. L. J. Billera and A. Sarangarajan, “All 0-1 polytopes are traveling salesman polytopes,” Combinatorica, 16, No. 2, 175–188 (1996).

    Article  MATH  MathSciNet  Google Scholar 

  3. V. A. Bondarenko and A. N. Maksimenko, Geometric Constructions and Complexity in Combinatorial Optimization [in Russian], LKI, Moscow (2008).

    Google Scholar 

  4. V. A. Bondarenko and S. V. Yurov, “About a Polyhedron of cubic graphs,” Fund. Inform., 28–35 (1996).

  5. S.-J. Chung, Structural Complexity of Adjacency on 0-1 Convex Polytopes, PhD Thesis, Univ. of Michigan (1980).

  6. M. M. Deza and M. Laurent, Geometry of Cuts and Metrics, Springer, Berlin (1997).

    Book  MATH  Google Scholar 

  7. S. Fiorini, “A combinatorial study of partial order polytopes,” Eur. J. Combin., 24, No. 2, 149–159 (2003).

    Article  MATH  MathSciNet  Google Scholar 

  8. S. Fiorini, S. Massar, S. Pokutta, H. R. Tiwary, and R. de Wolf, Linear vs. Semidefinite Extended Formulations: Exponential Separation and Strong Lower Bounds, arXiv:1111.0837v3 (2011).

  9. M. R. Garey and D. S. Johnson, Computers and Intractability: A Guide to the Theory of NP-Completeness, Freeman (1979).

  10. D. Geist and E. Y. Rodin, “Adjacency of the 0-1 knapsack problem,” Comput. Oper. Res., 19, No. 8, 797–800 (1992).

    Article  MATH  MathSciNet  Google Scholar 

  11. D. Hausmann, Adjacency on Polytopes in Combinatorial Optimization, Math. Systems Econ., Vol. 49, Hain, Konigstein (1980).

  12. M. Junger, G. Reinelt, and G. Rinaldi, “The traveling salesman problem,” in: Handbooks in Operations Research and Management Science, Vol. 7: Network Models, Elsevier, Amsterdam (1995), pp. 225–330.

  13. M. M. Kovalev, Discrete Optimization (Integer Programming) [in Russian], Bel. Gos. Univ., Minsk (1977).

    Google Scholar 

  14. A. N. Letchford, “The general routing polyhedron: a unifying framework,” Eur. J. Oper. Res., 112, No. 1, 122–133 (1999).

    Article  MATH  Google Scholar 

  15. A. N. Maksimenko, “Polyhedron combinatorial properties associated with the shortest path problem,” Comput. Math. Math. Phys., 44, No. 9, 1611–1614 (2004).

    MathSciNet  Google Scholar 

  16. A. N. Maksimenko, “On combinatorial properties of the double covering polytope,” in: Abstracts of the XIV Russ. Conf. “Mat. Progr. Pril.”, UrO RAN, Ekaterinburg (2011), pp. 197–198.

  17. A. N. Maksimenko, “On affine reducibility of combinatorial polytopes,” Dokl. Math., 85, No. 2, 283–285 (2012).

    Article  MATH  MathSciNet  Google Scholar 

  18. T. Matsui, “NP-completeness of non-adjacency relations on some 0-1-polytopes,” in: Operations Research with Applications in Engineering, Technology and Management. Int. Symp. 1st, Lect. Notes Operat. Res., Vol. 1, Beijing World Publ. (1995), pp. 249–258.

  19. T. Matsui and S. Tamura, “Adjacency on combinatorial polyhedra,” Discrete Appl. Math., 56, 311–321 (1995).

    Article  MATH  MathSciNet  Google Scholar 

  20. M. W. Padberg, “Equivalent knapsack-type formulations of bounded integer linear programs: An alternative approach,” Nav. Res. Logist. Quart., 19, No. 4, 699–708 (1972).

    Article  MATH  MathSciNet  Google Scholar 

  21. C. H. Papadimitriou, “The adjacency relation on the traveling salesman polytope is NP-complete,” Math. Programming, 14, No. 1, 312–324 (1978).

    Article  MATH  MathSciNet  Google Scholar 

  22. J.-J. Salazar-Gonzalez, “The Steiner cycle polytope,” Eur. J. Oper. Res., 147, No. 3, 671–679 (2003).

    Article  MATH  MathSciNet  Google Scholar 

  23. B. Toktas, J. W. Yen, and Z. B. Zabinsky, “Addressing capacity uncertainty in resource-constrained assignment problems,” Comput. Operat. Res., 33, No. 3, 724–745 (2006).

    Article  MATH  Google Scholar 

  24. M. Yannakakis, “Expressing combinatorial optimization problems by linear programs,” J. Comput. Syst. Sci., 43, No. 3, 441–466 (1991).

    Article  MATH  MathSciNet  Google Scholar 

  25. V. A. Yemelichev, M. M. Kovalev, and M. K. Kravtsov, Polytopes, Graphs and Optimization, Cambridge Univ. Press, New York (1984).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Yaroslavl State University, Sovetskaya 14, Yaroslavl, 150000, Russia

    A. Maksimenko

Authors
  1. A. Maksimenko
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. Maksimenko.

Additional information

Translated from Fundamentalnaya i Prikladnaya Matematika, Vol. 18, No. 2, pp. 105–118, 2013.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Maksimenko, A. The Common Face of some 0/1-Polytopes with NP-Complete Nonadjacency Relation. J Math Sci 203, 823–832 (2014). https://doi.org/10.1007/s10958-014-2172-9

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10958-014-2172-9

Keywords

Search

Navigation