Axisymmetric time-harmonic response of a surface-stiffened transversely isotropic half-space

Abstract

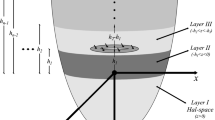
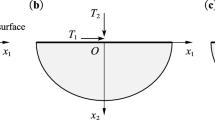
This study deals with the elastodynamic response of a surface-stiffened transversely isotropic half-space subjected to a buried time-harmonic normal load. The half-space is reinforced by a Kirchhoff thin plate on its surface. By virtue of a displacement potential function and appropriate time-harmonic Green’s functions of transversely isotropic half-spaces, a robust solution corresponding to two plate-medium bonding assumptions, namely (a) frictionless interface, and (b) perfectly bonded interface is obtained for the first time. All elastic fields of the problem are expressed explicitly in the form of semi-infinite line integrals. Results of some limiting cases including isotropic materials, static loading, and surface loading are recovered from the obtained solutions and subsequently have been verified with those available in the literature. Effects of anisotropy, depth of loading, bonding assumption, and frequency of excitation on the results are precisely discussed. Based on the proposed numerical scheme, some plots of practical importance are depicted.

Appendices

Appendix 1: Defined coefficients

$$\begin{aligned} a&=\frac{1}{2}\left( s_1^2+s_2^2\right) ,\\ b&=-\frac{1}{2}\rho \,\omega ^2\Big (\frac{1}{c_{33}} + \frac{1}{c_{44}}\Big ),\\ c&=\left( s_1^2-s_2^2\right) ^2,\\ d&=-2\rho \, \omega ^2\Big [\Big (\frac{1}{c_{33}}+\frac{1}{c_{44}} \Big )\left( s_1^2+s_2^2\right) -2\,\frac{c_{11}}{c_{33}} \left( \frac{1}{c_{11}}+\frac{1}{c_{44}}\right) \Big ],\\ e&=\rho ^2\omega ^4\Big (\frac{1}{c_{33}}-\frac{1}{c_{44}}\Big )^2, \\ \eta _i&=c_{13}\lambda _i^2+c_{11}(\xi ^2-k_P^2),\\ \vartheta _i&=(c_{13}+c_{44})\lambda _i^2-\eta _i, \\ \nu _i&=\Big [\eta _i-(c_{13}+c_{44})\big (\frac{c_{13}}{c_{33}}\, \xi ^2+\lambda _i^2\big )\Big ]\lambda _i,\\ I^{-}&=\eta _2\,\nu _1-\eta _1\,\nu _2,\quad i=1,2. \end{aligned}$$

Appendix 2: Internal forces and couples of plate

$$\begin{aligned} M_r^f(r) &=\int _0^{\infty } \frac{F_z D c_{11}(c_{13}+c_{44})\left( \xi ^2-k_P^2\right) }{2\pi c_{33}c_{44}I^-}\left( \frac{\nu _1}{\lambda _1}e^{-\lambda _1 s}-\frac{\nu _2}{\lambda _2}e^{-\lambda _2s}\right) \\&\quad\times\,\frac{\xi ^2}{1+{\varPsi }_f (\xi )}\Big [\xi J_0(r\xi ) + \Big (\frac{\nu _p-1}{r}\Big )J_1(r\xi )\Big ]d\xi , \\ M_\theta ^f(r)&=\int _0^{\infty } \frac{F_z D c_{11}(c_{13}+c_{44})\left( \xi ^2-k_P^2\right) }{2\pi c_{33}c_{44}I^-}\left( \frac{\nu _1}{\lambda _1}e^{-\lambda _1 s}-\frac{\nu _2}{\lambda _2}e^{-\lambda _2s}\right) \\&\quad\times\,\frac{\xi ^2}{1+{\varPsi }_f (\xi )}\Big [\nu _p\,\xi J_0(r\xi )-\Big (\frac{\nu _p-1}{r}\Big )J_1(r\xi )\Big ]d\xi , \\ Q_r^f(r)&=\int _0^{\infty } \frac{F_z D c_{11}(c_{13}+c_{44})\left( \xi ^2-k_P^2\right) }{2\pi c_{33}c_{44}I^-}\left( \frac{\nu _1}{\lambda _1}e^{-\lambda _1 s}-\frac{\nu _2}{\lambda _2}e^{-\lambda _2s}\right) \\&\quad\times\,\frac{\xi ^4 J_1(r \xi )}{1+{\varPsi }_f (\xi )}d\xi , \\ M_r^b(r)&=\int _0^{\infty } \frac{F_z D}{2\pi c_{33}c_{44}(\lambda _1^2-\lambda _2^2)}\left( \frac{\vartheta _1}{\lambda _1} e^{-\lambda _1 s}-\frac{\vartheta _2}{\lambda _2}e^{-\lambda _2s}\right) \\&\quad\times\,\frac{\xi ^2}{1+{\varPsi }_b (\xi )}\Big [\xi J_0(r\xi )+\Big (\frac{\nu _p-1}{r}\Big )J_1(r\xi )\Big ]d\xi , \\ M_\theta ^b(r)&=\int _0^{\infty } \frac{F_z D}{2\pi c_{33}c_{44}(\lambda _1^2-\lambda _2^2)}\left( \frac{\vartheta _1}{\lambda _1} e^{-\lambda _1 s}-\frac{\vartheta _2}{\lambda _2}e^{-\lambda _2s}\right) \\&\quad\times\,\frac{\xi ^2}{1+{\varPsi }_b (\xi )}\Big [\nu _p \,\xi J_0(r\xi )-\Big (\frac{\nu _p-1}{r}\Big )J_1(r\xi )\Big ]d\xi , \\ Q_r^b(r)&=\int _0^{\infty } \frac{F_z D}{2\pi c_{33} c_{44}(\lambda _1^2-\lambda _2^2)}\left( \frac{\vartheta _1}{\lambda _1} e^{-\lambda _1 s}-\frac{\vartheta _2}{\lambda _2} e^{-\lambda _2s}\right) \\&\quad\times\,\frac{\xi ^4 J_1(r \xi )}{1+{\varPsi }_b(\xi )}d\xi . \end{aligned}$$