Skip to main content
SpringerLink
Log in

Long-Time Asymptotics for the Focusing Hirota Equation with Non-Zero Boundary Conditions at Infinity Via the Deift-Zhou Approach

  • Published:
Mathematical Physics, Analysis and Geometry Aims and scope Submit manuscript

Abstract

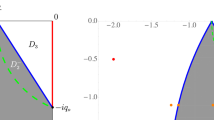
We are concerned with the long-time asymptotic behavior of the solution for the focusing Hirota equation (also called third-order nonlinear Schrödinger equation) with symmetric, non-zero boundary conditions (NZBCs) at infinity. Firstly, based on the Lax pair with NZBCs, the direct and inverse scattering problems are used to establish the oscillatory Riemann-Hilbert (RH) problem with distinct jump curves. Secondly, the Deift-Zhou nonlinear steepest-descent method is employed to analyze the oscillatory RH problem such that the long-time asymptotic solutions are proposed in two distinct domains of space-time plane (i.e., the plane-wave and modulated elliptic-wave domains), respectively. Finally, the modulation instability of the considered Hirota equation is also investigated.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Hirota, R.: Exact envelope-soliton solutions of a nonlinear wave equation. J. Math. Phys. 14, 805–809 (1973)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  2. Kivshar, Y.S., Agrawal, G.P.: Optical Solitons: From Fibers to Photonic Crystals. Academic Press, New York (2003)

    Google Scholar 

  3. Kodama, Y., Hasegawa, A.: Nonlinear pulse propagation in a monomode dielectric guide. IEEE J. Quantum Electron. 23, 510 (1987)

    Article  ADS  Google Scholar 

  4. Yan, Z., Dai, C.: Optical rogue waves in the generalized inhomogeneous higher-order nonlinear Schrödinger equation with modulating coefficients. J. Opt. 15, 064012 (2013)

    Article  ADS  Google Scholar 

  5. Ablowitz, M.J., Kaup, D.J., Newell, A.C., Segur, H.: Nonlinear-evolution equations of physical significance. Phys. Rev. Lett. 31, 125 (1973)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  6. Chen, H.-H.: General derivation of Bäcklund transformations from inverse scattering problems. Phys. Rev. Lett. 33, 925 (1974)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  7. Gedalin, M., Scott, T.C., Band, Y.B.: Optical solitary waves in the higher order nonlinear Schrödinger equation. Phys. Rev. Lett. 78, 448 (1997)

    Article  ADS  Google Scholar 

  8. Sasa, N., Satsuma, J.: New-type of soliton solutions for a higher-order nonlinear Schrödinger equation. J. Phys. Soc. Jpn. 60, 409–417 (1991)

    Article  ADS  MATH  Google Scholar 

  9. Zhang, G., Chen, S., Yan, Z.: Focusing and defocusing Hirota equations with non-zero boundary conditions: Inverse scattering transforms and soliton solutions. Commun. Nonlinear Sci. Numer. Simulat. 80, 104927 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  10. Ankiewicz, A., Soto-Crespo, J.M., Akhmediev, N.: Rogue waves and rational solutions of the Hirota equation. Phys. Rev. E 81, 046602 (2010)

    Article  ADS  MathSciNet  Google Scholar 

  11. Yang, Y., Yan, Z., Malomed, B.A.: Rogue waves, rational solitons, and modulational instability in an integrable fifth-order nonlinear Schrödinger equation. Chaos 25, 103112 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  12. Chen, S., Yan, Z.: The Hirota equation: Darboux transform of the Riemann-Hilbert problem and higher-order rogue waves. Appl. Math. Lett. 95, 65–71 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  13. Ablowitz, A.J., Clarkson, P.A.: Solitons, Nonlinear Evolution Equations and Inverse Scattering. Cambridge Univeristy Press, Cambridge (1991)

    Book  MATH  Google Scholar 

  14. Bilman, D., Miller, P.D.: A robust inverse scattering transform for the focusing nonlinear Schrödinger equation. Commun. Pure Appl. Math. 72, 1722–1805 (2019)

    Article  MATH  Google Scholar 

  15. Sulem, C., Sulem, P.L.: The Nonlinear SchrÖDinger Equation: Self-Focusing and Wave Collapse. Springer, New York (1999)

    MATH  Google Scholar 

  16. Malomed, B.A., Mihalache, D., Wise, F., Torner, L.: Spatiotemporal optical solitons. J. Opt. B Quantum Semiclass. Opt. 7, R53 (2005)

    Article  ADS  Google Scholar 

  17. Osborne, A.R.: Nonlinear Ocean Waves. Academic, New York (2009)

    Google Scholar 

  18. Pitaevskii, L., Stringari, S.: Bose-Einstein Condensation and Superfluidity. Oxford University Press, Oxford (2016)

    Book  MATH  Google Scholar 

  19. Yan, Z.: Financial rogue waves. Commun. Theor. Phys. 54, 947–949 (2010)

    Article  ADS  MATH  Google Scholar 

  20. Buckingham, R., Venakides, S.: Long-time asymptotics of the nonlinear Schrödinger equation shock problem. Comm. Pure Appl. Math. 60, 1349–1414 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  21. Biondini, G., Mantzavinos, D.: Long-time asymptotics for the focusing nonlinear Schrödinger equation with nonzero boundary conditions at infinity and asymptotic stage of modulational instability. Comm. Pure Appl. Math. 70, 2300–2365 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  22. Gardner, C.S., Greene, J.M., Kruskal, M.D., Miura, R.M.: Method for solving the Korteweg-de Vries equation. Phys. Rev. Lett. 19, 1095–1097 (1967)

    Article  ADS  MATH  Google Scholar 

  23. Zakharov, V.E., Shabat, A.B.: Exact theory of two-dimensional self-focusing and one-dimensional self-modulation of waves in nonlinear media. Sov. Phys. JETP 34, 62–69 (1972)

    ADS  MathSciNet  Google Scholar 

  24. Ablowitz, M.J., Kaup, D.J., Newell, A.C., Segur, H.: The inverse scattering transform-Fourier analysis for nonlinear problems. Stud. Appl. Math. 53, 249–315 (1974)

    Article  MathSciNet  MATH  Google Scholar 

  25. Novikov, S.P., Manakov, S.V., Pitaevskii, L.P., Zakharov, V.E.: The Theory of Solitons: The Inverse Scattering Method. Plenum, New York (1984)

    MATH  Google Scholar 

  26. Faddeev, L.D., Takhtajan, L.A.: Hamiltonian Methods in the Theory of Solitons. Springer, Berlin (1987)

    Book  MATH  Google Scholar 

  27. Shabat, A.: One-Dimensional Perturbations of a Differential Operator, and the Inverse Scattering Problem. Problems in Mechanics and Mathematical Physics, pp 279–296. Nauka, Moscow (1976)

    Google Scholar 

  28. Zakharov, V.E., Shabat, A.B.: Interaction between solitons in a stable medium. Sov. Phys. JETP 37, 823–828 (1973)

    ADS  Google Scholar 

  29. Au-Yeung, T., Fung, P., Au, C.: Modified KdV solitons with non-zero vacuum parameter obtainable from the ZS-AKNS inverse method. J. Phys. A: Math. Gen. 17, 1425 (1984)

    Article  ADS  MATH  Google Scholar 

  30. Alejo, M.A.: Focusing mKdV breather solutions with nonvanishing boundary condition by the inverse scattering method. J. Nonlinear Math. Phys. 19, 119–135 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  31. Prinari, B., Ablowitz, M.J., Biondini, G.: Inverse scattering transform for the vector nonlinear Schrödinger equation with nonvanishing boundary conditions. J. Math. Phys. 47, 063508 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  32. Demontis, F., Prinari, B., van der Mee, C., Vitale, F.: The inverse scattering transform for the defocusing nonlinear Schrödinger equations with nonzero boundary conditions. Stud. Appl. Math. 131, 1–40 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  33. Biondini, G., Kovacic, G.: Inverse scattering transform for the focusing nonlinear Schrödinger equation with nonzero boundary conditions. J. Math. Phys. 55, 031506 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  34. Prinari, B., Demontis, F., Li, S., Horikis, T.P.: Inverse scattering transform and soliton solutions for square matrix nonlinear Schrödinger equations with non-zero boundary conditions. Phys. D 368, 22–49 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  35. Ablowitz, M.J., Feng, B.-F., Luo, X.-D., Musslimani, Z.H.: Reverse space-time nonlocal sine-Gordon/sinh-Gordon equations with nonzero boundary conditions. Stud. Appl. Math. 141, 267–307 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  36. Zhang, G., Yan, Z.: Inverse scattering transforms and soliton solutions of focusing and defocusing nonlocal mKdV equations with non-zero boundary conditions. Phys. D. https://doi.org/10.1016/j.physd.2019.132170 (2019)

  37. Fokas, A.S.: A unified transform method for solving linear and certain nonlinear PDEs. Proc. R. Soc. Lond. Ser. A 453, 1411 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  38. Fokas, A.S., Himonas, A.A., Mantzavinos, D.: The nonlinear Schrödinger equation on the half-line. Trans. Amer. Math. Soc. 369, 681–709 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  39. Pelloni, B.: Advances in the study of boundary value problems for nonlinear integrable PDEs. Nonlinearity 28, R1 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  40. Boutet de Monvel, A., Fokas, A.S., Shepelsky, D.: Integrable nonlinear evolution equations on a finite interval. Comm. Math. Phys. 263, 133 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  41. Lenells, J.: Initial-boundary value problems for integrable evolution equations with 3 × 3 Lax pairs. Phys. D 241, 857 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  42. Xu, J., Fan, E.: The unified transform method for the Sasa-Satsuma equation on the half-line. Proc. R. Soc. Ser. A 469, 20130068 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  43. Geng, X., Liu, H., Zhu, J.: Initial-boundary value problems for the coupled nonlinear Schrödinger equation on the half-line. Stud. Appl. Math. 135, 310 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  44. Yan, Z.: An initial-boundary value problem for the integrable spin-1 Gross-Pitaevskii equations with a 4 × 4 Lax pair on the half-line. Chaos 27, 053117 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  45. Yan, Z.: Initial-boundary value problem for the spin-1 Gross-Pitaevskii system with a 4 × 4 Lax pair on a finite interval. J. Math. Phys. 60, 083511 (2019)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  46. Its, A.R.: Asymptotic behavior of the solutions to the nonlinear Schrödinger equation, and isomonodromic deformations of systems of linear differential equations, Dokl. Akad. Nauk SSSR 261 (1981) 14-18 (in Russian). Soviet. Math. Dokl. 24, 452–456 (1982). (in English)

    Google Scholar 

  47. Manakov, S.V.: Nonlinear Fraunhofer diffraction, Zh. Eksp. Teor. Fiz. 65 (1973) 1392-1398 (in Russian). Sov. Phys. JETP 38, 693–696 (1974). (in English)

    ADS  Google Scholar 

  48. Deift, P.A., Zhou, X.: A steepest descent method for oscillatory Riemann-Hilbert problems. Asymptotics for the MKdV equation. Ann. Math. 137, 295–368 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  49. Deift, P.A., Venakides, S., Zhou, X.: The collisionless shock region for the long-time behavior of solutions of the KdV equation. Comm. Pure Appl. Math. 47, 199–206 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  50. Kamvissis, S.: On the long time behavior of the doubly infinite Toda lattice under initial data decaying at infinity. Comm. Math. Phys. 153, 479–519 (1993)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  51. Deift, P.A., Venakides, S., Zhou, X.: An extension of the steepest descent method for Riemann-Hilbert problems: the small dispersion limit of the Korteweg-de Vries (KdV) equation. Proc. Natl. Acad. Sci. USA 95, 450–454 (1998)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  52. Kitaev, A.V., Vartanian, A.H.: Asymptotics of solutions to the modified nonlinear Schrödinger equation: solution on a nonvanishing continuous background. SIAM J. Math. Anal. 30, 787–832 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  53. Andreiev, K., Egorova, I., Lange, T.L., Teschl, G.: Rarefaction waves of the Korteweg-de Vries equation via nonlinear steepest descent. J. Diff. Equ. 261, 5371–5410 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  54. Boutet de Monvel, A., Kostenko, A., Shepelsky, D., Teschl, G.: Long-time asymptotics for the Camassa-Holm equation. SIAM J. Math. Anal. 41, 1559–1588 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  55. Xu, J., Fan, E.: Long-time asymptotics for the Fokas-Lenells equation with decaying initial value problem: Without solitons. J. Diff. Equ. 259, 1098–1148 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  56. Huang, L., Xu, J., Fan, E.: Long-time asymptotic for the Hirota equation via nonlinear steepest descent method. Nonlinear Anal. Real World Appl. 26, 229–262 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  57. Buckingham, R., Venakides, S.: Long-time asymptotics of the nonlinear Schrödinger equation Shock problem. Comm. Pure Appl. Math. 60, 1349–1414 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  58. de Monvel, A.B., Lenells, J., Shepelsky, D.: Long-time asymptotics for the Degasperis-Procesi equation on the half-line. Ann. Inst. Fourier, in press, arXiv:1508.04097v2 (2018)

  59. Lenells, J.: The nonlinear steepest descent method: asymptotics for initial-boundary value problems. SIAM J. Math. Anal. 48, 2076–2118 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  60. Wang, D., Guo, B., Wang, X.: Long-time asymptotics of the focusing Kundu-Eckhaous equation with nonzero boundary conditions. J. Diff. Equ. 266, 5209–5253 (2019)

    Article  ADS  MATH  Google Scholar 

  61. Guo, B., Liu, N., Wang, Y.: Long-time asymptotics for the Hirota equation on the half-line. Nonlinear Anal. 174, 118–140 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  62. Boutet de Monvel, A., Kotlyarov, V.P., Shepelsky, D.: Focusing NLS equation: long-time dynamics of step-like initial data. Int. Math. Res. Not. 7, 1613–1653 (2011)

    MathSciNet  MATH  Google Scholar 

  63. Zakharov, V.E., Ostrovsky, L.A.: Modulation instability: The beginning. Phys. D 238, 540–548 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  64. Benjamin, T.B., Feir, J.E.: The disintegration of wave trains on deep water Part 1. Theory. J. Fluid Mech. 27, 417–430 (1967)

    Article  ADS  MATH  Google Scholar 

  65. Biondini, G., Li, S., Mantzavinos, D.: Long-time asympototics for the focusing nonlinar Schrödinger equation with nonzero boundary conditions in the presentence of a discrete spectrum.arXiv:1907.09432v1 (2019)

  66. Gakhov, F.D.: Boundary Value Problems. Dover, New York (1966)

    Book  MATH  Google Scholar 

Download references

Acknowledgements

The authors thank the referee for the valuable suggestions and comments. This work was partially supported by the NSFC under Grants Nos. 11731014 and 11925108.

Author information

Authors and Affiliations

  1. Institute of Applied Physics and Computational Mathematics, 100088, Beijing, China

    Shuyan Chen & Boling Guo

  2. KLMM, Academy of Mathematics and Systems Science, Chinese Academy of Sciences, 100190, Beijing, China

    Zhenya Yan

  3. School of Mathematical Sciences, University of Chinese Academy of Sciences, 100049, Beijing, China

    Zhenya Yan

Authors
  1. Shuyan Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhenya Yan
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Boling Guo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhenya Yan.

Additional information

Communicated by:Mark Adler

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, S., Yan, Z. & Guo, B. Long-Time Asymptotics for the Focusing Hirota Equation with Non-Zero Boundary Conditions at Infinity Via the Deift-Zhou Approach. Math Phys Anal Geom 24, 17 (2021). https://doi.org/10.1007/s11040-021-09388-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11040-021-09388-0

Keywords

Mathematics Subject Classification (2010)

Search

Navigation