Switching costs, experience goods and dynamic price competition

Abstract

I analyze a dynamic duopoly with an infinite horizon where consumers are uncertain about their potential satisfaction from the products and face switching costs. I derive sufficient conditions for the existence of a Markov Perfect Equilibrium(MPE) where switching takes place each period. I show that when switching costs are sufficiently low, the prices in the steady state are lower than what they would have been when they are absent. This result is in contrast to those found in the literature. In the presence of low switching costs competition can be fiercer.

Appendices

Appendix

Proof of Lemma 1

Lemma 1

The policy function parameters which satisfies the FOCs are given by

$$\label{ll} l^*=\frac{1}{2} a^*+{\frac { \left( 2 z(a^*) -\beta \Delta a^* \right) \Delta}{{\Delta}^{2}+2 \beta \Delta s+2 z(a^*) }} $$

(24)

$$\label{kl} k^*=-a^* $$

(25)

and the parameters of the value functions consistent with (l^*,k^*) are given by

$$\label{n2l} n_2^*={a^*}^{2} \left( \frac{1}{\Delta}+\frac{1}{2}{\frac {\Delta}{z(a^*) } } \right) , $$

(26)

$$\label{n1l} n_1^*=-n_{{2}}^*+a^*+{\frac {2 s \left( 2 z(a^*) -\beta a\Delta \right) }{{\Delta}^{2}+2 \beta \Delta s +2 z(a^*) }}, $$

(27)

$$\label{n0l} n_0^*=\frac{1}{2}\,{\frac { \left( n_{{1}}^*+n_{{2}}^*-a^* \right) \Delta}{ \left( 1- \beta \right)s }}+\frac{1}{4}\,n_{{2}}^*+\frac{1}{2}\,n_{{1}}^* $$

(28)

where z(a) = Δ − 2 asβ and a^* solves g(a) = 0 with g(a) is given by

$$\label{aeqa} g(a)= a+{\frac { 4 z(a) ^{3} s}{ \left( { \Delta}^{2}+2 z(a) \right) \left( 3 z(a)^{2}-\beta {\Delta}^{2}{a}^{2} \right) }} $$

(29)

□

Proof

Firms solve the dynamic optimization problems stated in (11) and (12) taking the policy function of their opponent given. Therefore the candidate equilibrium prices of both firms at time period t, given the state is x ^t, can be found by simply differentiating the right hand side of (11) and (12) with the next state as defined in these problems, and equating the resulting derivatives to zero. This yields

$$p_1^*(x^t) = {\frac {2s\,z(a)^{2} \left( 2\,x^t-1 \right) }{6\,z(a)^{2}+3\,{\Delta}^{2} z(a) -2\,\beta\,{\Delta}^{3}n_{{2}}}}+{\frac {\Delta\, \left( 2\,z(a) -\Delta\,\beta\, \left( n_{{1}}+n_{{2}} \right) \right) }{{\Delta}^{2}+2\,z(a) }}, \label{cp1} $$

(30)

$$p_2^*(x^t) = -{\frac {2s\,z(a)^{2} \left( 2\,x^t-1 \right) }{6\, z(a)^{2}+3\,{\Delta}^{2} z(a) -2\,\beta\,{\Delta}^{3}n_{{2}}}}+{\frac {\Delta\, \left( 2\,z(a) -\Delta\,\beta\, \left( n_{{1}}+n_{{2}} \right) \right) }{{\Delta}^{2}+2\,z(a) }}. $$

(31)

The parameters of the policy functions, (l,k), are simply the constant term and the coefficient of the x ^t in these expressions. To arrive at the expressions given the lemma, the terms involving n ₁ and n ₂ have to be eliminated however.

Due to the symmetry of the value functions, it is sufficient to consider the problem faced by one of the firms. Substituting the candidate equilibrium prices in (11), I obtain

$$\label{bellman} V(x^t)=\pi(p_1^*(x^t),p_2^*(x^t),x^t)+\beta V(x^{t+1}). $$

(32)

There are quadratic functions of x ^t on both sides of (32). Equating the coefficients of each term provides three equations which I will use to solve for (n ₀,n ₁,n ₂). Furthermore, the price differential has to satisfy $p_1^*(x^t)-p_2^*(x^t)=a(1-2x^t)$ for the expectations to be fulfilled. Calculating this price differential results in

$$ p_1^*(x^t)-p_2^*(x^t)=-{\frac {4s\,z(a)^{2} \left( 1-2\,x^t \right) }{6\, z(a)^{2}+3\,{\Delta}^{2} z(a) -2\,\beta\,{\Delta}^{3}n_{{2}}}}. $$

This implies that a has to satisfy

$$\label{exps} h=a+{\frac {4s\,z(a)^{2} }{6\, z(a)^{2}+3\,{\Delta}^{2} z(a) -2\,\beta\,{\Delta}^{3}n_{{2}}}}=0. $$

(33)

Let us denote with f _i , the equation that collects the difference between the coefficients of the ith power of x ^t for i = 0,1,2 in (32). That is, f _i is the difference between the terms involving (x ^t)ⁱ, on the left and right hand side of (32). The equation involving the squared terms leads to

$$\label{x2} f_2=n_{{2}}+8\,{\frac {{s}^{2} z \left( a \right)^{3} \left( {\Delta}^{2}+2\,z \left( a \right) \right) }{ \left( 6\, z \left( a \right)^{2}+3\,{\Delta}^{2}z \left( a \right) -2\,\beta\,{\Delta}^{3}n_{{2}} \right) ^{2}}}=0. $$

(34)

Equations (33) and (34) can be solved for a and n ₂ in terms of the parameters of the model resulting in $n_2^*$ as given in the lemma. Observe that $n_2^*$ is nonnegative whenever a ^* is nonpositive. Substituting $n_2^*$ in (33) results in the expression given in (29) for g(a) and the equilibrium value of a must be one of the roots of g(a).

By equating the coefficients of x ^t in (32), one obtains an equation for n ₁ in terms of the already determined $n_2^*$ and a ^*. This equation looks complicated at first but can be rearranged as

$$\begin{array}{lll} f_1&=&-f_2+h+{\frac { \left( {\Delta}^{2}+2\,\beta\,s\,\Delta+2\,z \left( a^* \right) \right) \left( n_{{1}}+n_{{2}}^*-a^* \right) }{{\Delta}^{2}+2\, z \left( a^* \right) }}\\ &&-\,{\frac {2 s\, \left( 2\,z \left( a^* \right) - \beta\,a^*\Delta \right) }{{\Delta}^{2}+2\,z \left( a^* \right) }}=0. \end{array}$$

Given f ₂ and h are zero, solving this equation for n ₁ yields the expression given in the lemma for $n_1^*$.

Similarly, equating the constants on both sides of (32) leads to an equation which can be simplified to

$$\begin{array}{lll} f_0&=&\frac{f_2}{4}-\frac{h}{2}+\frac{\Delta-s}{2s}(f_1+f_2-h)+ \left( 1-\beta \right) n_{{0}}-{\frac { \left( n_{{1}}^*+n_{{2}}^*-a^* \right) \Delta}{2s}} \\ &&+\frac{1}{4}\left( n_{{2}}^*+2\,n_{{1}}^* \right) \left( 1 -\beta \right)=0. \end{array}$$

Given that f ₁,f ₂ and h are all identically zero, solving the above equation for n ₀ yields the expression given in the lemma for $n_0^*$.

Comparing the coefficient of x ^t in (30) with (33) implies that k ^* = − a ^*. Also substituting $n_1^*$ and $n_2^*$ in the constant term in (30) and simplifying yields the expression for l ^* given in the lemma. □

Proof of Lemma 2

Lemma 2

There is no root of g(a), given in (18), in the interval $(0, {\overline{a}}_1]$.□

Proof

The equilibrium value of a will be one of the four roots of g(a), therefore it will be useful characterize where these root must lie. Observe that there are three values of a which makes g(a) undefined. These are

$$\begin{array}{lll} \rho_1&=&-{\frac {\sqrt {3}\Delta}{\sqrt {\beta} \left( \Delta-2\,\sqrt {3} \sqrt {\beta}s \right) }},\\ \rho_2&=&{\frac {\sqrt {3}\Delta}{\sqrt {\beta} \left( \Delta+2\,\sqrt {3} \sqrt {\beta}s \right) }},\\ \rho_3&=&{\frac {\Delta\, \left( \Delta+2 \right) }{4\beta\,s}}. \end{array}$$

It is easy to verify that ρ ₁ < 0 < ρ ₂ < ρ ₃ whenever Δ² ≥ 12βs ², and ρ ₂ <  min (ρ ₁,ρ ₃) whenever Δ² < 12βs ². Furthermore, Δ² + z(a) ≥ 0, whenever a ≤ ρ ₃. On the other hand, 3z(a)² − βΔ ² a ² ≥ 0 whenever ρ ₁ ≤ a ≤ ρ ₂ if Δ² ≥ 12βs ², and whenever a ≤ ρ ₂ and ρ ₁ ≤ a if Δ² < 12βs ².

Recall also that

$$\begin{array}{lll} {\underline{a}}_1&=&-\frac{\Delta}{\Delta-2\beta s},\\ {\overline{a}}_1&=&\ \ \frac{\Delta}{\Delta+2\beta s},\\ {\underline{a}}_2&=&-\frac{\Delta}{2}+s,\\ {\overline{a}}_2&=&\ \ \frac{\Delta}{2}-s. \end{array}$$

It is also easy to verify that ${\underline{a}}_1>\rho_1$ and ${\overline{a}}_1<\rho_2$ whenever Δ² ≥ 12βs ², while ${\underline{a}}_1 < {\overline{a}}_1 < \rho_2 < \min(\rho_1,\rho_3)$ whenever Δ² < 12βs ².

Let g ₁(a) = g(a) − a. Notice that g ₁(a) ≥ 0 for all a such that ρ ₁ < a < ρ ₂ when Δ² ≥ 12βs ², and for all a < ρ ₂ when Δ² < 12βs ². Therefore for 0 < a < ρ ₂, g(a) = g ₁(a) + a is strictly positive, and has no roots satisfying g(a) = 0. □

Proof of Lemma 3

Let

$$\begin{array}{lll} \Delta_1&=& -1+s\, \left( 1-\beta \right) +\sqrt { \left( 1+ \left( 1+\beta \right) s \right) ^{2}+4\,s},\\ \Delta_2&=&-1+2\,\beta\,s\, \frac{4-\beta}{3-\beta} + \sqrt { \left( -1+2\,\beta\,s\, \frac{4-\beta}{3-\beta} \right) ^{2}+8\,\beta\,{s}^{2}}, \\ \Delta_3&=&- \left( 1+\beta \right) s+\sqrt {{s}^{2} \left( 1-\beta \right) ^{2}- 4\,s+8\,\beta\,s+4} ,\\ \Delta_4&=&3- \left( 1+\beta \right) s+\sqrt { \left( 3- \left( 1+\beta \right) s \right) ^{2}+4\, \left( 2-s \right) \left(1+ \beta\,s \right) } \end{array}$$

and ${\cal D}=\{(\beta,\Delta,s) \mid 0\le \beta \le 1, s\ge 0, \max(\Delta_1,\Delta_2,\Delta_3)\le \Delta \le \Delta_4\}$.

Lemma 3

A sufficient condition for g(a) to have a negative root, which satisfies (20), (21), leads to non-negative $(n_2^*,n_1^*,n_0^*)$ and neither firm deviates from the candidate equilibrium policies, is ${\cal D} \ne \emptyset$ and $(\beta,\Delta,s)\in {\cal D}$.

Proof

The proof will proceed in several steps.

• 1. There exists a such that g(a) = 0 and $a\in [max({\underline{a}}_1,{\underline{a}}_2),0]$ and leads to $n_1^*\ge 0$.

First I will find a particular value a ₀, such that $n_1^*\ge 0$ whenever a ≥ a ₀. Then, I will show that g(a ₀) ≤ 0 and g(0) ≥ 0, thus there must be a root of g(a) in [a ₀,0]. First, notice that $2 a<-n_2^*+a$, since a ²/Δ < − a/2 whenever ${\underline{a}}_2=-\Delta/2+s<a$ and a ²Δ/2z(a) < − a/2 whenever Δa/z(a) > − 1, thus $a<-n_2^*$. Therefore,

$$\tilde n_1 =2 a+{\frac {2 s \left( 2 z(a)-\beta a\Delta \right) }{{\Delta}^{2}+2 \beta \Delta s +2 z(a) }}<n_1^*,$$

for all $\max({\underline{a}}_1,{\underline{a}}_2)\le a \le 0$. Furthermore, it is easy to verify that $2 s \left( 2 z(a)-\beta a\Delta \right)/(\Delta^{2}+2 \beta \Delta s +2 z(a) )$ is nonnegative and decreasing in a for $\max({\underline{a}}_1,{\underline{a}}_2)\le a \le 0$. Hence,

$$\hat n_1 =2 a+\frac {4 s} {{\Delta} +2 \beta s + 2 }\le \tilde n_1 \le n_1^*,$$

Let

$$a_0=-\frac {2 s} {{\Delta} +2 \beta s + 2 }.$$

Observe that $\hat n_1\ge 0$, and in turn $n_1^*\ge 0$, whenever a ₀ ≤ a ≤ 0. It is easy to verify that $a_0\ge {\underline{a}}_1$ as long as Δ> 2s, however, we also need that ${\underline{a}}_2<a$. As long as ${\underline{a}}_2\le a_0$, this condition will be satisfied. Observe that, the denominator of

$$a_0-{\underline{a}}_2=-\frac {2 s} {{\Delta} +2 \beta s + 2 }+\frac{\Delta}{2}-s,$$

is positive and the numerator is quadratic-convex function of Δ. Thus, it is positive outside of the roots of the numerator in Δ. The positive root is given by Δ₁ above.

I will now proceed to show that there is an a ₀ ≤ a ≤ 0 which solves g(a) = 0. Let g ₁(a) = g(a) − a. It is easy to confirm that

$$g_2(a)={\frac { 4 z(a) ^{3} s}{\left( { \Delta}^{2}+2 \Delta \right) \left( 3 z(a)^{2} -\beta {\Delta}^{2}{a}^{2} \right) }}\ge g_1(a).$$

Note that g ₂(a) is decreasing and convex on $[{\underline{a}}_1,0]$ since

$$\frac{\partial }{\partial a}g_2(a)= -{\frac {8 \beta\, s\, z \left( a \right) ^{2} \left( (3\, z \left( a \right) ^{2}-\beta { \Delta}^{2} {a}^{2})s-{\Delta}^{3}a \right) }{\Delta\, \left(2+ \Delta \right) \left( 3\, z \left( a \right)^{2}-\beta\,{\Delta}^{2 }{a}^{2} \right) ^{2}}}\le 0, $$

and

$$\frac{\partial^2 }{\partial a^2}g_2(a)= {\frac {24 \beta\, {\Delta}^{3} s\,z \left( a \right) \left( z\left( a \right)^{2} +\beta\,{\Delta}^{2}{a}^{2} \right) }{ \left( 2+ \Delta \right) \left( 3\, z \left( a \right)^{2} -\beta\,{\Delta}^{2}{a}^{2} \right) ^{3}}}\ge 0. $$

Therefore, the line going through $({\underline{a}}_1,g_2({\underline{a}}_1))$ and (0,g ₂(0)) is always above g ₂(a). That is,

$$\begin{array}{lll} g_3(a)&=& -\frac{g_2(0)-g_2({\underline{a}}_1)}{{\underline{a}}_1}a+g_2(0)\\ &=&-\frac{4}{3}\,{\frac { \left( \Delta+6\,s-2\,\beta\,s \right) \beta\,s\,}{ \Delta\, \left( 3-\beta \right) \left( \Delta+2 \right) }}\ a+\frac{4}{3}\,{ \frac {s}{\Delta+2}} \ge g_2(a), \end{array}$$

and, hence,

$$a+g_3(a)=a -\frac{g_2(0)-g_2({\underline{a}}_1)}{{\underline{a}}_1}a+g_2(0)\ge a+g_2(a)\ge g(a).$$

Notice that

$$g_3(0)=g(0)=\frac{4s}{3(\Delta+2)}\ge 0.$$

Furthermore, evaluating a ₀ + g ₃(a ₀) yields

$$a_0+g_3(a_0)=-{\frac {2 s\, \left[ \left( 3-\beta \right) \left( {\Delta}^{2}+ 2\,\Delta-4\,\beta\,s\,\Delta-8\,\beta\,{s}^{2} \right) -4\,\beta\,s\, \Delta \right] } {3\Delta\,\left( 3-\beta \right) \left( \Delta+2 \right) \left( \Delta+2\,\beta\,s+2 \right) }}, $$

and given that the denominator is positive, the sign of g ₃(a ₀) is the opposite of the sign of the term in the brackets. This term is a quadratic-convex function of Δ, hence it is positive outside of its roots. Computing the roots results in one negative root and a positive one given by Δ₂, defined above. Thus, for Δ ≥ Δ₂, 0 ≥ a ₀ + g ₃(a ₀) ≥ g(a ₀), therefore there must be a root of g(a) in [a ₀,0]. Moreover, $n_1^*$ evaluated at this root must be non-negative. Observe that whenever a ≤ 0, $n_2^*$ is non-negative, and whenever $n_1^*$ and $n_2^*$ are non-negative as well as a ^* non-positive, $n_0^*$ is non-negative. □

I will now proceed to derive the bounds necessary so that firms do not deviate from the candidate equilibrium policies. The underlying reason why they might want to deviate is the difference in price sensitivities of the demand from older customers and younger customers. For large Δ, old customers become less price sensitive, therefore a firm might find it profitable to charge a price high enough that no young consumers buy from it. While for Δ very small, firms might find it most profitable just to target some of the young consumers. Let us first derive the necessary conditions, so that the latter type deviation is not possible. I will develop the arguments, without loss of generality, by considering a deviation of firm 1 while firm 2 follows the equilibrium policy.

• 2. Firm 1 cannot deviate to just serve the young consumers.

Recall that the demand faced by firm 1 from the older customers is given by

$$d_1^o=\frac{1}{2}+{\frac {s\, \left( 2\,{x}^{t}-1 \right) }{\Delta}}+{\frac {p_{{2}} -p_{{1}}}{\Delta}},$$

and is increasing in x ^t. It is easy to confirm that $d_1^o\le 0$, whenever

$$p_1-p_2=\varrho\ge \frac{\Delta}{2}+s.$$

Similarly, the demand from the young consumers is given by

$$d_1^n=\frac{1}{2}+\frac{1}{2}\,{\frac {\Delta\, \left( p_{{2}}-p_{{1}} \right) }{z(a)}}$$

and is positive whenever $\varrho\le z(a)/\Delta$.

The type of deviation I am considering requires that firm 1 serves no old consumers, i.e. $d_1^o\le 0$, and serve some of the younger generation, i.e. $d_1^n\ge 0$. Notice that such a demand configuration is only possible if z(a)/Δ > Δ/2 + s, otherwise there are no prices which support such a configuration. In this case, firm 1 cannot even think of just serving the new customers as a possible deviation. Observe that

$$\phi(a)=\frac{\Delta}{2}+s-\frac{z(a)}{\Delta},$$

is increasing in a. Therefore, if φ(a ₀) is positive, then φ(a ^*) is positive, and hence, firm 1 cannot deviate to serve just the young. Evaluating φ(a ₀) yields

$$\begin{array}{lll} \phi(a_0)&=&s+\frac{1}{2}\,\Delta-1-\,{\frac {4 \beta\,{s}^{2}}{ \left(\Delta+ 2\,\beta\,s+2 \right) \Delta}}\\ &>&s+\frac{1}{2}\,\Delta-1-\,{\frac {2 \beta\,{s}}{ \left(\Delta+ 2\,\beta\,s+2 \right)}}\\ &=&\frac{1}{2}\,{\frac {{\Delta}^{2} +2\,s\,\Delta\, \left( 1 +\beta \right)+4\,s\, \left(1+ \beta\,s \right) -8\,\beta\,s-4}{\Delta+2\,\beta\,s+2}}=\hat \phi(a_0). \end{array}$$

where the second inequality follows from s ≤ Δ/2. It could be easily checked that, $\hat \phi(a_0)>0$, whenever Δ ≥ Δ₃, with Δ₃ as defined above. Thus, a (very strong) sufficient condition for firm 1 not deviate to a price to just serve the young consumers is Δ ≥ Δ₃.

• 3. Firm 1 cannot deviate to just serve the old consumers.

If the older generation is less price sensitive, firms might find it beneficial to just serve this segment by charging a higher price excluding the younger generation altogether. I will once again presume that firm 2 plays the candidate equilibrium policy, while, firm 1 considers a one period deviation. If firm 1 serves the old customers only, then in the next period it starts game with a zero installed base. Therefore, the deviation profit of firm 1 is simply given by

$$\pi_1^d=p_1d_1^o+\beta V_1(0).$$

The value of the future is governed by the same value function since firm 1 returns back to the equilibrium policy next period. The optimal deviation price of firm 1 can be computed via maximizing the deviation profits. First order conditions for this problem implies a deviation price of

$$p_1^d=\frac{1}{2}\,s\, \left( 2\,{x}^{t}-1 \right) +\frac{1}{4}\,\Delta+\frac{1}{2}\,p_{{2}}^*,$$

where $p_{{2}}^*=l^*+k^*(1-x^t)$. For this deviation to be possible at all, we need $d_1^n\le 0$ at $(p_1^d,p_2^*)$ as well as $d_1^o\ge 0$. Notice that $d_1^n\le 0$, if $p_1^d-p_2^*>z(a)/\Delta$, otherwise young will also purchase at the deviation price, implying that firm 1 will have to consider selling to young generation, in which case its optimal action is to follow the equilibrium policy. That is, I will find conditions on Δ such that

$$ \psi(a,x^t)=p_1^d-p_2^*-z(a)/\Delta <0. $$

First notice that

$$p_1^d-p_2^*=\frac{1}{2}\,s\, \left( 2\,{x}^{t}-1 \right) +\frac{1}{4}\,\Delta-\frac{l^*}{2}+\frac{a}{2}(1-x^t)$$

is increasing in x ^t, thus ψ(a,x ^t) is increasing in x ^t. Hence, $\psi(a,x^t)\le \psi(a,1)=\hat \psi(a)$. By substituting for l ^* and rearranging, one can obtain

$$\hat \psi(a)= \frac{s}{2}+\frac{\Delta}{4}-1-\frac{1}{4}a+\frac{2\beta s}{\Delta}a- {\frac { \left( 2 z(a) -\beta \Delta a \right) \Delta}{{\Delta}^{2}+2 \beta \Delta s+2 z(a) }}.$$

It could be easily verified that fourth term in the above expression is decreasing with a, while the fifth and sixth terms are increasing. Replacing a with a ₀ in the fourth term and with zero in the fifth and sixth term we arrive at

$$\bar \psi=\frac{s}{2}+\frac{\Delta}{4}-1+\frac { s} {2({\Delta} +2 \beta s + 2 )} -\frac {2 \Delta} {{\Delta} +2 \beta s + 2 }\ge \hat \psi(a).$$

The denominator in $\bar \psi$ is positive, and the numerator is quadratic-convex function of Δ. Thus, $\bar \psi \le 0$ in between the two values of Δ which make the numerator zero. One of these roots is negative, the one which could be positive is given by Δ₄ defined above.

I will briefly summarize the steps of the proof of the lemma. For Δ ≥ Δ₁, $a_0\ge \max({\underline{a}}_1,{\underline{a}}_2)$ and for Δ ≥ Δ₂, g(a ₀) ≤ 0, which combined with the fact that g(0) ≥ 0, implies that there is a root of g(a), a ^*, in [a ₀,0] and at this value of a ^*, $n_1^*$ is non-negative. A non-positive a ^* and a non-negative $n_1^*$ imply that $n_0^*$ and $n_2^*$ are also non-negative. There is no price a firm can deviate to such that it only serves the younger generation whenever Δ ≥ Δ₃. On the other hand, when a firm selects its optimal price in order to serve just the old, it faces a non-negative demand from younger ones if Δ ≤ Δ₄. Thus, it should take into account the demand from the younger ones when selecting its profit maximizing price. But in that case, the best it could do is to follow the equilibrium policy. Combining these conditions leads to the set ${\cal D}$ defined above.

Proof of Proposition 1

Proposition 1

(Existence of Markov Perfect Equilibrium) There is a set of parameters where a symmetric MPE in stationary affine strategies exists. The parameters of the affine policy functions and quadratic value functions are given by Lemma 1. In this equilibrium,

• 1. The firm with a higher customer base charges a higher price.

• 2. Each firm charges a price above cost, that is, the parameters of the policy functions, (l^*,k^*), are both non-negative.

• 3. Customers bases of both firms converge to the steady state in an oscillatory fashion.

• 4. Market is shared equally in the steady state.

• 5. At every period, switching occurs in both directions.

Proof

Lemma 3 establishes a characterization of a set of parameters such that when firms adopt the candidate policies given in Lemma 1, market is covered and shared, switching in both directions occurs, firms make nonnegative profits and neither firm deviates to serve just one segment. These candidate policies form an equilibrium if the objective functions, given in (11) and (12), used to derive them were concave in each firms own strategy. The second order condition implied by (11), after simplifications is given by

$$-\frac{1}{4}\,{\frac { \left( 2\,z \left( a^* \right) +{\Delta}^{2} \right) \left( 4\, z \left( a^* \right)^{2}-\beta\,{\Delta}^{2 }{a^*}^{2} \right) }{\Delta\, z \left( a^* \right)^{3}}}<0, $$

where the negativity follows from 4z(a ^*)² − βΔ a ^* > 3z(a ^*)² − βΔ a ^* > 0.¹⁹ Thus, the objective function of firms are indeed concave for relevant parameters and the solution of the first order conditions indeed describe an equilibrium. Lemma 1 characterizes the equilibrium values of (l,k), (n ₀,n ₁,n ₂) and a in this equilibrium.

• 1. The fact that k ^* = − a ^*, and a ^* < 0 implies that both firms employ pricing policies which are increasing in the size of their own customer base. Thus a firm with a large customer base charges a higher price in equilibrium.

• 2. The nonnegativity of k ^* immediately follows from the nonpositivity of a ^*. Recall that $0<\tilde n_1\le n_1^*$, where $\tilde n_1$ was defined in the proof Lemma 3. It is easy to verify that

  $$l(a)-\tilde n_1=-\frac{3}{2} a+{\frac { \left( 2 z(a) -\beta \Delta a \right) \left(\Delta-2s\right)}{{\Delta}^{2}+2 \beta \Delta s+2 z(a) }}\ge 0,$$

  for all a ∈ [a ₀,0], where l(a) is l ^* viewed as a function of a. Thus, l ^* = l(a ^*) is also nonnegative.

• 3. The coefficient of x ^t in (19) is negative at the equilibrium value of a. Thus, a firm which has a higher stock of old customers at time t, will capture less than half of the new customers. Therefore, at t + 1 this firm would also have a smaller customer base.

• 4. Once again, a brief inspection of (19) suggest that, whenever x ^t = 1/2, the next period customer base is also one half, i.e. x ^t + 1 = 1/2. Since prices of both firms are equal whenever x ^t = 1/2, equation (9) implies that firms will serve customers with a mass of one. The total market size is two, thus in the steady state firms share the market equally.

• 5. The equilibrium value of a satisfies (21), thus switching occurs in the both directions in equilibrium. This completes the proof.

□

Proof of Proposition 2

Proposition 2

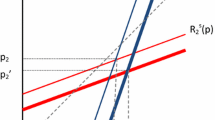
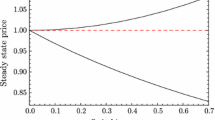
The steady state prices are decreasing in s, at s = 0. Therefore, for all the parameter values that yields an MPE described in Proposition 1, very small switching costs intensify competition.

Proof

After many cumbersome manipulations, the best response function for firm 1 at the steady state, i.e. when x ^t = 1/2, can be written as

$$R(p_2,s)=\frac{\left( 2 z \left( a \right)^{2}-\beta\,{\Delta}^{2}{a}^{2} \right) p_{ {2}}}{{4\, z \left( a \right) ^{2}-\beta\,{\Delta}^{2}{a}^{2}}} +\frac { 2\left( 2\,z \left( a \right) -\beta \Delta a\right) z \left( a \right) ^{2}\Delta} {({\Delta}^{2}+2\,s\, \beta\,\Delta+2\,z \left( a \right) )(4\, z \left( a \right) ^{2}-\beta\,{\Delta}^{2}{a}^{2})}, $$

therefore, the best response function of firm 1 is increasing in p ₂ if

$$\frac{\left( 2 z \left( a \right)^{2}-\beta\,{\Delta}^{2}{a}^{2} \right) }{{4\, z \left( a \right) ^{2}-\beta\,{\Delta}^{2}{a}^{2}}}\ge 0. $$

In particular, this term is equal to 1/2 when s = 0 and a = 0. Thus, around s = 0 strategies of firms are complements, and best response functions are increasing in each other’s strategies. This also holds away from s = 0, at least in the region of parameters that I have derived in Lemma 3. We know from lemma 2 that at the equilibrium value of a, 3z(a)² − βΔ ² a ² ≥ 0, therefore we only need to show that κ(a) = 2z(a)² − βΔ ² a ² ≥. Let

$$\rho_4=-\frac{\sqrt{2} \Delta}{\sqrt{\beta}\Delta-2\sqrt{2}\beta s}.$$

Whenever $\sqrt{\beta}\Delta-2\sqrt{2}\beta s<0$, it is easy to verify that κ(a) ≥ 0 for all a ≤ 0. On the other hand, whenever $\sqrt{\beta}\Delta-2\sqrt{2}\beta s\ge 0$, κ(a) ≥ 0 if a ≥ ρ ₄. It is easy to verify that a ₀ > ρ ₄, thus κ(a) ≥ 0 whenever ${\cal D} \ne \emptyset$ and $(\beta,\Delta,s) \in {\cal D}$. Therefore, the best response function of firm 1 is increasing in the price of firm 2 in the set of parameters derived in Lemma 3. Thus, the strategies of firms are strategic complements as expected. The steady state equilibrium prices will be decreasing in s as long as the best response function of firms shift downwards with an increase in s.

The derivative of the best response function of firm 1, R(p ₂,s), with respect to switching costs can be written as

$$\label{MCS1a} \frac{\partial}{\partial s}R(p_2,s)=\frac{\frac{\partial^2}{\partial s \partial p_1}\Pi_{1}(p_1,p_2,s)} {-\frac{\partial^2}{\partial {p_1}^2} \Pi_{1}(p_1,p_2,s)}, $$

(35)

where $\Pi_1(p_1,p_2,s)=p_1(d_1^o+d_1^y)+\beta V_1(d_1^y)$. This formulation assumes that in a particular period firm 2 charges p ₂ and firm 1 charges its best response to p ₂, namely p ₁, but they continue from the next period onwards to follow the equilibrium policies which form an MPE. It is clear from (35) that the sign of the change in the best response of firm 1 in response to a change in s is equal to the sign of the numerator in (35) due to concavity of Π ₁(p ₁,p ₂,s), that is

$$\mbox{sign}\bigg[\frac{\partial}{\partial s}R(p_2,s)\bigg]=\mbox{sign}\bigg[{\frac{\partial^2}{\partial s \partial p_1}\Pi_{1}(p_1,p_2,s)} \bigg]. $$

Notice that the numerator of (35) is nothing but the derivative of the FOC of firm 1 with respect to s holding p ₁ and p ₂ constant. Therefore, (35) provides information on the direction the FOC as a function p ₁ will move in response to a change in s.

The FOC of firm 1 can be written as

$$\begin{array}{lll} \frac{\partial}{\partial p_1}\Pi_{1}(p_1,p_2,s)&=&d_1^o+d_1^y+p_1\left(\frac{\partial d_1^o}{\partial p_1}+ \frac{\partial d_1^y}{\partial p_1}\right) \\ &&+\beta \left(n_1^*+2 n_2^*d_1^y\right)\frac{\partial d_1^y}{\partial p_1} \label{foc11a}. \end{array} $$

(36)

In taking the derivative of this expression, one should account for the dependence of a, $n_1^*$ and $n_2^*$ on s. With this caveat, taking the derivative of (36), holding p ₁ and p ₂ constant yields

$$\begin{array}{lll} \frac{\partial^2}{\partial s \partial p_1}\kern-.5pt\Pi_{1}\kern-.5pt(\kern-.5pt p_1,p_2,s\kern-.5pt)&=&\frac{\partial d_1^o}{\partial s}\kern-.5pt+\kern-.5pt \frac{\partial d_1^y}{\partial s}\kern-.5pt+\kern-.5pt \frac{\partial d_1^y}{\partial a} \frac{\partial a}{\partial s} \kern-.5pt+\kern-.5ptp_1\left(\frac{\partial^2 d_1^o}{\partial s \partial p_1}\kern-.5pt+\kern-.5pt \frac{\partial^2 d_1^y}{\partial s\partial p_1}\kern-.5pt+\kern-.5pt \frac{\partial^2 d_1^y}{\partial a\partial p_1}\frac{\partial a}{\partial s}\right) \\ &&+\beta \left(\frac{\partial n_1^*}{\partial s}+\frac{\partial n_1^*}{\partial a}\frac{\partial a}{\partial s}\right) \frac{\partial d_1^y}{\partial p_1}+\beta n_1^*\left(\frac{\partial^2 d_1^y}{\partial s \partial p_1} +\frac{\partial^2 d_1^y}{\partial a \partial p_1}\frac{\partial a}{\partial s}\right) \\ &&+2\beta \kern-1pt\left(\kern-.5pt\frac{\partial n_2^*}{\partial s}\kern-.5pt+\kern-.5pt\frac{\partial n_2^*}{\partial s}\frac{\partial a}{\partial s}\kern-.5pt\right)\kern-.5pt d_1^y\frac{\partial d_1^y}{\partial p_1}\kern-.5pt+\kern-.5pt2\beta n_2^* \kern-1pt\left(\kern-.5pt\frac{\partial d_1^y}{\partial s}\kern-.8pt+\kern-.8pt \frac{\partial d_1^y}{\partial a}\frac{\partial a}{\partial s}\kern-.5pt\right)\kern-1pt\frac{\partial d_1^y}{\partial p_1} \\ &&+2\beta n_2^* d_1^y \left(\frac{\partial^2 d_1^y}{\partial s \partial p_1}+\frac{\partial^2 d_1^y}{\partial a \partial p_1}\frac{\partial a}{\partial s}\right) \label{derp1s}. \end{array} $$

(37)

It is impossible to sign (37) in general, however, at s = 0 all but one of the terms in this expression is zero. First notice that when s = 0, the value of a that solves g(a) = 0 is identically zero. Also note that in the steady state equilibrium prices are equal, that is p ₁ = p ₂ and the customer base of a firm is simply $\frac{1}{2}$. I will present each derivative below and use the notation ≐ to imply $\Big\lvert_{s~=~0,a~=~0,x^t=\frac{1}{2},p_1=p_1^*(\frac{1}{2}),p_2=p_2^*(\frac{1}{2})}\Big.$, i.e., evaluated at the steady state equilibrium when s = 0. In the arguments below, I will not repeat that the exercise is performed at particular this point as long as no confusion is possible.

Let us start with the derivative of the equilibrium value of a with respect to s which is given by

$$\frac{\partial a}{\partial s}=\frac{-\frac{\partial g(a)}{\partial s}}{\frac{\partial g(a)}{\partial a}}\doteq -\frac{4}{3(\Delta +2)}.$$

This implies that as switching costs slightly increase consumers expect a price differential favoring the firm with a larger user base in the future. The demand from the younger generation becomes less elastic as switching costs increase slightly above zero. The various derivatives involving $d_1^o$ are

$$ {\ensuremath{\frac{\partial {d_1^o}}{\partial {s}}}}=\frac{2x^t-1}{\Delta}\doteq 0 \qquad {\ensuremath{\frac{\partial^2 {d_1^o}}{\partial {s} \partial {p_1}}}}=0\doteq 0. $$

The derivatives involving $d_1^y$ are complicated by the fact $d_1^y$ is also a function of a. They are given by

$$\begin{array}{lll} {\ensuremath{\frac{\partial {d_1^o}}{\partial {s}}}}=-\frac{\beta \Delta a(p_1-p_2)}{z(a)^2} \doteq 0 &\qquad& {\ensuremath{\frac{\partial{d_1^y}}{\partial {a}}}}=-\frac{\beta \Delta s (p_1-p_2)}{z(a)^2} \doteq 0\\ {\ensuremath{\frac{\partial^2 {d_1^y}}{\partial {s} \partial {p_1}}}}=\frac{\beta \Delta a}{z(a)^2} \doteq 0 &\qquad& {\ensuremath{\frac{\partial^2 {d_1^y}}{\partial {a} \partial {p_1}}}}=\frac{\beta \Delta s}{z(a)^2} \doteq 0 \end{array}$$

Also, note that

$${\ensuremath{\frac{\partial {d_1^y}}{\partial {p_1}}}}=-\frac{\Delta}{z(a)}\doteq -\frac{1}{2}.$$

The remaining terms involve the value function parameters $n_1^*$ and $n_2^*$. The derivatives of $n_2^*$ with respect to s and a are

$$ {\ensuremath{\frac{\partial {n_2^*}}{\partial {s}}}}=\frac{\beta \Delta a^3}{z(a)^2}\doteq 0 \qquad {\ensuremath{\frac{\partial {n_2^*}}{\partial {a}}}}=\frac{2a}{\Delta}+\frac{\Delta a^2}{2z(a)} \doteq 0. $$

And finally, the derivative of $n_1^*$ with respect to s is given by

$$\begin{array}{lll} {\ensuremath{\frac{\partial {n_1^*}}{\partial {s}}}}&=&-{\ensuremath{\frac{\partial {n_2^*}}{\partial {s}}}}+2\,{\frac { \left( 2\,z \left( a \right) -\beta\,a\Delta \right) \Delta\, \left( 2+\Delta \right) }{ \left( {\Delta}^{2}+2\,\beta\,s\, \Delta+2\,z \left( a \right) \right) ^{2}}}-8\,{\frac {\beta\,s\,a}{{ \Delta}^{2}+2\,\beta\,s\,\Delta+2\,z \left( a \right) }},\\ &=&\frac{4}{\Delta+2}, \end{array}$$

while with respect to a it is given by

$$\begin{array}{lll} {\ensuremath{\frac{\partial {n_1^*}}{\partial {a}}}}&=&-{\ensuremath{\frac{\partial {n_2^*}}{\partial {a}}}}+1-2\,{\frac {\beta\,s\,\Delta\, \left( \left( 4\,s+\Delta \right) \left( \Delta+2\,\beta\,s \right) +2\,\Delta \right) }{ \left( { \Delta}^{2}+2\,\beta\,s\,\Delta+2\,z \left( a \right) \right) ^{2}}} ,\\ &=&1. \end{array}$$

Given these results, it is clear that the only effect of a change in s on the FOC of firm 1 comes through its effects on $n_1^*$. In fact, the only non zero term in (37) is the first one on the third line. That is,

$$\begin{array}{lll} \frac{\partial^2}{\partial s \partial p_1}\Pi_{1}(p_1,p_2,s)&=&\beta (\frac{\partial n_1^*}{\partial s}+ \frac{\partial n_1^*}{\partial a}\frac{\partial a}{\partial s}) \frac{\partial d_1^y}{\partial p_1}\\ &=&\beta\left(\frac{4}{\Delta+2}+(1)\left(-\frac{4}{3(\Delta +2)}\right)\right)\left(-\frac{1}{2}\right)\\ &=&-\frac{4 \beta}{3(\Delta+2)}<0. \end{array}$$

Thus, the best response function of firm 1 will shift downwards when s increases slightly above zero. Similar arguments apply for the best response function of firm 2 as well. Therefore, a slight increase in switching costs will yield lower steady state equilibrium prices.□