North–South Asymmetry of Solar Meridional Circulation and Synchronization: Two Rings of Four Coupled Oscillators

Abstract

We apply the Kuramoto model with four coupled oscillators to the description of the phase evolution of solar magnetic field proxies at different latitudes. We show that a ring of four coupled oscillators does represent the frequency synchronization of the meridional circulation and the phase evolution of solar proxies. The model allows one to reconstruct the long-term evolution of the meridional flow speed and hemispheric asymmetry at different latitudes. We study the N-S asymmetry of the meridional flow and find a centennial variation of natural frequencies and couplings. Extremes of the north–south asymmetry of the near-equatorial meridional flow correspond to the anomalies of solar activity in Solar Cycles (SC)19–20 and SC23–24. We also find a degeneration of the meridional circulation ring profile in SC23–24, which agrees with helioseismic observations. We show that the N-S asymmetry depends on latitude and is strongly connected with the near-equatorial meridional flow.

Appendices

Appendix A: Stability of Synchronization

The stability of the stationary solution of Equation 8 is determined by negative eigenvalues of the symmetrical matrix

$$ M= \begin{pmatrix} a+b & -b & 0 & -a \\ -b & b+c+d & -d & -c \\ 0 & -d & d+f & -f \\ -a & -c & -f & a+c+f \end{pmatrix} $$

(16)

where

$$\begin{aligned} a=-\kappa_{HP}\cos(\Phi_{P}-\Phi_{H}),\quad b=-\kappa_{HM}\cos( \Phi_{H}-\Phi_{M}) \\ c=-\kappa_{MP}\cos(\Phi_{P}-\Phi_{M}),\quad d=-\kappa_{ML}\cos( \Phi_{M}-\Phi_{L}), \\ f=-\kappa_{LP}\cos(\Phi_{P}-\Phi_{L}). \end{aligned}$$

(17)

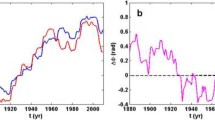
Let us note that cosines in $a$, $c$ and $f$ are negative and cosines in $b$ and $d$ are positive (Figure 1, right). The characteristic equation is

$$\begin{aligned} \mathit{det} (M-\lambda E)=0 , \end{aligned}$$

(18)

whose left side is simplified to

$$\begin{aligned} \begin{vmatrix} a+b-\lambda& -b & 0 & -a \\ -b & b+c+d-\lambda& -d & -c \\ 0 & -d & d+f-\lambda& -f \\ -a & -c & -f & a+c+f-\lambda \end{vmatrix} \\ =(a+b-\lambda) \begin{vmatrix} b+c+d-\lambda& -d & -c \\ -d & d+f-\lambda& -f \\ -c & -f & a+c+f-\lambda \end{vmatrix} \\ +b \begin{vmatrix} -b & -d & -c \\ 0 & d+f-\lambda& -f \\ -a & -f & a+c+f-\lambda \end{vmatrix} +a \begin{vmatrix} -b & b+c+d-\lambda& -d \\ 0 & -d & d+f-\lambda \\ -a & -c & -f \end{vmatrix} \\ =\lambda(\lambda^{3}-2\lambda^{2}(a+b+c+d+f)+ \\ +\lambda(3(ab+bc+ac+cd+bd+af+cf)+4ad+4bf))- \\ -4(abd+abf+bcd+acd+acf+bcf+bdf))=0. \end{aligned}$$

(19)

Equation 19 has all non-positive solutions only when all its coefficients are non-negative. Then we have the following inequalities:

$$\begin{aligned} a+b+c+d+f< 0, \\ 3ab+3bc+3ac+4ad+3bd+3cd+3af+3cf+4bf+3df>0, \\ abf+acd+abd+bcd+adf+acf+bcf+bdf< 0. \end{aligned}$$

(20)

The stable stationary solution in the minimal model is determined by three non-zero coefficients $A$, $B$, $C$ with the imposed inequalities:

$$ A+B+C< 0, \quad3AB+4BC+3AC>0, \quad ABC< 0 .$$

(21)

To satisfy these three inequalities, all coefficients should be negative: $A<0$, $B<0$, $C<0$.

Let us now consider the minimal models one by one and resolve them for the constant mean values of coupling and natural frequencies relevant to the synchronization when $\dot{\Phi}_{i}=0$. The synchronized solution of Equation 8 satisfies the following equations:

$$\begin{aligned} \Omega-\omega_{H}= \kappa_{HP}\sin(\Phi_{P}-\Phi_{H})+ \kappa_{HM} \sin(\Phi_{M}-\Phi_{H}) \\ \Omega-\omega_{M}= \kappa_{HM}\sin(\Phi_{H}-\Phi_{M})+ \\ {}+\kappa_{MP}\sin(\Phi_{P}-\Phi_{M}) +\kappa_{ML}\sin(\Phi_{L}- \Phi_{M}), \\ \Omega-\omega_{L}= \kappa_{ML}\sin(\Phi_{M}-\Phi_{L}) +\kappa_{LP} \sin(\Phi_{P}-\Phi_{L}), \\ \Omega-\omega_{P}= \kappa_{LP}\sin(\Phi_{L}-\Phi_{P})+ \\ {}+\kappa_{MP}\sin(\Phi_{M}-\Phi_{P}) +\kappa_{HP}\sin(\Phi_{H}- \Phi_{P}). \end{aligned}$$

(22)

We use the following properties of the mean values of observed phase differences:

$$\begin{aligned} \langle\sin(\Phi_{H}-\Phi_{P})\rangle< 0, \quad\langle\sin( \Phi_{L}-\Phi_{P})\rangle>0, \\ \langle\sin(\Phi_{H}-\Phi_{M})\rangle>0, \quad\langle\sin( \Phi_{L}-\Phi_{M})\rangle< 0, \end{aligned}$$

(23)

where the average is taken over any long time interval greater than one solar cycle, excluding the interval of the anomalous Solar Cycle 20. We test that the coherence of coupling and natural frequencies, which agrees the stability of the stationary solution (Equation 20) does not contradict the condition of synchronization (Equation 22). The contradiction may appear if the clockwise rotation determines positive left side of one of equations in Equation 22, when the phases difference and coupling determine the negative right side.

H-M-L-P:

The negative values of $b$, $d$, $f$ determine $\kappa_{HM}>0$, $\kappa_{ML}>0$ and $\kappa_{LP}<0$. Then three cells P, H, and L rotate clockwise and the M-cell rotates counter-clockwise. The two terms in the right side of the second equation of Equation 22 have different signs, so we do not find a contradiction with the positive of left side $\Omega-\omega_{M}$. $\Omega-\omega_{P}$ is negative in agreement with current observations.

P-H-M-L:

The negative values of $a$, $b$, $d$ determine $\kappa_{HP}<0$, $\kappa_{HM}>0$ and $\kappa_{ML}>0$. Then three cells P, H, and L rotate clockwise and the M-cell rotates counter-clockwise. As above there is no contradiction with the positive $\Omega-\omega_{M}$, but the positive right side determines $\Omega-\Omega_{P}>0$ in contradiction with current observations $\omega_{P}>\Omega$. Thus, in terms of this model the current observations of the near-surface meridional flow are not representative on a long time span.

H-P-M-L:

The negative values of $a$, $c$, $d$ determine $\kappa_{HP}<0$, $\kappa_{HM}>0$ and $\kappa_{ML}>0$. Then three cells P, H, and M rotate clockwise and the L-cell rotates counter-clockwise. There is no contradiction with the positive left side $\Omega-\omega_{L}$, but $\Omega-\Omega_{P}>0$ in contradiction with current observations $\omega_{P}>\Omega$. Thus, the current observations appear to be not representative.

H-M-P-L:

The negative values of $b$, $c$, $f$ determine $\kappa_{HM}>0$, $\kappa_{MP}<0$ and $\kappa_{LP}<0$. Then P, M and L cells rotate counter-clockwise and H-cell rotates clockwise. The positive left side $\Omega-\omega_{H}$ contradicts the negative right side of the same equation.

H-P-L-M:

The negative values of $a$, $d$, $f$ determine $\kappa_{HP}>0$, $\kappa_{ML}>0$ and $\kappa_{LP}<0$. Then P, H and L cells rotate counter-clockwise and M-cell rotates clockwise. The positive left side $\Omega-\omega_{M}$ contradict the negative left side of the same equation.

M-H-P-L:

The negative values of $a$, $b$, $f$ determine $\kappa_{HP}>0$, $\kappa_{HM}>0$ and $\kappa_{LP}<0$. Then P, H and L cells rotate counter-clockwise and M-cell rotates clockwise. There is no contradiction between $\Omega-\omega_{M}>0$ and the positive right side.

P-model:

The negative values of $a$, $c$, $f$ determine $\kappa_{HP}>0$, $\kappa_{MP}<0$ and $\kappa_{LP}<0$. Then all cells rotate counter-clockwise. There is no clockwise rotating cells, therefore there is no contradiction.

M-model:

The negative values of $b$, $c$, $d$ determine $\kappa_{HM}>0$, $\kappa_{MP}<0$ and $\kappa_{ML}>0$. Then P and M cells rotate counter-clockwise, H and L cells rotate clockwise. There is a contradiction between positive $\Omega-\omega_{H}$ and the negative right side $\kappa_{HM}\sin(\Phi_{M}-\Phi_{H})$.

Appendix B: The Ring Model

Chains M-H-P-L and H-M-L-P are stable under the assumptions $\omega_{P}>\Omega$, $\omega_{L}<\Omega$, $\omega_{H}>2\Omega$. Chains M-H-P-L and P-H-M-L are stable under the assumptions $\omega_{P}<\Omega$, $\omega_{L}<\Omega$, $\omega_{H}>2\Omega$. Solving the inverse problem we first reconstruct the evolution of coupling for constant natural frequencies and then reconstruct the evolution of natural frequencies for constant coupling. The above restrictions with the condition restrict possible values of constant natural frequencies.

2.1 B.1 Coupling Reconstruction

The reconstruction of coupling for the chain H-M-L-P is determined by the equations

$$\begin{aligned} \kappa_{ML}(t)= \frac{2\Omega-\omega_{L}-\omega_{P}}{\sin(\Phi_{M}(t)-\Phi_{L}(t))}, \qquad\kappa_{HM}(t)= \frac{\Omega-\omega_{H}}{\sin(\Phi_{M}(t)-\Phi_{H}(t))}, \\ \kappa_{LP}(t)= \frac{\Omega-\omega_{P}}{\sin(\Phi_{L}(t)-\Phi_{P}(t))}. \end{aligned}$$

(24)

The reconstruction of coupling for the chain M-H-P-L is determined by the equations

$$\begin{aligned} \kappa_{HP}(t)= \frac{2\Omega-\omega_{L}-\omega_{P}}{\sin(\Phi_{H}(t)-\Phi_{P}(t))}, \qquad\kappa_{HM}(t)= \frac{\Omega-\omega_{M}}{\sin(\Phi_{H}(t)-\Phi_{M}(t))}, \\ \kappa_{LP}(t)= \frac{\Omega-\omega_{L}}{\sin(\Phi_{P}(t)-\Phi_{L}(t))}. \end{aligned}$$

(25)

The reconstruction of coupling for the chain P-H-M-L is determined by the equations:

$$\begin{aligned} \kappa_{HP}(t)= \frac{\Omega-\omega_{P}}{\sin(\Phi_{H}(t)-\Phi_{P}(t))},\qquad \kappa_{HM}(t)= \frac{2\Omega-\omega_{M}-\omega_{L}}{\sin(\Phi_{H}(t)-\Phi_{M}(t))} \\ \kappa_{ML}(t)= \frac{\Omega-\omega_{L}}{\sin(\Phi_{M}(t)-\Phi_{L}(t))} \end{aligned}$$

(26)

At time $t$ when the value of sine is smaller than a threshold value $h=0.1$ it is replaced by its average estimated over the whole time interval of observations.

An example of the coupling reconstruction for the chain P-H-M-L is presented in Figure 7. The evolution of $\kappa_{ML}$ follows the evolution of the solar cycle frequency $\Omega(t)$ with correlation coefficient 0.76 in the northern and 0.86 in the southern hemisphere.

Figure 7

Evolution of coupling coefficient (from left to right): $\kappa_{HP}$, $\kappa_{HM}$, $\kappa_{ML}$ in the northern (blue) and southern (red) hemisphere reconstructed from the chain models P-H-M-L assuming the mean frequency $\Omega$ to be constant (top row) or variable (bottom row). Parameters of natural frequencies in the chain model are $\omega_{H}=1.8$, $\omega_{L}=0.5$, $\omega_{P}=0.55$, $\omega_{M}=-0.51$.

The coupling evolution reconstructed through any of the stable chain model is practically the same (Figure 8)

Figure 8

Evolution of coupling coefficients (from left to right): $\kappa_{HP}$, $\kappa_{HM}$, $\kappa_{ML}$ in the northern (blue) and southern (red) hemisphere reconstructed from the chain models (from top to bottom) H-M-L-P, M-H-P-L for $\omega_{P}>\Omega$, M-H-P-L for $\omega_{P}<\Omega$, P-H-M-L assuming the mean frequency $\Omega$ to be constant. Parameters of natural frequencies in the chain models H-M-L-P, M-H-P-L ($\omega_{P}>\Omega$) are $\omega_{H}=1.8$, $\omega_{L}=0.3$, $\omega_{M}=-0.56$, $\omega_{P}=0.8$ in the chain models M-H-P-L ($\omega_{P}<\Omega$), P-H-M-L: $\omega_{H}=1.8$; $\omega_{L}=0.5$, $\omega_{M}=-0.51$, $\omega_{P}=0.55$.

2.2 B.2 Reconstruction of Natural Frequencies

Now we estimate the evolution of natural frequencies considering coupling values to be equal to their average values over the whole time span (see Table 2). The average couplings are estimated for constant $\Omega=\Omega_{0}$ and substituted into Equation 22. In order to ensure stability we estimate coupling in the chain model H-M-L-P under the assumption $\omega_{P}>\Omega$, in the chain model P-H-M-L under the assumption $\omega_{P}<\Omega$, and in the chain model M-H-P-L in both cases. The reconstruction of natural frequencies is performed for $\Omega=\Omega(t)$ (Figure 9).

Figure 9

Evolution of natural frequencies (from left to right) $\omega_{H}$, $\omega_{M}$, $\omega_{L}$ and $\omega_{P}$ in the northern (blue) and southern (red) hemisphere reconstructed from the chain model (from top to bottom) H-M-L-P, M-H-P-L ($\omega_{P}>\Omega_{0}$), M-H-P-L ($\omega_{P}<\Omega_{0}$), P-H-M-L. The coupling coefficients are given in Table 2.

2.3 B.3 Quality of the Reconstruction

Let us compare the reconstructed phases with the original ones in the stable chain models belonging to the same ring. The quality of the reconstruction is evaluated by the correlation coefficients computed in a centered sliding window T = 11 yr. Figure 10 compares the quality of the reconstruction in the stable chain models. We see that the best reconstruction in the northern hemisphere is provided by the chain M-H-P-L (Figure 10, top middle), and the reconstruction of the polar field by the model P-H-M-L fails in the southern hemisphere (Figure 10, bottom right).

Figure 10

Correlation between original and simulated phases of the H (blue), M (red), L (yellow) and P (purple) cells for constant couplings and variable natural frequencies reconstructed for the same parameters as Figure 7 in the stable chain models of the same ring (from left to right) H-M-L-P and M-H-P-L for $\omega_{P}>\Omega$, M-H-P-L and P-H-M-L for $\omega_{P}<\Omega$ in the northern (top row) and southern (bottom row) hemisphere. The solar cycle frequency is taken $\Omega=\Omega(t)$.

2.4 B.4 WSO Polar Field Index

Starting from 1976 we can perform the above reconstructions with the WSO polar field index. Helioseismic observations of the near-surface meridional flow cover most of the time period from 1976 to 2017 and report its speed to be above 10 m s⁻¹ (Hathaway and Upton, 2014). Assuming the surface circulation cell to extend from equator to pole and up to $0.1~R_{\odot}$ in depth we get $\omega_{P}>\Omega$. So for the time period 1976–2017 only two chain models are valid: H-M-L-P and M-H-P-L. Figure 11 compares the coupling reconstruction in this two chains for the two polar field indices $P(t)$ and $W(t)$. The difference manifested in the southern hemisphere is related to the phase lag between indices $P$ and $W$ (Figure 1, right column). The closeness of $\sin(\Phi_{W}(t)-\Phi_{H}(t))$ determines jumps of the coupling coefficient $\kappa_{HP}$ in the southern hemisphere.

Figure 11

Evolution of coupling coefficients in the chain models H-M-L-P (top) $\kappa_{HM}$, $\kappa_{ML}$, $\kappa_{LP}$ and M-H-P-L (bottom) $\kappa_{HM}$, $\kappa_{HP}$, $\kappa_{LP}$ in the northern (blue) and southern (red) hemisphere reconstructed for WSO (solid) and polar faculae (dashed) polar field indices. Reconstruction is performed for constant natural frequencies $\omega_{H}=1.8$, $\omega_{L}=0.3$, $\omega_{M}=-0.56$, $\omega_{P}=0.8$.