Abstract
In this paper a principle of substitutivity of logical equivalents salve veritate and a version of Leibniz’s law are formulated and each is shown to cause problems when combined with naive truth theories.
Similar content being viewed by others
Notes
It is important to distinguish this from a weaker notion of logical equivalence that obtains when A and B entail each other. Given modus ponens and conjunction elimination two sentences are equivalent in my sense only if they are equivalent in this sense. In logics without conditional proof, however, the converse does not hold.
Moreover, given this incompleteness, the dependence on the choice of formal system can begin to seem arbitrary.
These might be a class of many valued models described in a classical set theory, as Field does in (2008). Alternatively one could run the model theory within a non-classical theory of sets as suggested in Bacon (2013b). In the former case one can expect ‘S is logically true’ to behave classically (i.e. it will conform to the laws of classical logic) whereas in the latter one cannot. The paradoxes I consider in what follows do not assume that these notions behave classically.
See Field (2010). It should be noted that I am adopting Field’s initial rough way of describing the view. The precise view is a bit more intricate, especially as it is designed to given a general account of logical consequence with multiple premises and multiple conclusions. The precise account of logical truth (the 0 premise 1 conclusion instance of this theory) requires that A is a logical truth (if and) only if ones credence in A must be 1 conditional on fully accepting C and fully rejecting D for any C and D. It’s also worth noting that Field does not take claims about what we should believe to constitute an analysis of validity, although for my purposes material equivalence is sufficient.
And more generally, it allows us to substitute logically equivalent sentences in valid arguments in such a way as to preserve validity.
To put it more transparently, someone who accepts the truth of A ought to be able to say "for any B and C, if B and C are equivalent A[B/C] is true". Of course, one might quibble that this formulation of the quantified statement in terms of a conditional is an adequate paraphrase, but this just pushes the problem back a step—analogous paradoxes can be formulated using whatever resources one needs to account for the quantified statement.
The relevant sense of ‘ought’ presumably is not so objective as to not depend on our evidence, but this is not at issue here since the two principles in question seem to be a priori.
Although not necessarily uncontentious. Some reject I(\(\dot{=})\) when the arguments are non-denoting terms.
Indeed there are many different non-equivalent ways of stating Leibniz’s law in non-classical logics; see for example the discussion in Priest (2001), sections 24.6 and 24.7.
The assumption in question is the rule \(A\rightarrow (B\rightarrow C), B\vdash (A\rightarrow C)\).
I am grateful to Hartry Field and an anonymous referee for drawing my attention to this. In Łukasiewicz’s three valued logic we can ensure that instances of LL(\(\dot{=}\)) where A is atomic come out true if we stipulate that for every atomic predicate F, the value of Fa and Fb differs by no more than 1 minus the value of a = b. However, if the value of a = b is a half, Fa one and Fb a half then \(\neg (Fa \rightarrow \neg Fa)\) will have value one but \(a=b \rightarrow \neg (Fb \rightarrow \neg Fb)\) will have value a half. See also the discussion of Leibniz’s law in relevant logics in 24.6 and 24.7 of Priest (2001).
If we define a determinacy operator as \(A\wedge \neg(A\rightarrow \neg A)\) and take Field’s three-valued model to guide us in what to assert we get even more bizarre commitments. For example, one would have to assert that a is F, b is not F and that it’s not determinate that a is distinct from b.
In some of the theories we will discuss one can a putatively stronger notion of logical equivalence using a so called fusion connective, \(\circ: V(\ulcorner {((A\rightarrow B) \circ (B\rightarrow A))}\urcorner\). In the following arguments substituting this notion weakens the premises even further.
I should say, however, that I only do this to simplify the discussion. Notions applying to sentences, such as logical equivalence, are clearly quite different from the notion of propositional identity expressed using a connective, for example.
For example, if we set A to be the formula Tr(S) where S is a name for the sentence ϕ[Tr(S)/B] then the T-schema gives us that \(Tr(S) \leftrightarrow \phi[Tr(S)/B]\) as required).
Alternatively one could take the notion of logical necessity as primitive and define a notion of logical equivalence as \(\square((A\rightarrow B)\wedge(B\rightarrow A))\).
Suppose you can prove A, so \(\vdash A\), and by I, you also have \(\vdash A=A\). All one needs then is enough conditional logic to infer that \(A\leftrightarrow (A=A),\) from which one can infer A = (A = A) by RE.
This axiom assumes that there is a such constant. One can define such a thing in the truth and property theories considered provided you have an axiom of universal instantiation (for example, in a naïve truth theory you can achieve this by identifying \(\bot\) with ∀ xTr(x).)
As before I present these arguments with a connective, A ⇒ B, for expressing the fact that A entails B, rather than a predicate \(V(\cdot, \cdot)\) for ease of reading. As mentioned before, the differences are insubstantial when there is a predicate, Tr, such that A and \(Tr(\ulcorner {A}\urcorner)\) are intersubstitutable.
In Priest’s set-up things are complicated by the fact that he has two conjunction symbols. According to one of these PMP is valid and according to the other CI is, however neither makes both principles true at once.
See, for example, the incompleteness of axiomatic systems of second order logic with respect to the semantic notion of validity for those languages. More to the point: the concept of validity which Field endorses in (2008) is highly non-recursive (see Welch 2008) and so has no complete axiomatisation. Note that to infer that a rule preserves validity from the fact that it preserves provability requires both soundness and completeness (whereas to infer that a rule or principle is valid from the fact that it is provable one only needs soundness).
By ‘provable’ I mean ‘provable from the axioms and rules of the background logic and the rule RN.’ The principle RN is thus impredicative and allows us to prefix arbitrary strings of □’s to theorems. In fact the proofs we present only apply RN once or twice in a give proof, so this aspect of the strength RN is not really the issue.
The ‘paradoxes of provability’ can be represented by completely determinate (albeit unprovable) facts about the natural numbers, as Gödel has showed, but it is not obvious that the paradoxes of validity need to be completely determinate (see for example Schiffer 2003). Indeterminate validities arise even in the context of model theoretic accounts of validity if the model theory is carried out in a non-classical metatheory (see Bacon 2013b).
Thus, of course, this argument does not apply directly to those who respond to the semantic paradoxes by denying the transitivity of entailment [such as Ripley 2013 and Weir (this volume)].
A referee has pointed out to me that these proofs rely on the fact that we can apply Sub to make substitutions of the same formula within the scope of = at different depths. If you restricted Sub to permit only substitutions of the same depth, then one would need to use it twice and apply contraction.
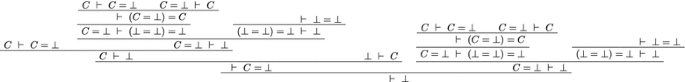
For example each instance of the schema \(Pr_{FS}(\ulcorner {A\leftrightarrow B}\urcorner) \rightarrow (Tr(\ulcorner {C}\urcorner)\rightarrow Tr(\ulcorner {C[A/B]}\urcorner),\) where Pr FS is an arithmetical formula expressing provability in FS , is true in the revision sequence described in Friedman and Sheard (1987) (Pr FS can in fact be strengthened to ‘provability from FS and true arithmetic’). Differences between the motivations of this kind of theory unfortunately prevent a direct comparison to the non-classical approach.
References
Bacon A (2013a) A new conditional for naive truth theory. Notre Dame J Formal Log 54(1):87–104
Bacon A (2013b) Non-classical meta-theory for non-classical logic. J Philos Log 42(2):335–355
Beall JC (2009) Spandrels of truth. Oxford University Press, USA
Beall JC, Murzi J (2013) Two flavors of curry paradox. J Philos CX(5):143–165
Brady R (2006) Universal logic. CSLI Publications, Stanford
Cresswell MJ (1967) Propositional identity. Logique et Analyse 40:283–291
Field H (2008) Saving truth from paradox. Oxford University Press, USA
Field H What is logical validity? In Colin C, Ole H (eds) Foundations of logical consequence. Oxford University Press, Oxford (forthcoming)
Friedman H, Sheard M (1987) An axiomatic approach to self-referential truth. Ann Pure Appl Log 33:1–21
Grišin VN (1981) Predicate and set-theoretical calculi based on logic without the contraction rule, mathematical ussr izvestiya 18 (1982), 41–59. English translaion, Izvéstia Akademii Nauk SSSR, 45:47–68
Halbach V (1994) A system of complete and consistent truth. Notre Dame J Formal Log 35(3):311–327
Murzi J, Shapiro L (2013) Validity and truth-preservation. In: Unifying the philosophy of truth. Springer (forthcoming)
Priest G (2001) An introduction to non-classical logic. Cambridge University Press, Cambridge
Priest G (2006) In contradiction: a study of the transconsistent. Oxford University Press, Oxford
Priest G Fusion and confusion. Topoi (forthcoming)
Ripley D (2013) Paradoxes and failures of cut. Aust J Philos 91(1):139–164
Schiffer S (2003) The things we mean. Clarendon Oxford, Oxford
Welch P (2008) Ultimate truth vis-a-vis stable truth. Rev Symb Log 1(01):126–142
Whittle B (2004) Dialetheism, logical consequence and hierarchy. Analysis 64(284):318–326
Zardini E (2013) Naive modus ponens. J Philos Log 42(4):1–19
Author information
Authors and Affiliations
Corresponding author
Additional information
I’d like to thank Hartry Field for some extremely helpful comments on an earlier draft of this paper, and three referees for this journal for their many helpful suggestions and corrections.
Rights and permissions
About this article
Cite this article
Bacon, A. Paradoxes of Logical Equivalence and Identity. Topoi 34, 89–98 (2015). https://doi.org/10.1007/s11245-013-9193-8
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11245-013-9193-8





