Coherent-Incoherent Transition of ε-Carbide in Steels Found with Mechanical Spectroscopy

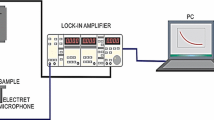
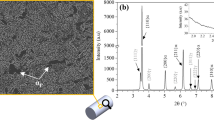
Abstract

Although a coherent-incoherent transition in the ε-carbide precipitated in steels is supposedly linked to hardening and microstructural changes, the existence of this transition has not yet been confirmed. In this paper, we investigate this subject using mechanical spectroscopy. By measuring mechanical loss spectra below room temperature of quench-aged Fe-C alloys, mild steel, and pearlitic steel, we reveal a new broad peak (NBP). This peak is related to thermal activation, and its line shape obeys the equation of the Debye peak with a distribution in relaxation time. The Arrhenius plot yielded a large activation energy and gigantic pre-exponential factor. Its intensity grew by aging at temperatures where precipitation of ε-carbide has been reported. However, it starts to decay at duration far too early for ε-carbide to transform to cementite. For isothermal aging at 393 K (120 °C), the intensity sharply decreased at durations over 3 hours. This decay was accompanied by appearance of another similar peak (NBP′), which had a peak frequency two orders higher than that of NBP. These peaks had comparable intensity. We attribute NBP and NBP′ to coherent and incoherent ε-carbides, respectively. We produced a model that attributes the relaxation peaks to reorientations of extra carbon pairs in the ε-carbide. The extraordinary values of the Arrhenius parameters may be interpreted by using this model. Based on these results, we assert that mechanical spectroscopy can detect the coherent-incoherent transition in carbon steels. This method will be powerful in studying problems related to the coherency in carbon steels.

Appendices

Appendix A. Debye equation

When a stress, σ = σ ₀exp (iωt), is applied to an anelastic solid, the real part of the complex compliance is given by

$$ J_{1} \left( \omega \right) = J_{\text{U}} + {{\delta J} \mathord{\left/ {\vphantom {{\delta J} {\left( {1 + \omega^{2} \tau^{2} } \right)}}} \right. \kern-0pt} {\left( {1 + \omega^{2} \tau^{2} } \right)}}, $$

(A1)

and the imaginary part by

$$ J_{2} \left( \omega \right) = {{\delta J \cdot \omega \tau } \mathord{\left/ {\vphantom {{\delta J \cdot \omega \tau } {\left( {1 \, + \omega^{2} \tau^{2} } \right)}}} \right. \kern-0pt} {\left( {1 \, + \omega^{2} \tau^{2} } \right)}}. $$

(A2)

Here, ω is the circular frequency and τ is the relaxation time. The quantity δJ is defined by

$$ \delta J = J_{\text{R}} {-}J_{\text{U}} , $$

(A3)

which is called the relaxation of the compliance and is a measure of the magnitude of the relaxation effect. The suffixes R and U stand for relaxed and unrelaxed, respectively.

An expression for the internal friction, Q ⁻¹, is described by

$$ Q^{ - 1} = \tan \varphi = {{J_{2} } \mathord{\left/ {\vphantom {{J_{2} } {J_{1} }}} \right. \kern-0pt} {J_{1} }} = {\Delta \mathord{\left/ {\vphantom {\Delta {(\surd (1 + \Delta ))}}} \right. \kern-0pt} {(\surd (1 + \Delta ))}} \cdot {{\omega \tau } \mathord{\left/ {\vphantom {{\omega \tau } {\left( {1 \, + \omega^{2} \tau^{2} } \right)}}} \right. \kern-0pt} {\left( {1 \, + \omega^{2} \tau^{2} } \right)}} \approx {{\Delta \cdot \omega \tau } \mathord{\left/ {\vphantom {{\Delta \cdot \omega \tau } {\left( {1 \, + \omega^{2} \tau^{2} } \right)}}} \right. \kern-0pt} {\left( {1 \, + \omega^{2} \tau^{2} } \right)}}, $$

(A4)

where Δ is defined by δJ/J _U. The Q ⁻¹ has a maximum of Δ/2 at ωτ = 1. Because the inverse of J ₁ equals the modulus of the solid M, the change in relative modulus between the unrelaxed and relaxed states is related to Δ by

$$ {{\delta M} \mathord{\left/ {\vphantom {{\delta M} {M_{\text{R}} }}} \right. \kern-0pt} {M_{\text{R}} }} = {{\left( {M_{\text{U}} - M_{\text{R}} } \right)} \mathord{\left/ {\vphantom {{\left( {M_{\text{U}} - M_{\text{R}} } \right)} {M_{\text{R}} }}} \right. \kern-0pt} {M_{\text{R}} }} = {{\delta J} \mathord{\left/ {\vphantom {{\delta J} {J_{\text{U}} }}} \right. \kern-0pt} {J_{\text{U}} }} = \Delta \simeq 2Q^{ - 1} \left( {{\text{at}}\,{\text{peak}}} \right) \, . $$

(A5)

Appendix B. Debye Equation with a Distribution in Relaxation Time

The normalized Gaussian distribution function is given as

$$ \Psi \left( z \right) = \frac{1}{\beta \surd \pi }\exp \left[ { - \left( {\frac{z}{\beta }} \right)^{2} } \right] $$

(B1)

Here z = ln(τ/τ _m), where τ _m is the most probable value of τ. The quantity β measures the half-width of the distribution at the point where Ψ(z) falls to 1/e of its maximum value, Ψ(0). In this case, equations (A1) and (A2) are reformulated as

$$ J_{1} \left( \omega \right) = J_{\text{U}} + \delta J\mathop \smallint \limits_{ - \infty }^{\infty } \left[ {{{\Psi (\ln \tau )} \mathord{\left/ {\vphantom {{\Psi (\ln \tau )} {\left( {1 + \omega^{2} \tau^{2} } \right)}}} \right. \kern-0pt} {\left( {1 + \omega^{2} \tau^{2} } \right)}}} \right]d\ln \tau $$

(B2)

and

$$ J_{2} \left( \omega \right) = \delta J \mathop \smallint \limits_{ - \infty }^{\infty } \left[ {{{\Psi (\ln \tau )\omega \tau } \mathord{\left/ {\vphantom {{\Psi (\ln \tau )\omega \tau } {\left( {1 + \omega^{2} \tau^{2} } \right)}}} \right. \kern-0pt} {\left( {1 + \omega^{2} \tau^{2} } \right)}}} \right]d\ln \tau, $$

(B3)

respectively.