Predator–prey systems in streams and rivers

Abstract

Many predator–prey systems are found in environments with a predominantly unidirectional flow such as streams and rivers. Alterations of natural flow regimes (e.g., due to human management or global warming) put biological populations at risk. The aim of this paper is to devise a simple method that links flow speeds (currents) with population retention (persistence) and wash-out (extinction). We consider systems of prey and specialist, as well as generalist, predators, for which we distinguish the following flow speed scenarios: (a) coexistence, (b) persistence of prey only or (c) predators only (provided they are generalists), and (d) extinction of both populations. The method is based on a reaction–advection–diffusion model and traveling wave speed approximations. We show that this approach matches well spread rates observed in numerical simulations. The results from this paper can provide a useful tool in the assessment of instream flow needs, estimating the flow speed necessary for preserving riverine populations.

Appendices

Appendix A: (Non-)dimensional models

Our starting point is a dimensional model of prey N and predators P at time t and spatial location x:

$$ \begin{array}{lll} \frac{\partial}{\partial t} N(x,t) & = & -v_N \frac{\partial}{\partial x} N(x,t) + D_N \frac{\partial^2}{\partial x^2} N(x,t)\\ && + f(N, P) N , \\ \frac{\partial}{\partial t} P(x,t) & = & -v_P \frac{\partial}{\partial x} P(x,t) + D_P \frac{\partial^2}{\partial x^2} P(x,t) \\ &&+ g(N, P) P. \end{array} $$

The downstream advection speeds experienced by the prey and predators are denoted by v _N and v _P , respectively. The diffusivities describing random movement are D _N for the prey and D _P for the predators. The growth function of the prey is given by

$$ f(N, P) = r \left(1-\frac{N}{K}\right) - a P , $$

where r is the intrinsic growth rate, K is the carrying capacity, and a is the predation rate. The growth rates of specialist and generalist predators are differently defined as follows:

A.1 Specialist predators

For specialist predators, we assume

$$ g(N, P) = e a N -m , $$

where e is the trophic conversion efficiency and m the mortality rate. Introducing the dimensionless quantities

$$ \begin{array}{llllll} \tilde{N} & = \dfrac{N}{K} ~, & \qquad \tilde{P} & = \dfrac{P}{e K} ~, & \qquad \tilde{t} & = e a K t ~, \\[12pt] \tilde{x} & = \sqrt{\dfrac{e a K}{D_N}} x ~, & \qquad v & = \dfrac{v_N}{\sqrt{e a K D_N}} ~ , & \qquad \alpha & = \dfrac{r}{e a K} ~, \\[12pt] \delta & = \dfrac{v_P}{v_N} ~, & \qquad \varepsilon & = \dfrac{D_P}{D_N} ~, & \qquad \mu & = \dfrac{m}{e a K}, \end{array} $$

we arrive at the following dimensionless system:

$$ \begin{array}{lll} \frac{\partial}{\partial \tilde{t}} \tilde{N} & = & -v \frac{\partial}{\partial \tilde{x}} \tilde{N} + \frac{\partial^2}{\partial \tilde{x}^2} \tilde{N} + \alpha \tilde{N} (1-\tilde{N}) -\tilde{N} \tilde{P}~, \\ \frac{\partial}{\partial \tilde{t}} \tilde{P} & = & -\delta v \frac{\partial}{\partial \tilde{x}} \tilde{P} + \varepsilon \frac{\partial^2}{\partial \tilde{x}^2} \tilde{P} + \tilde{N} \tilde{P} -\mu \tilde{P} ~. \end{array} $$

Dropping the tildes for notational convenience gives the dimensionless Eqs. 1–4.

A.2 Generalist predators

For generalist predators, we assume

$$ g(N, P) = e a N +b \left(1-\frac{P}{K_P}\right) , $$

where e again is the trophic conversion efficiency. Due to alternative food sources available, the predators grow logistically with intrinsic growth rate b and carrying capacity K _P . Introducing the dimensionless quantities

$$ \beta = \frac{b}{a K_P} , \qquad \kappa = \frac{K_P}{e K}, $$

we arrive at the following dimensionless system:

$$ \begin{array}{lll} \frac{\partial}{\partial \tilde{t}} \tilde{N} & = & -v \frac{\partial}{\partial \tilde{x}} \tilde{N} + \frac{\partial^2}{\partial \tilde{x}^2} \tilde{N} + \alpha \tilde{N} (1-\tilde{N}) -\tilde{N} \tilde{P}, \\ \frac{\partial}{\partial \tilde{t}} \tilde{P} & = & -\delta v \frac{\partial}{\partial \tilde{x}} \tilde{P} + \varepsilon \frac{\partial^2}{\partial \tilde{x}^2} \tilde{P} + \tilde{N} \tilde{P} +\beta \tilde{P} (\kappa -\tilde{P}) , \end{array} $$

where the remaining quantities are defined as in the specialist predators model. Dropping the tildes for notational convenience gives the dimensionless model Eqs. 1–3 with Eq. 11.

Appendix B: Derivation of traveling wave speeds

Our first step is to consider traveling wave solutions to system Eqs. 1–2 without the unidirectional flow, i.e.,

$$ \frac{\partial}{\partial t} N(x,t) = \frac{\partial^2}{\partial x^2} N(x,t) + f(N, P) N ~, \label{eq:N-diff} $$

(17)

$$ \frac{\partial}{\partial t} P(x,t) = \varepsilon \frac{\partial^2}{\partial x^2} P(x,t) + g(N, P) P ~. \label{eq:P-diff} $$

(18)

In a second step, we will look at the full system Eqs. 1–2.

Traveling waves are translationally invariant solutions of the form N(z) = N(x,t) and P(z) = P(x,t) with z = x − c t. They have a fixed profile and move with constant speed c. Corresponding boundary conditions are

$$ \begin{array}{llll} N(-\infty) & = N_l ~, & \qquad N(+\infty) &= N_r , \\[12pt] P(-\infty) & = P_l ~, & \qquad P(+\infty) &= P_r . \end{array} $$

Substituting

$$ \begin{array}{llll} \dfrac{\partial}{\partial t} N(x,t)& = -c N' ~, & \qquad \dfrac{\partial^2}{\partial x^2} N(x,t) &= N'' , \\[12pt] \dfrac{\partial}{\partial t} P(x,t)& = -c P' ~, & \qquad \dfrac{\partial^2}{\partial x^2} P(x,t) &= P'' , \end{array} $$

where the primes denote differentiation with respect to z, the partial differential Eqs. 17–18 can be transformed to the following system of ordinary differential equations:

$$ \begin{array}{lll} -c \, N' & = & N'' + f(N,P) N , \\ -c \, P' & = & \varepsilon P'' + g(N,P) P . \end{array} $$

Introducing the new variables O = N′ and Q = P′, we arrive at a system of four differential equations of first order:

$$ N' = O ~, \label{eq:ode-N} $$

(19)

$$ O' = -c \: O -f(N,P) N ~, \label{eq:ode-O} $$

(20)

$$ P' = Q ~, \label{eq:ode-P} $$

(21)

$$ \varepsilon Q' = -c \: Q -g(N,P) P ~. \label{eq:ode-Q} $$

(22)

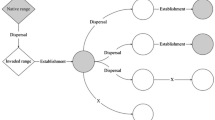
Recall that we are interested in two different scenarios (cf. Fig. 1). First, the prey spread into uninhabited space. We can specify the following boundary conditions. For z → + ∞, the prey still need to invade, i.e., N _r  = 0. For z → − ∞, the prey have already grown to carrying capacity, i.e., N _l  = 1. Moreover, we can assume that the predators are absent, P ≡ 0. Then, system Eqs. 19–22 reduces to

$$ N' = O ~, \label{eq:ode-N-only} $$

(23)

$$ O' = -c \: O -f(N,0) N ~. \label{eq:ode-O-only} $$

(24)

If f(N,0) is of logistic type as in Eq. 3, system Eqs. 23–24 corresponds to the Fisher equation (Fisher 1937; Kolmogorov et al. 1937). The minimum wave speed for which traveling wave solutions exist is the one given in Eq. 8. For Fisher’s equation, the minimum wave speed corresponds to the spread rate with which a locally introduced population will spread outwards (Aronson and Weinberger 1975).

Second, we are interested in the spread of predators. They propagate into an area where the prey have grown to carrying capacity. Ahead of the wave front, i.e., for z → + ∞, we have N = 1 and P = 0. Behind the wave front, i.e., for z → − ∞, predators and prey approach their coexistence state (N ^*,P ^*), cf. Fig. 1. Dunbar (1983, 1984) has proven the existence of such traveling waves and shown that their minimum wave speed is the one given in Eq. 10. This can also be heuristically derived by approximating N ≈ 1 and P ≈ 0 at the wave fronts (cf. Shigesada and Kawasaki 1997). System Eqs. 19–22 then reduces to

$$ P' = Q ~, \label{eq:ode-P-only} $$

(25)

$$ \varepsilon Q' = -c \: Q -g(1,P) P ~, \label{eq:ode-Q-only} $$

(26)

with $P_l=P^*$ and P _r  = 0. If g(1,P) is of the type as in Eq. 4, system Eqs. 25–26 corresponds to the Luther/Skellam model (Luther 1906; Skellam 1951). If g(1,P) is of the type as in Eq. 11, system Eqs. 25–26 corresponds to the Fisher model. In either case, the minimum wave speed is given by Eq. 10.

The wave speeds derived here, i.e., Eqs. 8 and 10, are referred to as reaction–diffusion speeds in the main text. Finally, we return to the initial Eqs. 1–2 with advective flow. The advection term is equivalent to using a moving reference frame as in Eqs. 17–18. That is, changing (x,t) to x − v t or x − δv t transforms Eqs. 1–2 to the same form as in Eqs. 17–18, cf. Lewis et al. (2009). We just need to consider two types of waves for both prey and predators, depending on whether they spread downstream or upstream. Their respective speeds are given by Eqs. 5 and 6 in the main text.