Two-parameter homogenization for a nonlinear periodic Robin problem for the Poisson equation: a functional analytic approach

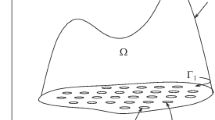
Abstract

We consider a nonlinear Robin problem for the Poisson equation in an unbounded periodically perforated domain. The domain has a periodic structure, and the size of each cell is determined by a positive parameter $\delta$. The relative size of each periodic perforation is instead determined by a positive parameter $\epsilon$. We prove the existence of a family of solutions which depends on $\epsilon$ and $\delta$ and we analyze the behavior of such a family as $(\epsilon ,\delta )$ tends to (0, 0) by an approach which is alternative to that of asymptotic expansions and of classical homogenization theory.

A Appendix

We first introduce the following variant of a result of Preciso [47, Prop. 1.1, p. 101].

Proposition A.1

Let $n_{1}$, $n_{2}\in {\mathbb {N}}{\setminus }\{0\}$, $\rho \in ]0,+\infty [$, $m\in {\mathbb {N}}$, $\alpha \in ]0,1]$. Let ${\varOmega }_{1}$ be a bounded open subset of ${\mathbb {R}}^{n_{1}}$. Let ${\varOmega }_{2}$ be a bounded open connected subset of ${\mathbb {R}}^{n_{2}}$ of class $C^1$. Then the composition operator T from $C^{0}_{\omega ,\rho }({\mathrm {cl}}{\varOmega }_{1})\times C^{m,\alpha }( {\mathrm {cl}}{\varOmega }_{2},{\varOmega }_{1})$ to $C^{m,\alpha }({\mathrm {cl}}{\varOmega }_{2})$ defined by

$$\begin{aligned} T[u, v]\equiv u\circ v \quad \forall (u,v)\in C^{0}_{\omega ,\rho }({\mathrm {cl}}{\varOmega }_{1})\times C^{m,\alpha }( {\mathrm {cl}}{\varOmega }_{2},{\varOmega }_{1}), \end{aligned}$$

is real analytic.

Then we introduce the following statement of [44, Lem. 3.8, Prop. 3.14, Rmk. 3.15].

Theorem A.2

Let $m \in {\mathbb {N}}{\setminus }\{0\}$, $\alpha \in ]0,1[$. Let $p\in Q$. Let ${\varOmega }$ be as in (1.1). Let $\epsilon _{0}$ be as in (1.2). Let $\tilde{g}\in C^{m,\alpha }(\partial {\varOmega })$. Then there exist $\epsilon _{1}\in ]0,\epsilon _{0}[$ and an open neighborhood $\tilde{{\varGamma }}$ of $\tilde{g}$ in $C^{m,\alpha }(\partial {\varOmega })$ and a real analytic map $(\hat{\eta }[\cdot ,\cdot ], \hat{\xi }[\cdot ,\cdot ])$ from $]-\epsilon _{1},\epsilon _{1}[\times \tilde{{\varGamma }}$ to $C^{m,\alpha }(\partial {\varOmega })_{0}\times {\mathbb {R}}$ such that the only solution $\varsigma [\epsilon ,g]\in C^{m,\alpha }_{q}({\mathrm {cl}}{\mathbb {S}}[{\varOmega }_{p,\epsilon }]^{-})$ of the Dirichlet problem

$$\begin{aligned} \left\{ \begin{array}{ll} {\varDelta }u (x)=0 &{} \forall x\in {\mathbb {S}}(\epsilon ,1 )^{-}, \\ u\ {\mathrm {is}}\ q-{\mathrm {periodic\ in }}\ {\mathbb {S}}(\epsilon ,1 )^{-}, \\ u(p+t\epsilon )= g(t) &{} \forall t\in \partial {\varOmega }, \end{array} \right. \end{aligned}$$

is delivered by the formula

$$\begin{aligned} \varsigma [\epsilon ,g](x)=w^{-}_{q}[\partial {\varOmega }_{p,\epsilon }, \hat{\eta }[\epsilon ,g] (\epsilon ^{-1}(\cdot - p))](x)+\hat{\xi }[\epsilon ,g]\qquad \forall x\in {\mathrm {cl}} {\mathbb {S}}(\epsilon ,1 )^{-}, \end{aligned}$$

for all $(\epsilon ,g)\in ]0,\epsilon _{1}[\times \tilde{{\varGamma }}$. Moreover,

$$\begin{aligned} (\hat{\eta }[0,\tilde{g}], \hat{\xi }[0,\tilde{g}])=(\tilde{\eta },\tilde{\xi }), \end{aligned}$$

where $(\tilde{\eta },\tilde{\xi })\in C^{m,\alpha }(\partial {\varOmega })_{0}\times {\mathbb {R}}$ is the only solution of the equation

$$\begin{aligned} -\frac{1}{2}\tilde{\eta } + w[\partial {\varOmega },\tilde{\eta }]+\tilde{\xi }=\tilde{g}\qquad {\mathrm {on}}\ \partial {\varOmega }\,. \end{aligned}$$

Also,

$$\begin{aligned} \tilde{\xi }=\int _{\partial {\varOmega }}\tilde{g}\tilde{\tau }\,d\sigma , \end{aligned}$$

where $\tilde{\tau }\in C^{m-1,\alpha }(\partial {\varOmega })$ is the only solution of the problem

$$\begin{aligned} -\frac{1}{2}\tau +w_{*}[\partial {\varOmega },\tau ]=0\qquad {\mathrm {on}}\ \partial {\varOmega },\qquad \int _{\partial {\varOmega }}\tau \,d\sigma =1\,. \end{aligned}$$

(A.1)

In order to compute $\tilde{\xi }$, the following lemma is sometimes useful.

Lemma A.3

Let the same assumptions of Theorem A.2 hold. Then

$$\begin{aligned} \lim _{]0,\epsilon _{1}[\times \tilde{{\varGamma }}\ni (\epsilon ,g)\rightarrow (0,\tilde{g})}\varsigma [\epsilon ,g](x)=\tilde{\xi }\qquad \forall x\in {\mathbb {R}}^{n}{\setminus } (p+q{\mathbb {Z}}^{n})\,. \end{aligned}$$

Proof

Since

$$\begin{aligned} \varsigma [\epsilon ,g](x)= & {} -\epsilon ^{n-1}\int _{\partial {\varOmega }}\nu _{{\varOmega }}(s)DS_{q,n}(x-p-\epsilon s)\hat{\eta }[\epsilon ,g](s)\,d\sigma _{s}+\hat{\xi }[\epsilon ,g]\\&\forall x\in {\mathrm {cl}}{\mathbb {S}}[{\varOmega }_{p,\epsilon }]^{-}, \end{aligned}$$

for all $(\epsilon ,\gamma ) \in ]0,\epsilon _{1}[\times \tilde{{\varGamma }}$, the statement follows by the continuity of $\hat{\eta }$ and $\hat{\xi }$ at $(0,\tilde{g})$, and by the continuity of $DS_{q,n}$ in ${\mathbb {R}}^{n}{\setminus } (p+q{\mathbb {Z}}^{n})$. $\square$

Then we deduce the validity of the following corollary.

Corollary A.4

Let the same assumptions of Theorem A.2 hold. Then there exist $\epsilon _{1}\in ]0,\epsilon _{0}[$, and an open neighborhood $\tilde{{\varGamma }}$ of $\tilde{g}$ in $C^{m,\alpha }(\partial {\varOmega })$, and an analytic map $J_{1}$ from $]-\epsilon _{1},\epsilon _{1}[\times \tilde{{\varGamma }}$ to ${\mathbb {R}}$ such that

$$\begin{aligned} \int _{Q{\setminus }{\varOmega }_{p,\epsilon }}\varsigma [\epsilon ,g]\,dx=J_{1}[\epsilon ,g]\qquad \forall (\epsilon ,g)\in ]0,\epsilon _{1}[\times \tilde{{\varGamma }}\,. \end{aligned}$$

Moreover, $J_{1}[0,\tilde{g}]=m_{n}(Q)\int _{\partial {\varOmega }}\tilde{g}\tilde{\tau }\,d\sigma$, where $\tilde{\tau }$ is the only solution in $C^{m-1,\alpha }(\partial {\varOmega })$ of problem (A.1).

Proof

We first observe that

$$\begin{aligned} \int _{Q{\setminus }{\mathrm {cl}}{\varOmega }_{p,\epsilon }}\varsigma [\epsilon ,g]\,d\sigma= & {} \int _{Q{\setminus }{\mathrm {cl}}{\varOmega }_{p,\epsilon }} w_{q}^{-}[\partial {\varOmega }_{p,\epsilon }, \hat{\eta }[\epsilon ,g](\epsilon ^{-1}(\cdot -p))](x)\,dx\nonumber \\&+\,\hat{\xi }[\epsilon ,g]m_{n}(Q{\setminus } {\varOmega }_{p,\epsilon }) \end{aligned}$$

(A.2)

for all $(\epsilon ,g)\in ]0,\epsilon _{1}[\times \tilde{{\varGamma }}$. Next we note that

$$\begin{aligned}&\int _{Q{\setminus }{\mathrm {cl}}{\varOmega }_{p,\epsilon }}w_{q}^{-}[\partial {\varOmega }_{p,\epsilon },\hat{\eta }[\epsilon ,g](\epsilon ^{-1}(\cdot -p))](x)\,dx\nonumber \\&\quad =-\int _{Q{\setminus }{\mathrm {cl}}{\varOmega }_{p,\epsilon }} \int _{\partial {\varOmega }_{p,\epsilon }}\nu _{ {\varOmega }_{p,\epsilon } }(y)DS_{q,n}(x-y) \hat{\eta }[\epsilon ,g](\epsilon ^{-1}(y-p))\,d\sigma _{y}\,dx \nonumber \\&\quad =-\int _{Q{\setminus }{\mathrm {cl}}{\varOmega }_{p,\epsilon }} \sum _{j=1}^{n}\frac{\partial }{\partial x_{j}}\int _{\partial {\varOmega }_{p,\epsilon }} S_{q,n}(x-y)\hat{\eta }[\epsilon ,g](\epsilon ^{-1}(y-p))(\nu _{{\varOmega }_{p,\epsilon }}(y))_{j}\,d\sigma _{y}\,dx\nonumber \\&\quad =\int _{\partial {\varOmega }_{p,\epsilon }}\sum _{j=1}^{n} (\nu _{{\varOmega }_{p,\epsilon }}(x))_{j} \int _{\partial {\varOmega }_{p,\epsilon }} S_{q,n}(x-y)\hat{\eta }[\epsilon ,g](\epsilon ^{-1}(y-p))(\nu _{{\varOmega }_{p,\epsilon }}(y))_{j}\,d\sigma _{y}\,d\sigma _{x} \nonumber \\&\quad =\sum _{j=1}^{n} \int _{\partial {\varOmega }}(\nu _{{\varOmega }}(t))_{j}\int _{\partial {\varOmega }}S_{q,n}(\epsilon (t-s))\hat{\eta }[\epsilon ,g](s)(\nu _{{\varOmega }}(s))_{j} \,d\sigma _{s}d\sigma _{t}\epsilon ^{2n-2} \nonumber \\&\quad =\sum _{j=1}^{n} \int _{\partial {\varOmega }}(\nu _{{\varOmega }}(t))_{j}\int _{\partial {\varOmega }}S_{ n} (t-s)\hat{\eta }[\epsilon ,g](s)(\nu _{{\varOmega }}(s))_{j} \,d\sigma _{s}d\sigma _{t}\epsilon ^{n} \nonumber \\&\qquad +\,\frac{\delta _{2,n}}{2\pi }\epsilon (\epsilon \log \epsilon ) \sum _{j=1}^{n} \int _{\partial {\varOmega }}(\nu _{{\varOmega }}(t))_{j}\,d\sigma _{t} \int _{\partial {\varOmega }}\hat{\eta }[\epsilon ,g](s)(\nu _{{\varOmega }}(s))_{j} \,d\sigma _{s} \nonumber \\&\qquad +\,\sum _{j=1}^{n} \int _{\partial {\varOmega }}(\nu _{{\varOmega }}(t))_{j}\int _{\partial {\varOmega }}R_{q, n}(\epsilon (t-s))\hat{\eta }[\epsilon ,g](s)(\nu _{{\varOmega }}(s))_{j} \,d\sigma _{s}d\sigma _{t}\epsilon ^{2n-2} \nonumber \\&\quad =\sum _{j=1}^{n} \int _{\partial {\varOmega }}(\nu _{{\varOmega }}(t))_{j}\int _{\partial {\varOmega }}S_{ n} (t-s)\hat{\eta }[\epsilon ,g](s)(\nu _{{\varOmega }}(s))_{j} \,d\sigma _{s}d\sigma _{t}\epsilon ^{n} \nonumber \\&\qquad +\,\sum _{j=1}^{n} \int _{\partial {\varOmega }}(\nu _{{\varOmega }}(t))_{j}\int _{\partial {\varOmega }}R_{q, n}(\epsilon (t-s))\hat{\eta }[\epsilon ,g](s)(\nu _{{\varOmega }}(s))_{j} \,d\sigma _{s}d\sigma _{t}\epsilon ^{2n-2}, \end{aligned}$$

(A.3)

for all $(\epsilon ,g)\in ]0,\epsilon _{1}[\times \tilde{{\varGamma }}$. Thus it is natural to define $J_{1}$ as the map from $]-\epsilon _{1},\epsilon _{1}[\times \tilde{{\varGamma }}$ to ${\mathbb {R}}$ which takes $(\epsilon ,g)$ to the sum of the right hand side of (A.3) and of the term $\hat{\xi }[\epsilon ,g]m_{n}(Q{\setminus } {\varOmega }_{p,\epsilon }) =\hat{\xi }[\epsilon ,g](m_{n}(Q)-\epsilon ^{n}m_{n}({\varOmega }))$ in the right hand side of equality (A.2). By classical potential theory, the operator $v[\partial {\varOmega },\cdot ]_{|\partial {\varOmega }}$ is linear and continuous from $C^{m-1,\alpha }(\partial {\varOmega })$ to $C^{m,\alpha }(\partial {\varOmega })$. Then the continuity of the pointwise product in $C^{m-1,\alpha }(\partial {\varOmega })$ and the analyticity of $\hat{\eta }[\cdot ,\cdot ]$ imply the analyticity of the first sum in the right hand side of (A.3). Then the analyticity of the map in (6.9), and the continuity of the product in $C^{m-1,\alpha }(\partial {\varOmega })$ and the analyticity of $\hat{\eta }[\cdot ,\cdot ]$ imply the analyticity of the second sum in the right hand side of (A.3) in the variable $(\epsilon ,g)$. The analyticity of $\hat{\xi }[\cdot ,\cdot ]$ implies the analyticity of the term $\hat{\xi }[\epsilon ,g](m_{n}(Q)-\epsilon ^{n}m_{n}({\varOmega }))$ upon the variable $(\epsilon ,g)$. Hence, $J_{1}[\cdot ,\cdot ]$ is real analytic from $]-\epsilon _{1},\epsilon _{1}[\times \tilde{{\varGamma }}$ to ${\mathbb {R}}$. Finally,

$$\begin{aligned} J_{1}[0,\tilde{g}]=m_{n}(Q)\hat{\xi }[0,\tilde{g}]=m_{n}(Q)\tilde{\xi } =m_{n}(Q)\int _{\partial {\varOmega }}\tilde{\tau }\tilde{g}\,d\sigma , \end{aligned}$$

where $\tilde{\tau }$ is the unique solution of problem (A.1). $\square$

Next we introduce the following technical statement.

Proposition A.5

Let $m \in {\mathbb {N}}{\setminus }\{0\}$, $\alpha \in ]0,1[$. Let $p\in Q$. Let ${\varOmega }$ be as in (1.1). Let $\epsilon _{0}$ be as in (1.2).

• (i) Let $\rho \in ]0,+\infty [$. Then there exists a real analytic map G from $]-\epsilon _{0},\epsilon _{0}[\times C^{0}_{\omega ,\rho }({\mathrm {cl}}Q)$ to ${\mathbb {R}}$ such that

  $$\begin{aligned} \int _{Q{\setminus }{\varOmega }_{p,\epsilon } }h\,dx=G[\epsilon ,h] \qquad \forall (\epsilon ,h)\in ]0,\epsilon _{0}[\times C^{0}_{\omega ,\rho }({\mathrm {cl}}Q),\\ G[0,h]=\int _{Q }h\,dx \qquad \forall h\in C^{0}_{\omega ,\rho }({\mathrm {cl}}Q) \,. \end{aligned}$$

• (ii) There exists a real analytic function $G_{1}$ from $]-\epsilon _{0},\epsilon _{0}[$ to ${\mathbb {R}}$ such that

  $$\begin{aligned} \int _{Q{\setminus }{\varOmega }_{p,\epsilon }}S_{q,n}(x-p)\,dx= G_{1}(\epsilon )-\delta _{2,n}\frac{\epsilon ^{2}\log \epsilon }{2\pi }m_{n}({\varOmega })\qquad \forall \epsilon \in ]0,\epsilon _{0}[\,. \end{aligned}$$

  Moreover,

  $$\begin{aligned} G_{1}(0)=\int _{Q}S_{q,n}(x-p)\,dx\,. \end{aligned}$$

Proof

For the existence of G, we follow the proof of Lemma 2.2 of [26] and we note that $\int _{Q{\setminus }{\varOmega }_{p,\epsilon }}h\,dx=\int _{Q}h\,dx-\epsilon ^{n}\int _{{\varOmega }}h(p+\epsilon s)\,ds$ for all $(\epsilon ,h)\in ]0,\epsilon _{1}[\times C^{0}_{\omega ,\rho }({\mathrm {cl}}Q)$, and we define G as the map from $]-\epsilon _{0},\epsilon _{0}[\times C^{0}_{\omega ,\rho }({\mathrm {cl}}Q)$ to ${\mathbb {R}}$ which takes $(\epsilon ,h)$ to the right hand side of such an equality. The analyticity of G follows by Proposition A.1. The formula for G[0, h] follows by the definition of G. Next we turn to prove statement (ii). By identity (2.3) and by the rule of change of variables, we have

$$\begin{aligned}&\int _{Q{\setminus }{\varOmega }_{p,\epsilon }}S_{q,n}(x-p)\,dx= \int _{Q}S_{q,n}(x-p)\,dx\\&\qquad -\,\epsilon ^{2}\int _{{\varOmega }}S_{n}(t)\,dt -\delta _{2,n}\frac{\epsilon ^{2}\log \epsilon }{2\pi }m_{n}({\varOmega }) -\epsilon ^{n}\int _{{\varOmega }}R_{q,n}(\epsilon t)\,dt \qquad \forall \epsilon \in ]0,\epsilon _{0}[\,. \end{aligned}$$

Then we can set

$$\begin{aligned} G_{1}(\epsilon )\equiv \int _{Q}S_{q,n}(x-p)\,dx-\epsilon ^{2}\int _{{\varOmega }}S_{n}(t)\,dt -\epsilon ^{n}\int _{{\varOmega }}R_{q,n}(\epsilon t)\,dt \qquad \forall \epsilon \in ]-\epsilon _{0},\epsilon _{0}[\,. \end{aligned}$$

By the analyticity of $R_{q,n}$ in $({\mathbb {R}}^{n}{\setminus } q{\mathbb {Z}}^{n})\cup \{0\}$ and by analyticity results on the composition operator (cf. Böhme and Tomi [3, p. 10], Henry [22, p. 29], Valent [48, Thm. 5.2, p. 44]), we deduce that the map from $]-\epsilon _{0},\epsilon _{0}[$ to $C^{m,\alpha }({\mathrm {cl}}{\varOmega })$, which takes $\epsilon$ to the function $R_{q,n}(\epsilon t)$ of the variable $t\in {\mathrm {cl}}{\varOmega }$ is real analytic. Then by the continuity of the linear operator from $C^{m,\alpha }({\mathrm {cl}}{\varOmega })$ to ${\mathbb {R}}$ which takes a map to its integral, the function $G_{1}$ is analytic from $]-\epsilon _{0},\epsilon _{0}[$ to ${\mathbb {R}}$. Then we obviously have $G_{1}(0)=\int _{Q}S_{q,n}(x-p)\,dx$.

$\square$

Next we introduce the following inequality for dilated q-periodic functions, which we prove by arguments akin to those of Braides and De Franceschi [6, ex. 27, p. 20]. We denote by $u_{\delta }$ the function from ${\mathbb {R}}^{n}$ to ${\mathbb {C}}$ defined by

$$\begin{aligned} u_{\delta }(x)\equiv u(x/\delta )\qquad \forall x \in {\mathbb {R}}^{n}, \end{aligned}$$

(A.4)

for all $\delta \in ]0,+\infty [$ and for all q-periodic functions $u\in L^{1}_{{\mathrm {loc}}}({\mathbb {R}}^{n})$. Then we have the following.

Lemma A.6

Let $r\in [1,+\infty [$, $\delta _{0}\in ]0,+\infty [$. Let V be a bounded open subset of ${\mathbb {R}}^{n}$. Then there exists $C\in ]0,+\infty [$ such that

$$\begin{aligned} \Vert u_{\delta }\Vert _{L^{r}(V)}\le C \Vert u \Vert _{L^{r}(Q)}\qquad \forall \delta \in ]0,\delta _{0}[, \end{aligned}$$

for all q-periodic $u\in L^{1}_{{\mathrm {loc}}}({\mathbb {R}}^{n})$.

Proof

Since V is bounded, there exists a family $\{z_{l}\}_{l=1}^{s}$ of points of ${\mathbb {Z}}^{n}$ such that

$$\begin{aligned} V\subseteq \bigcup _{l=1}^{s} (qz_{l}+{\mathrm {cl}}Q) \,. \end{aligned}$$

Then the q-periodicity of u implies that

$$\begin{aligned} \int _{V}|u_{\delta }(y)|^{r}\,dy\le & {} \sum _{l=1}^{s}\int _{ qz_{l}+{\mathrm {cl}}Q }|u_{\delta }(y)|^{r}\,dy =\sum _{l=1}^{s}\int _{\delta ^{-1}qz_{l}+\delta ^{-1}{\mathrm {cl}}Q }|u (x)|^{r}\,dx\delta ^{n} \\\le & {} \sum _{l=1}^{s}\int _{\delta ^{-1}qz_{l}+([\delta ^{-1}]+1){\mathrm {cl}}Q }|u (x)|^{r}\,dx\delta ^{n} =s\int _{ ([\delta ^{-1}]+1){\mathrm {cl}}Q }|u (x)|^{r}\,dx\delta ^{n} \\\le & {} C^{r}\int _{ Q }|u(x)|^{r}\,dx \qquad \forall \delta \in ]0,\delta _{0}[, \end{aligned}$$

for all q-periodic $u\in L^{1}_{{\mathrm {loc}}}({\mathbb {R}}^{n})$, where

$$\begin{aligned} C\equiv s^{1/r}\left\{ \sup _{ \delta \in ]0,\delta _{0}[ }([\delta ^{-1}]+1)^{n}\delta ^{n} \right\} ^{1/r}<+\infty , \end{aligned}$$

and where $[\delta ^{-1}]$ denotes the integer part of $\delta ^{-1}$. $\square$

Next we introduce the following lemma for dilated q-periodic functions.

Lemma A.7

Let $u\in L^{1}_{{\mathrm {loc}}}({\mathbb {R}}^{n})$ be a q-periodic function. Let $\tilde{y}\in {\mathbb {R}}^{n}$, $s\in ]0,+\infty [$, $l\in {\mathbb {N}}{\setminus }\{0\}$. Then the following equality holds

$$\begin{aligned} \int _{{\mathbb {R}}^{n}}u_{s/l}(x)\chi _{\tilde{y}+sQ}(x)\,dx=s^{n}\int _{Q}u\,dx, \end{aligned}$$

[see (A.4)].

Proof

Since $u_{s/l}$ is $l^{-1}sq$-periodic, it is also sq-periodic and accordingly,

$$\begin{aligned} \int _{{\mathbb {R}}^{n}}u_{s/l}(x)\chi _{\tilde{y}+sQ}(x)\,dx =\int _{\tilde{y}+sQ}u_{s/l}(x)\,dx=\int _{sQ}u_{s/l}(x)\,dx\,. \end{aligned}$$

Next we observe that

$$\begin{aligned} \bigcup _{ 0\le z_{j}\le l -1 }(qz+l^{-1} Q) \subseteq Q,\qquad m_{n}\left( Q{\setminus }\bigcup _{0\le z_{j}\le l-1 }(qz+l^{-1} Q) \right) =0\,. \end{aligned}$$

Accordingly, the $l^{-1}s q$-periodicity of $u_{s/l}(\cdot )$ implies that

$$\begin{aligned} \int _{sQ}u_{s/l}(x)\,dx= & {} \int _{sl^{-1}Q}u_{s/l}(x)\,dxl^{n}\\= & {} \int _{sl^{-1}Q}u( x/(s/l))\,dxl^{n}=\int _{Q}u(y)\,dyl^{n}(s/l)^{n}= s^{n}\int _{Q}u\,dx\,. \end{aligned}$$

$\square$

Finally, we introduce the following elementary lemma of [33, Lem. A.5].

Lemma A.8

Let $m\in {\mathbb {N}}{\setminus }\{0\}$, $\alpha \in ]0,1[$. Let $p\in Q$. Let ${\varOmega }$ be as in (1.1). Let $\epsilon _{0}\in ]0,+\infty [$ be as in (1.2). Let $\epsilon _{1}\in ]0,\epsilon _{0}[$.

• (i) Let $\tilde{{\varOmega }}$ be an open subset of ${\mathbb {R}}^{n}$ with a nonzero distance from $p+q{\mathbb {Z}}^{n}$. Then there exist $\epsilon _{ \tilde{{\varOmega }} }^{*}\in ]0, \epsilon _{1}[$ such that

  $$\begin{aligned} {\mathrm {cl}} \tilde{{\varOmega }} \subseteq {\mathbb {S}}[{\varOmega }_{p,\epsilon }]^{-} \qquad \forall \epsilon \in [-\epsilon _{ \tilde{{\varOmega }} }^{*},\epsilon _{ \tilde{{\varOmega }} }^{*}] , \end{aligned}$$

  and $\epsilon _{ \tilde{{\varOmega }} }\in ]0,\epsilon _{ \tilde{{\varOmega }} }^{*}[$ such that

  $$\begin{aligned} {\mathrm {cl}}{\mathbb {S}}[{\varOmega }_{p,\epsilon _{ \tilde{{\varOmega }} }^{*}}]^{-} \subseteq {\mathbb {S}}[{\varOmega }_{p,\epsilon }]^{-} \qquad \forall \epsilon \in [-\epsilon _{ \tilde{{\varOmega }} } ,\epsilon _{ \tilde{{\varOmega }} } ] \,. \end{aligned}$$

• (ii) Let ${\varOmega }^{\sharp }$ be a bounded open subset of ${\mathbb {R}}^{n}$ such that ${\varOmega }^{\sharp }\subseteq {\mathbb {R}}^{n}{\setminus } {\mathrm {cl}}{\varOmega }$. Then there exists $\epsilon _{ {\varOmega }^{\sharp },r }\in ]0,\epsilon _{1}[$ such that

  $$\begin{aligned} p+\epsilon {\mathrm {cl}}{\varOmega }^{\sharp } \subseteq Q, \qquad p+\epsilon {\varOmega }^{\sharp }\subseteq {\mathbb {S}}[{\varOmega }_{p,\epsilon }]^{-}\qquad \forall \epsilon \in [-\epsilon _{ {\varOmega }^{\sharp },r } ,\epsilon _{ {\varOmega }^{\sharp } ,r} ]{\setminus }\{0\}\,. \end{aligned}$$