Abstract
We introduce the uniform coprime hypergraph of integers \(\mathrm{CHI}_k\) which is the graph with vertex set \({\mathbb {Z}}\) and a  -hyperedge exactly between every \(k+1\) elements of \({\mathbb {Z}}\) having greatest common divisor equal to 1. This generalizes the concept of coprime graphs. We obtain some basic properties of these graphs and give upper and lower bounds for the clique number of certain subgraphs of \(\mathrm{CHI}_k\).
-hyperedge exactly between every \(k+1\) elements of \({\mathbb {Z}}\) having greatest common divisor equal to 1. This generalizes the concept of coprime graphs. We obtain some basic properties of these graphs and give upper and lower bounds for the clique number of certain subgraphs of \(\mathrm{CHI}_k\).
Similar content being viewed by others
Notes
Actually they proved a stronger version where l does not have to be larger than m, although in that case we would not have such a nice graph theoretic interpretation.
Every tree T on n vertices is isomorphic to a subgraph of
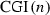 . This conjecture was proved in 2011 [10] for all sufficiently large n.
. This conjecture was proved in 2011 [10] for all sufficiently large n.
References
Ahlswede, R., Khachatrian, L.H.: On extremal sets without coprimes. Acta Arith. 66(1), 89–99 (1994)
Ahlswede, R., Khachatrian, L.H.: Maximal sets of numbers not containing \(k + 1\) pairwise coprime integers. Acta Arith. 72(1), 77–100 (1995)
Ahlswede, R., Khachatrian, L.H.: Sets of integers and quasi-integers with pairwise common divisor. Acta Arith. 74(2), 141–153 (1996)
Ahlswede, R., Khachatrian, L.H.: Sets of integers with pairwise common divisor and a factor from a specified set of primes. Acta Arith. 75(3), 259–276 (1996)
Bondy, J.A., Murty, U.S.R.: Graph Theory. Graduate Texts in Mathematics, vol. 244. Springer, New York (2008)
Dusart, P.: The \(k{\rm th}\) prime is greater than \(k(\ln k+\ln \ln k-1)\) for \(k\geqslant 2\). Math. Comp. 68(225), 411–415 (1999)
Erdős, P.: Remarks in number theory. IV. Extremal problems in number theory. I. Mat. Lapok 13, 228–255 (1962) (in Hungarian)
Erdős, P., Gallai, T.: Graphs with prescribed degrees of vertices. Mat. Lapok 11, 264–274 (1960) (in Hungarian)
Erdős, P., Sarkozy, G.N.: On cycles in the coprime graph of integers. Electron. J. Combin. 4(2), #R8 (1997)
Haxell, P., Pikhurko, O., Taraz, A.: Primality of trees. J. Comb. 2(4), 481–500 (2011)
Mutharasu, S., Mohamed Rilwan, N., Angel Jebitha, M.K., Tamizh Chelvam, T.: On generalized coprime graphs. Iran. J. Math. Sci. Inform. 9(2), 1–6 (2014)
Pomerance, C., Selfridge, J.L.: Proof of D.J. Newman’s coprime mapping conjecture. Mathematika 27(1), 69–83 (1980)
Robertson, L., Small, B.: On Newman’s conjecture and prime trees. Integers 9(2), 117–128 (2009)
Sander, J.W., Sander, T.: On the kernel of the coprime graph of integers. Integers 9(5), 569–579 (2009)
Sárközy, G.N.: Complete tripartite subgraphs in the coprime graph of integers. Discrete Math. 202(1–3), 227–238 (1999)
Tripathi, A., Vijay, S.: A note on a theorem of Erdős & Gallai. Discrete Math. 265(1–3), 417–420 (2003)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
de Wiljes, JH. Complete subgraphs of the coprime hypergraph of integers I: introduction and bounds. European Journal of Mathematics 3, 379–386 (2017). https://doi.org/10.1007/s40879-017-0137-5
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40879-017-0137-5




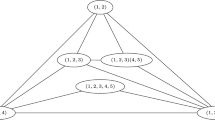
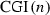 . This conjecture was proved in 2011 [
. This conjecture was proved in 2011 [