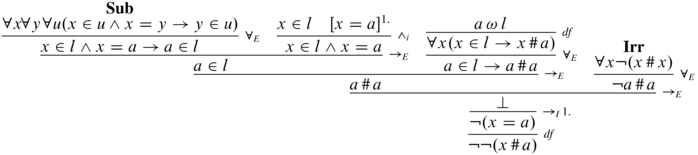Abstract
A comparison is made between logical notions of constructivity and the traditional Euclidean conception of geometry as a science of constructions. In particular, it is investigated whether the actions performable by an abstract human geometer can already be captured, at a more syntactic level, by some logical notions of constructivity. Three of these notions are analyzed: (i) intuitionistic admissibility; (ii) provability by finitistic, direct, or effective means; and (iii) algorithmic executability. Two results are then presented. On the one hand, it is shown how a plausible characterization of geometrical constructivity can be achieved by combining all three previous features. On the other hand, through a comparison with arithmetic, it is argued that geometry is essentially characterized by hypothetical and potential constructions: geometrical objects are not effectively constructed, but they are only constructible. This latter result is mainly achieved via the analysis of the existential witness extraction for \(\Pi _{2}\) sentences. This property, which is usually considered as emblematic of proof-theoretical and syntactic approaches to constructivity, represents in fact the vestige of a referentialist position which seems to be dispensable for the description of geometrical practice.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Notes
- 1.
A similar analogy is drawn in Beeson (2010, §1).
- 2.
The fact that we do not consider actions performed within a limited amount of resources should not be construed as deeming this aspect irrelevant for the discussion of the relation between geometry and logic. For further information about the connections between geometrical proofs and resource-sensitive logics, see Pambuccian (2004).
- 3.
Note that starting from the first French translation of the Grundlagen der Geometrie, in 1900, Hilbert adds the axiom of completeness in order to force the models of Euclidean geometry to be maximal, that is, isomorphic to the field of real numbers, thus obtaining categorical results (cf. Awodey and Reck 2002, §§3.2, 3.3). For further details, see Hallett (2008, §§8.2, 8.3) and Venturi (2011).
- 4.
The two ways of conceiving the geometrical space presented here reflect the two ways in which, according to Kreisel (1981), mathematical concepts are traditionally analyzed. Borrowing Pascal’s terminology, these two ways can be called the esprit de géométrie, “where we think of arbitrary points, not only those constructed by use of Euclid’s operations, a ruler and a pair of compasses” (1981, p. 70), and the esprit de finesse, where, as in algebra, the set of objects considered is any set which is closed under a given list of operations, and in particular the smallest of these sets (1981, p. 71; cf. the difference from what has been said in Note 3).
- 5.
For a survey of this kind of algorithmic study of Euclidean structures, see Pambuccian (2008).
- 6.
An axiomatic presentation of Euclidean geometry based instead on an intuitionistic framework can be found in Lombard and Vesley (1998). This system is to EG as Heyting arithmetic is to Peano arithmetic: when its axioms are interpreted classically, one gets a theory equivalent to classical Euclidean geometry.
- 7.
In the same vein, according to Mueller (1981, p. 14), “For Hilbert geometric axioms characterize an existent system of points, straight lines, etc. At no time in the Grundlagen is an object brought into existence, constructed. Rather its existence is inferred from the axioms.”
- 8.
It is worth noting that what we said is not in contrast with Tarski’s claim according to which his presentation of elementary geometry concerns “that part of Euclidean geometry which can be formulated and established without the help of any set theoretical notions” (Tarski 1959, p. 16). Actually, what Tarski wants to say here is simply that his formalization of geometry does not make any appeal to a higher-order language allowing quantification over sets (cf. Tarski 1959, p. 17, Szczerba, L.W. 1986, p. 908). But, evidently, this is a problem concerning the complexity of the language and it does not mean that there is no use at all of set-theoretical machinery as a background theory.
- 9.
- 10.
Note that the definition (8.1) cannot be obtained simply via the negation of equality. On the one hand, the negation of a universal sentence cannot be in general intuitionistically transformed into an existential sentence. On the other hand, not even making appeal to classical negation would be sufficient: although the definiens of (8.1) entails the classical negation of equality, i.e., the existence of \(k \in \mathbb{Z}^{+}\), such that \(\vert a_{k} - b_{k}\vert > 2k^{-1}\), the converse does not hold (at least using the definitions present in Bishop 1967, §2.2).
- 11.
It has to be mentioned that in Tarski (1959, p. 17), among the primitive relations of the language of EG, there appears a relation of “diversity.” Unfortunately, Tarski does not explain how this relation should be intended; thus we cannot say if it corresponds to the apartness relation or not. Due to this lack of specification, as well as the fact that none of Tarski’s collaborators paid any attention to this diversity relation, we have decided to present EG along the lines of Givant and Tarski (1999), where equality is a primitive relation and diversity is defined simply as its negation.
- 12.
This failure is not strictly due to some necessary epistemic limitations, as it could be the case that the two points are indeed the same: in this case an ideal precision would be required for the verification, and such capacity is not possessed by human beings. On the other hand, the failure could also be due to some contingent epistemic limitations. For instance, the computation could be so long that, after a certain amount of time, we become too tired to continue it and we thus decide to abandon it.
- 13.
With the adjective “free,” we want simply to point out that the subject generating a sequence can freely decide the degree of restrictions in the selection of each element of the sequence. At one extreme of the range each element is completely determined in advance by a law (see p. 154, infra), so that the choice of this element is temporally independent. On the other extreme, the choice is totally unrestricted, in the sense that there is no kind of a priori fixed rule guiding the generation of the sequence, so that each element is undetermined in advance but depends on the particular point in time at which the choice is made.
- 14.
The language used in Heyting’s presentation of intuitionistic geometry is thus a two-sorted first-order language. In this work, we will not adopt any particular notation to distinguish between the two sorts of variables for points and lines, respectively; it will be the presence of relations that disambiguates the reading of formulas. However, in general, we will use the letters x, y, z to indicate (eigen)variables and a, b, c to indicate parameters for points, while for lines, u, v will be used to indicate (eigen)variables and l, m the parameters.
- 15.
The use of the membership symbol is due to the intuitive idea that a line is nothing else but a set of points. As we have seen, this idea is literally followed by the Tarskian approach, while in Heyting it is simply a façon de parler; it is in fact contradicted by taking points and lines to be two distinct sets, irreducible one to the other. A much more interesting aspect is that, historically, Heyting (1959) did not consider the outsideness relation as a primitive relation, but as defined from incidence and apartness, namely, \(a\,\omega \,l \equiv _{df}\forall x(x \in l \rightarrow x\,\#\,a)\). However, as was noticed by von Plato (2010b), this definition is too weak from the intuitionistic point of view. If we understand it correctly, this means that when it is imposed to use the previous definition in conjunction with the axiom of substitution for points
$$\displaystyle{\forall x\forall y\forall u(x \in u \wedge x = y \rightarrow y \in u)\quad \mathbf{Sub}}$$then the formula x # a is no longer intuitionistically derivable from x ∈ l. Actually, what can be derived is ¬(x = a), which is equivalent to ¬¬(x # a), and, as we have seen, double negation elimination is not in general admissible in the intuitionistic theory of apartness (cf. pp. 134135, supra):
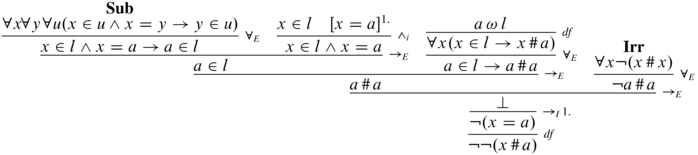
- 16.
The last step of the proof corresponds to the reductio ad absurdum. This means that when we reason about the properties of the derivability relation in EG, we are reasoning classically. However, this does not yet preclude the possibility of a BHK reading of disjunction in \(EG\). The reason is that some clauses of BHK semantics already induce forms of non-constructive meta-level reasoning (cf. von Plato 2013, p. 103). In particular, interpreting \(\perp \) as a proposition which is never realized, i.e., for which no proof can be exhibited, already entails classical forms of meta-level reasoning on the properties of the derivability relation in a purely intuitionistic logical setting. For instance, consider the proof that the \(0\)-ary rule \(L\perp \) of \(\mathbf{G3i}\) is valid, i.e., that \(\overline{\perp,\Gamma \vdash \Delta }\) is valid. By assuming that ⊥ is never realized, the antecedent is always false and therefore the sequent is vacuously valid. In other words, assuming that \(\perp \) is never realized induces proving the validity of the previous sequent in the same way as the validity of a material implication is classically proved.
- 17.
By the term “proof,” we here indicate a closed derivation, that is, a derivation having all the assumptions discharged. When we want to speak of derivations having non-discharged assumptions, we will call them open proofs (see p. 152, infra).
- 18.
Some clarifications have to be made at this point. Actually, in the presence of some kind of infinitary rule, it could be possible to conclude \(\vdash _{EG}\forall x\neg A(x)\) from the infinite number of premisses \(\neg A(t_{i})\), where every t i denotes an object of the intended model of EG. But the use of an infinitary rule would be in conflict with C2. However, the problem de facto does not hold because the language of EG does not contain any kind of individual constants or function operators; therefore, it is not possible to form any closed term in EG. The second case analyzed is thus only a purely theoretical exercise, since it would be excluded from the very nature of the language of EG.
- 19.
Although a different language is used, namely, the one introduced in Sect. 8.3.3, our presentation of the axiom of non-collinearity closely follows that of the lower-dimensional axiom adopted by Tarski in EG, i.e.,
$$\displaystyle{\exists x_{1}\exists x_{2}\exists x_{3}(\neg \beta (x_{1}x_{2}x_{3}) \wedge \neg \beta (x_{2}x_{3}x_{1}) \wedge \neg \beta (x_{2}x_{1}x_{2})).}$$It should be noted that sometimes this axiom is formulated by specifying that the three points are different, that is, by adding to the propositional matrix the conjunction \(\bigwedge _{1\leqslant i,j\leqslant 3}x_{i}\neq x_{j}\). However, this condition is not really necessary for our analysis. It is thus dropped in order to simplify the use of this axiom and NC as well.
- 20.
For simplicity, we limit ourselves here to the case in which only one variable is universally quantified and no type dependencies are involved. We will see later some more specific examples which need an appeal to dependent types.
- 21.
The decidability of the propositional matrix A is a direct consequence of the fact that A is a primitive recursive formula, that is, a formula constituted by relations which are themselves primitive recursive. And if a relation is primitive recursive, then its characteristic function is a primitive recursive function, that is, a function computable in a mechanical way.
- 22.
The expression “last rule lemma” (or “last rule property”) comes from the French word lemme de la dernière règle (or propriété de la dernière règle) that we have learned from G. Dowek’s lectures on proof theory, but this terminology is not very standard in the proof-theoretical literature. An alternative formulation could be “introduction form property,” as can be found in Schroeder-Heister (2014, §1.3). However, neither does this expression have a large diffusion. See below for the explanation of this property.
- 23.
Here we are working in a natural deduction setting. When natural deduction proofs are translated into a sequent calculus setting, then the status of detours becomes clearer: the use of detours corresponds to cuts, and cuts correspond to the use of lemmas. A proof without detours is thus a proof where every inferential passage has been made completely explicit.
- 24.
The claim that every theory, in order to be meaningful, has to satisfy this property is known under the name of Dummett’s fundamental assumption.
- 25.
It is worth noting that if the propositional matrix A of a \(\Pi _{2}\) sentence is not primitive recursive, the procedure we have just described cannot be carried out. Consider, for instance, the sentence \(\forall x\exists y((y = 0 \wedge B(x)) \vee (y = 1 \wedge \neg B(x)))\), where B is an undecidable predicate. It can be shown that this sentence could be derivable in PA, without being derivable in HA. In effect, if it was derivable in HA, we could instantiate the universal quantifier with a numeral n, eliminate all possible detours in the proof, and obtain then a witness t for the existential quantifier, so that
$$\displaystyle{\vdash _{HA^{R}}(t = 0 \wedge B(n)) \vee (t = 1 \wedge \neg B(n)).}$$Since this last sentence is a closed one, and the proof does not contain open assumptions, we could then apply Proposition 2 and obtain one of the two disjoints. But this is impossible, because it amounts to rendering B decidable.
- 26.
For the sake of the argument, we assume, without actually proving it, that the transformation of the classical theory into the intuitionistic one is sound at least with respect to \(\Pi _{2}\) sentences: if A is a \(\Pi _{2}\) sentence formulated in the language of the classical theory T and provable in T, then its intuitionistic translation \(\mathtt{I}(\mathit{A})\) is provable in I(T) as well. For further details about the relation between classical theories and their corresponding intuitionistic ones, see Negri and von Plato (2005).
- 27.
Using a terminology borrowed from Skolem, we could say that these rules are just principles of derivation (Erzeugungsprinzipien; cf. von Plato 2007, p. 199) representing specific kinds of mathematical reasoning.
- 28.
Note that here we are reasoning in a purely hypothetical way. Skolemizing inside an intuitionistic setting is far from being trivial, in particular, because the derivability relation could be affected (as is the case for intuitionistic logic with equality; cf. Mints 2000). However, reasoning as if this kind of Skolemization was not only possible, but even free from pernicious consequences, is not problematic for our argumentative goals: what we want to show is just that even if it were possible to accomplish this kind of Skolemization, we would still not be able to reach the desired result, namely, to exploit normalization for constructing geometrical objects.
- 29.
In fact, it could be objected that the witness can be obtained by executing the algorithm inscribed in the relation ω. This algorithm could give as a result a certain c i (\(1\leqslant i\leqslant 3\)) such that c i ω l, and thus, it would be possible to conclude \(\exists y(y\,\omega \,l)\) by a \(\exists _{I}\). The problem is that, taken as syntactic objects, individual constants do not possess any kind of structure; hence, the algorithm inscribed in ω cannot be used, since there is no data (structured) on which to operate. Once again it seems necessary to interpret constants on numerical values.
- 30.
More prosaically, the idea is that ln can be seen as a non-injective way to map points to lines, in the sense that there can be different ways to get the same line as a certain particular position on the plane.
- 31.
In Barendregt’s cube, which is a way of classifying theories in terms of their type dependencies, such a theory would be considered as belonging to the system \(\lambda \text{P}\underline{\omega }\). Cf. Hindley and Seldin (2008, pp. 194, 200).
- 32.
To be more precise, we should say that the model contemplated here is something like a realizability model.
- 33.
Roughly speaking, the idea is that the reduction steps for detours involving logical connectives are defined on open proofs and not closed ones (cf. Schroeder-Heister 2006, §§3.1, 3.2). Moreover, as was said on p. 145, no new detours are created by mathematical rules. It remains to analyze the permutations between logical and mathematical rules, but we will see this on p. 150. It should be noticed that putting open proofs on the same level as closed ones, at least with respect to their semantic role, is far from trivial. Usually, in proof-theoretical semantics, the priority is given to closed proofs because the semantic key concept is that of validity, rather than that of computation, as it is in the case under analysis (cf. Schroeder-Heister 2006, §3.3; Schroeder-Heister 2014, §§2.2.2, 2.2.3).
- 34.
In order to obtain this kind of situation, some non-trivial work might be required. The main problem is that the term appearing as a premiss of the \(\exists _{I}\) rules may not always be the same; in particular there could be a term t i for each application of \(\exists _{I}\). However, if a subterm property can be proved (cf. von Plato 2010a, §3), then each term t i would appear in Q i or in some other assumptions of the subtree concluding with A[t i ∕x]. Thus, by means of appropriate substitutions, it would be possible to identify all the t i . Note that this does not create captures of eigenvariables, because by hypothesis there are no more GR rules. In some special cases, it would be simply sufficient to add a sequence of equalities t i = t j in the assumptions.
- 35.
In fact, in this case, inputs and outputs are in the form of data structures, since they are given in a canonical normal form (see Martin-Löf 1984, p. 71), i.e., they are in the form
$$\displaystyle{\mathop{\underbrace{s(\ldots s}}\limits _{n\;\text{ times}}(0)\ldots ).}$$ - 36.
Notice that rational numbers can be reduced to natural numbers by the usual techniques, such as Cantor’s anti-diagonal argument. It follows that in order to speak of real numbers, we do not need to substantially expand the set of primitive signs we already used for arithmetic.
- 37.
We should indeed assume this finite text to be correct from a grammatical point of view; otherwise, it could not convey any kind of contentful instruction.
- 38.
For instance, in the case under analysis, the principles leading to the choice of a particular form of the continuum consist both in its epistemic accessibility and in its syntactic representability, while structural properties, as, for example, cardinality or compactness, are left aside.
- 39.
A similar comparison between geometry and arithmetic is drawn by Mumma (2012, §3). Roughly speaking, his idea is that while in arithmetic the arguments and values of constructions (functions) can always be put into canonical normal form (see note 35, supra), in geometry this is not the case, since this kind of data can vary continuously. Thus, while in arithmetic the study of the identity between constructions concerns also the identity between their respective values, in geometry such identities concern only the constructions, but not their objects (Mumma 2012, p. 117). Indeed, as noticed by Panza (2011, p. 48), in Euclidean geometry, questions about the identity of geometrical objects are ill-posed, since “there is no clear sense in which one could fix the reference of a singular term” for geometrical entities, like, for example, equilateral triangles, “in such a way that it be taken to refer to the same equilateral triangle in any one of its occurrences.”
- 40.
The word “postulate” comes indeed from the Latin verb postulare, which means to demand, to require. Note that the Greek word used by Euclid, i.e., αἴτεμα, has basically the same meaning.
- 41.
The idea is that this assumption plays a similar role to Wittgenstein’s ladder of §6.54 of the Tractatus. Assuming the geometrical space to be continuous is essential in order to ground our formal and logical reconstruction of Euclidean geometry on the analysis of the activity of an idealized human geometer, as described in Sect. 8.1.3. But once this reconstruction has been achieved, this assumption can finally be dropped, and the system obtained can be used as a non-interpreted formal system.
- 42.
A similar position is endorsed by von Plato (1995, p. 192), when he describes his system of constructive geometry as a system that “does not stipulate what the basic objects are, or how their basic relations are proved. In this sense it belongs to abstract mathematics, rather than to traditional constructive mathematics, where the aim has been to define once and for all the natural numbers and build all other mathematical structures upon them.”
References
Avigad, J., E. Dean, and J. Mumma. 2009. A formal system for Euclid’s Elements. Review of Symbolic Logic 2: 700–768.
Awodey, S., and E.H. Reck. 2002. Completeness and categoricity. Part I: nineteenth-century axiomatics to twentieth-century metalogic. History and Philosophy of Logic 23: 1–30.
Beeson, M. 2010. Constructive geometry. In Proceedings of the tenth Asian logic conference, ed. T. Arai et al., 19–84. Singapore: World Scientific.
Bernays, P. 1930. Die Philosophie der Mathematik und die Hilbertsche Beweistheorie. [The philosophy of mathematics and Hilbert’s proof theory] English trans. P. Mancosu. In Mancosu (1998), 234–265.
Bernays, P. 1967. David Hilbert. In Encyclopedia of philosophy, ed. P. Edwards, vol. 3, 496–505. New York: MacMillan.
Bishop, E. 1967. Foundations of constructive analysis. New York: McGraw-Hill.
Brouwer, L.E.J. 1924. Intuitionistische Zerlegung mathematischer Grundbgriffe. Jahresbericht der Deutsche Mathematiker-Vereinigung 33: 241–256.
Dowek, G. 2009. Principles of programming languages. Berlin: Springer.
Dummett, M. 1963. Realism. In Id., Truth and other enigmas, 145–165. London: Duckworth.
Dummett, M. 1977. Elements of intuitionism. Oxford: Clarendon Press.
Engeler, E. 1968. Remarks on the theory of geometrical constructions. In The syntax and semantics of infinitary languages, Lecture notes in mathematics, vol. 72, ed. J. Barwise, 64–76. Berlin: Springer.
Engeler, E. 1993. Foundations of mathematics: questions of analysis, geometry and algorithmics, English trans. C.B. Thomas. Berlin: Springer.
Feferman, S. 1964. Systems of predicative analysis. Journal of Symbolic Logic 29: 1–30.
Feferman, S. 2005. Predicativity. In The Oxford handbook of philosophy of mathematics and logic, ed. S. Shapiro, 590–624. Oxford: Oxford University Press.
Friedman, H. 1999. A consistency proof for elementary algebra and geometry. Manuscript.
Gandy, R. 1988. The confluence of ideas in 1936. In The universal turing machine: a half-century survey, ed. R. Herken, 51–102. Berlin: Springer.
Givant, S., and A. Tarski. 1999. Tarski’s system of geometry. The Bulletin of Symbolic Logic 5: 175–214.
Greenberg, M.J. 1993. Euclidean and non-Euclidean geometries: development and history, 3rd ed. New York: W.H. Freeman.
Hallett, M. 2008. Reflections on the purity of method in Hilbert’s Grundlagen der Geometrie. In The philosophy of mathematical practice, ed. P. Mancosu, 198–255. Oxford: Oxford University Press.
Heyting, A. 1925. Intuitionistische Axiomatik der Projectieve Meetkunde. Groningen: P. Noordhoff.
Heyting, A. 1959. Axioms for intuitionistic plane affine geometry. In L. Henkin et al. (1959), 160–173.
Heyting, A. 1971. Intuitionism: an introduction. Amsterdam: North Holland.
Henkin, L., P. Suppes, and A. Tarski (eds.). 1959. The axiomatic method, with special reference to geometry and physics. Amsterdam: North Holland.
Hindley, J.R. and J.P. Seldin 2008. Lambda-calculus and combinators: an introduction. Cambridge: Cambridge University Press.
Kolmogorov, A. 1932. Zur Deutung der intuitionistischen Logik. [On the interpretation of intuitionistic logic], English trans. P. Mancosu. In Mancosu (1998), 328–333.
Kreisel, G. 1981. Constructivist approaches to logic. In Modern logic, a survey: historical, philosophical, and mathematical aspects of modern logic and its applications, ed. E. Agazzi, 67–91. Dordrecht: Reidel.
Lombard, M., and R. Vesley 1998. A common axiom set for classical and intuitionistic plane geometry. Annals of Pure and Applied Logic 95, 229–255.
Mancosu, P. (ed.). 1998. From Brouwer to Hilbert: the debate on the foundations of mathematics in the 1920’s. Oxford: Oxford University Press.
Martin-Löf, P. 1970. Notes on constructive mathematics. Stockholm: Almqvist & Wiksell.
Martin-Löf, P. 1984. Intuitionistic type theory. Naples: Bibliopolis.
Mäenpää, P., and J. von Plato. 1993. The logic of Euclidean construction procedure. Acta Philosophica Fennica 49: 275–293.
Mints, G.E. 2000. Axiomatization of a Skolem function in intuitionistic logic. In Formalizing the dynamics of information, CSLI lecture notes, vol. 91, ed. M. Faller et al., 105–114. Stanford: CSLI Publications.
Moler, N., and P. Suppes. 1968. Quantifier-free axioms for constructive plane geometry. Compositio Mathematica 20: 143–152.
Mueller, I. 1981. Philosophy of mathematics and deductive structure in Euclid’s elements. Cambridge, Mass.: MIT.
Mumma, J. 2012. Constructive geometrical reasoning and diagrams. Synthese 186: 103–119.
Negri, S. 2003. Contraction-free sequent calculi for geometric theories with an application to Barr’s theorem. Archive for Mathematical Logic 42: 389–401.
Negri, S., and J. von Plato. 2001. Structural proof theory. Cambridge: Cambridge University Press.
Negri, S., and J. von Plato. 2005. The duality of classical and constructive notions and proofs. In From sets and types to topology and analysis: towards practicable foundations for constructive mathematics, ed. L. Crosilla and P. Schuster, 149–161. Oxford: Oxford University Press.
Negri, S., and von Plato, J. 2011. Proof analysis: a contribution to Hilbert’s last problem. Cambridge: Cambridge University Press.
Quaife, A. 1989. Automated development of Tarski’s geometry. Journal of Automated Reasoning 5: 97–118.
Pambuccian, V. 2004. Early examples of resource-consciousness. Studia Logica 77: 81–86.
Pambuccian, V. 2008. Axiomatizing geometric constructions. Journal of Applied Logic 6: 24–46.
Panza, M. 2011. Rethinking geometrical exactness. Historia Mathematica 38: 42–95.
von Plato, J. 1995. The axioms of constructive geometry. Annals of Pure and Applied Logic 76: 169–200.
von Plato, J. 2006. Normal form and existence property for derivations in Heyting arithmetic. Acta Philosophica Fennica 78: 159–163.
von Plato, J. 2007. In the shadows of the Löwenheim–Skolem theorem: early combinatorial analyses of mathematical proofs. The Bulletin of Symbolic Logic 13: 189–225.
von Plato, J. 2010a. Combinatorial analysis of proofs in projective and affine geometry. Annals of Pure and Applied Logic 162: 144–161.
von Plato, J. 2010b. Geometric proof theory of intuitionistic geometry. Workshop on constructive aspects of logic and mathematics, Kanazawa, 8–10 March 2010. <http://www.jaist.ac.jp/is/labs/ishihara-lab/wcalm2010/vonplato.pdf>
von Plato, J. 2013. Elements of logical reasoning. Cambridge: Cambridge University Press.
Poincaré, H. 1902. La science et l’hypothèse, 1968. Paris: Flammarion.
Prawitz, D. 1971. Ideas and results in proof theory. In Proceedings of the second Scandinavian logic symposium, J.E. Fenstad, 235–307. Amsterdam: North Holland.
Proclus 1970. A commentary on the first book of Euclid’s elements, ed. G.R. Morrow. Princeton: Princeton University Press.
Rathjen, M. 2005. The constructive Hilbert program and the limit of Martin-Löf type theory. Synthese 147: 81–120.
Rybowicz, M. 2003. On the normalization of numbers and functions defined by radicals. Journal of Symbolic Computation 35: 651–672.
Schroeder-Heister, P. 2006. Validity concepts in proof-theoretic semantics. Synthese 148: 525–571.
Schroeder-Heister, P. 2014. Proof-theoretic semantics. In The Stanford encyclopedia of philosophy (Summer 2014 edition), ed. E.N. Zalta.
Seeland, H. 1978. Algorithmische Theorien und konstruktive Geometrie. Stuttgart: Hochschulverlag.
Sieg, W. 1994. Mechanical procedures and mathematical experiences. In Mathematics and mind, ed. A. George, 71–117. Oxford: Oxford University Press.
Szczerba, L.W. 1986. Tarski and geometry. The Journal of Symbolic Logic 51: 907–912.
Tarski, A. 1959. What is elementary geometry? In L. Henkin et al. (1959), 16–29.
Tarski, A., and S. Givant. 1999. Tarski’s system of geometry. The Bulletin of System Logic 5: 175–214.
Troelstra, A.S. 1991. History of constructivism in the twentieth century. ITLI Prepublication series, ML-91-05, University of Amsterdam. <http://www.illc.uva.nl/Research/Reports/ML-1991-05.text.pdf>
Troelstra, A.S., and D. van Dalen. 1988. Constructivism in mathematics: an introduction, vol. 1. Amsterdam: North Holland.
Troelstra, A.S., and H. Schwichtenberg. 2000. Basic proof theory, 2nd ed. Cambridge: Cambridge University Press.
Turing, A.M. 1937. On computable numbers, with an application to the Entscheidungsproblem. Proceedings of the London Mathematical Society 42(s. 2): 230–265.
Van Bendegem, J.P. 2010. Finitism in geometry. In The stanford encyclopedia of philosophy, ed. E.N. Zalta, Spring 2010 ed. <http://plato.stanford.edu/archives/spr2010/entries/geometry-finitism>
Venturi, G. 2011. Hilbert, completeness and geometry. Rivista Italiana di Filosofia Analitica Junior 2: 82–104.
Vesley, R. 2000. Constructivity in geometry. History and Philosophy of Logic 20: 291–294.
Weyl, H. 1918. Das Kontinuum: Kritischen Untersuchungen über die Grundlagen der Analysis. Leipzig: Veit & Co.
Weyl, H. 1949. Philosophy of mathematics and natural science, English trans. O. Helmer. Princeton: Princeton University Press.
Acknowledgements
I wish to thank Davide Crippa, Gilles Dowek, Pierluigi Graziani, Clément Houtmann, Jean-Baptiste Joinet, John Mumma, Marco Panza, Jan von Plato, Claudio Sacerdoti Coen, and Davide Rinaldi for useful discussions and insightful comments on earlier versions of the paper. I would also like to thank two anonymous referees for valuable suggestions and critiques which helped to improve my work.
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2015 Springer International Publishing Switzerland
About this chapter
Cite this chapter
Naibo, A. (2015). Constructibility and Geometry. In: Lolli, G., Panza, M., Venturi, G. (eds) From Logic to Practice. Boston Studies in the Philosophy and History of Science, vol 308. Springer, Cham. https://doi.org/10.1007/978-3-319-10434-8_8
Download citation
DOI: https://doi.org/10.1007/978-3-319-10434-8_8
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-10433-1
Online ISBN: 978-3-319-10434-8
eBook Packages: Humanities, Social Sciences and LawPhilosophy and Religion (R0)