Frequency-Dependent Amplitude Versus Offset Variations in Porous Rocks with Aligned Fractures

Abstract

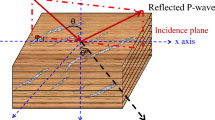
The theory of frequency-dependent amplitude versus offset (AVO) was developed for patchy-saturated model. In this work, we consider this theory in the case of an anisotropic medium based on a fractured-sandstone model. Thus, building on viscoelastic theory, we introduce a method for the computation of frequency-dependent AVO that is suitable for use in the case of an anisotropic medium. We use both analytical methods and numerical simulations to study P-P and P-S reflection coefficients, and results suggest that dispersion and anisotropy should not be neglected in AVO analysis. Indeed, for class I AVO reservoirs, the reflection magnitude of P-wave increases with frequency, while the responses of class II AVO reservoirs suggest that phase reversal occurs as frequency increases positively. In the case of class III AVO reservoirs, reflection magnitude decreases as frequency increases positively, while in the offset domain, the presence of anisotropy can distort or even reverse AVO responses. Thus, when compared to reflection coefficients for P-wave, reflection magnitude features of S-wave are more complex. The frequency-dependent AVO responses reported in this study provide insights for the interpretation of seismic anomalies in vertical transverse isotropy (VTI) dispersive reservoirs.

Appendices

Appendix A

1.1 Elastic moduli for low and high-frequency limits

In Eq. 1, low-frequency limit (relaxed) stiffnesses are as follows:

$$c_{33}^{r} = C_{\text{b}} \left[ {1 + \frac{{Z_{\text{N}} \left( {\alpha_{\text{b}} M_{\text{b}} - C_{\text{b}} } \right)^{2} }}{{C_{\text{b}} \left( {1 + Z_{\text{N}} \frac{{M_{\text{b}} L_{\text{b}} }}{{C_{\text{b}} }}} \right)}}} \right],$$

(17)

$$c_{13}^{r} = c_{33}^{r} \left[ {1 - 2\gamma_{\text{b}} \; + \;2\alpha_{\text{b}} \gamma_{\text{b}} \frac{{M_{\text{b}} }}{{C_{\text{b}} }}\frac{{\alpha_{\text{b}} \; + \;Z_{\text{N}} L_{\text{b}} }}{{1\; + \;Z_{\text{N}} \frac{{M_{\text{b}} L_{\text{b}} }}{{C_{\text{b}} }}}}} \right],$$

(18)

$$c_{11}^{r} = \frac{{\left( {c_{13}^{r} } \right)^{2} }}{{c_{33}^{r} }}\; + \;4\left[ {(1 - \gamma_{\text{b}} )\mu_{\text{b}} \; + \;\frac{{\alpha_{\text{b}}^{2} \gamma_{\text{b}}^{2} \frac{{M_{\text{b}} L_{\text{b}} }}{{C_{\text{b}} }}}}{{1 + Z_{\text{N}} \frac{{M_{\text{b}} L_{\text{b}} }}{{C_{\text{b}} }}}}} \right],$$

(19)

$$c_{55}^{r} = \left(\frac{1}{{\mu_{\text{b}} }} + Z_{\text{T}}\right )^{ - 1} ,$$

(20)

$$c_{66}^{r} = \mu_{\text{b}} .$$

(21)

Besides that, the high-frequency limited (unrelaxed) stiffnesses in Eq. 1 are given as follows:

$$c_{33}^{u} = C_{\text{b}} ,$$

(22)

$$c_{13}^{u} = C_{\text{b}} - 2\mu_{\text{b}} ,$$

(23)

$$c_{11}^{u} = C_{\text{b}} ,$$

(24)

$$c_{55}^{u} = \left( {\frac{1}{{\mu_{\text{b}} }} + Z_{\text{T}} } \right)^{ - 1} = c_{55}^{r} ,$$

(25)

$$c_{66}^{u} = \mu_{\text{b}} = c_{66}^{r} .$$

(26)

Here

$$\Delta_{\text{N}} = \frac{{Z_{\text{N}} L_{\text{b}} }}{{1 + Z_{\text{N}} L_{\text{b}} }},$$

(27)

$$\Delta_{\text{T}} \; = \;\frac{{\mu_{\text{b}} Z_{\text{T}} }}{{1\; + \;\mu_{\text{b}} Z_{\text{T}} }},$$

(28)

$$Z_{\text{N}} \equiv \mathop {\lim }\limits_{{h_{\text{c}} \to 0}} \frac{{h_{\text{c}} }}{{L_{\text{c}} }},$$

(29)

$$Z_{\text{T}} \equiv \mathop {\lim }\limits_{{h_{\text{c}} \to 0}} \frac{{h_{\text{c}} }}{{\mu_{\text{c}} }}.$$

(30)

The normalized frequency in Eq. 2 is as follows:

$$\varOmega = \frac{{\omega \eta H^{2} M_{\text{b}} }}{{4\kappa_{\text{b}} C_{\text{b}} L_{\text{b}} }}.$$

(31)

Appendix B

2.1 Empirical model for elastic modulus

On the basis of the empirical model presented by Krief et al. (1990), dry-rock bulk and shear modulus can be computed as follows:

$$\frac{{K_{\text{b}} }}{{K_{\text{s}} }} = \frac{{\mu_{\text{b}} }}{{\mu_{\text{s}} }} = \left( {1 - \phi } \right)^{{3/\left( {1 - \phi } \right)}} .$$

(32)

Rock permeability, $\kappa_{b}$, is computed using the equation derived by Carcione et al. (2000), as follows:

$$\kappa = \frac{{r_{\text{s}}^{2} \phi^{3} }}{{45(1 - \phi )^{2} }},$$

(33)

where $r_{\text{s}} = 20$ μm denotes average grain radius.

Appendix C

3.1 Slowness in VTI media

Horizontal slowness $s_{x}$ in VTI media is defined as follows:

$$s_{x} = \sin \theta /V(\theta ).$$

(34)

In this expression, $\theta$ is the angle of incidence, while $V(\theta )$ is the phase velocity of a qP-wave, given by Eq. 3.

Vertical slowness ${\text{s}}_{z}$ is expressed as follows:

$$s_{z} = \pm \frac{1}{\sqrt 2 }\left( {K_{1} \mp p.v.\sqrt {K_{1}^{2} - 4K_{2} K_{3} } } \right)^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-0pt} 2}}} .$$

(35)

In Eq. 35, as follows:

$$K_{1} = \rho \left( {\frac{1}{{p_{55} }} + \frac{1}{{p_{33} }}} \right) + \frac{1}{{p_{55} }}\left[ {\frac{{p_{13} }}{{p_{33} }}(p_{13} + p_{55} ) - p_{11} } \right]s_{x}^{2} ,$$

(36)

$$K_{2} = \frac{1}{{p_{33} }}(p_{11} s_{x}^{2} - \rho ),$$

(37)

and

$$K_{3} = s_{x}^{2} - \frac{\rho }{{p_{55} }}.$$

(38)

In this expression, $p_{11}$, $p_{13}$, $p_{33}$, $p_{55}$ are given by Eq. 1, and the signs in $s_{z}$ correspond to:

(+, −) downward qP-wave;

(+, +) downward qS-wave;

(−, −) upward qP-wave, and;

(−, +) upward qS-wave.