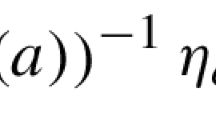Abstract
The Yang–Baxter and pentagon equations are two well-known equations of Mathematical Physic. If S is a set, a map \(s:S\times S\rightarrow S\times S\) is said to be a set-theoretical solution of the quantum Yang–Baxter equation if
where \(s_{12}=s\times {{\,\mathrm{id}\,}}_S\), \(s_{23}={{\,\mathrm{id}\,}}_S\times s\), and \(s_{13}=({{\,\mathrm{id}\,}}_S\times \tau )\,s_{12}\,({{\,\mathrm{id}\,}}_S\times \tau )\) and \(\tau \) is the flip map, i.e., the map on \(S\times S\) given by \(\tau (x,y)=(y,x)\). Instead, s is called a set-theoretical solution of the pentagon equation if
The main aim of this work is to display how solutions of the pentagon equation turn out to be a useful tool to obtain new solutions of the Yang–Baxter equation. Specifically, we present a new construction of solutions of the Yang–Baxter equation involving two specific solutions of the pentagon equation. To this end, we provide a method to obtain solutions of the pentagon equation on the matched product of two semigroups, that is a semigroup including the classical Zappa product.
Similar content being viewed by others
References
Baaj, S., Skandalis, G.: Unitaires multiplicatifs et dualité pour les produits croisés de C*-algèbres. Ann. Sci. Éc. Norm. Sup. 26(4), 425–488 (1993). https://doi.org/10.24033/asens.1677
Baaj, S., Skandalis, G.: Transformations pentagonales. C. R. Acad. Sci. Paris Sér. I Math. 327(7), 623–628 (1998). https://doi.org/10.1016/S0764-4442(99)80090-1
Castelli, M., Catino, F., Miccoli, M.M., Pinto, G.: Dynamical extensions of quasi-linear left cycle sets and the Yang–Baxter equation. J. Algebra Appl. 18(11), 1950220, 16 (2019). https://doi.org/10.1142/s0219498819502207
Castelli, M., Catino, F., Pinto, G.: A new family of set-theoretic solutions of the Yang–Baxter equation. Commun. Algebra 46(4), 1622–1629 (2018). https://doi.org/10.1080/00927872.2017.1350700
Castelli, M., Catino, F., Pinto, G.: Indecomposable involutive set-theoretic solutions of the Yang–Baxter equation. J. Pure Appl. Algebra 223(10), 4477–4493 (2019). https://doi.org/10.1016/j.jpaa.2019.01.017
Catino, F.: Factorizable semigroups. Semigroup Forum 36(2), 167–174 (1987). https://doi.org/10.1007/BF02575013
Catino, F., Colazzo, I., Stefanelli, P.: Semi-braces and the Yang–Baxter equation. J. Algebra 483, 163–187 (2017). https://doi.org/10.1016/j.jalgebra.2017.03.035
Catino, F., Colazzo, I., Stefanelli, P.: The matched product of the solutions to the Yang–Baxter Equation of finite order. Mediterr. J. Math. 17, 58 (2020). https://doi.org/10.1007/s00009-020-1483-y
Catino, F., Mazzotta, M., Miccoli, M.M.: Set-theoretical solutions of the pentagon equation on groups. Commun. Algebra 48(1), 83–92 (2020). https://doi.org/10.1080/00927872.2019.1632331
Cedó, F., Gateva-Ivanova, T., Smoktunowicz, A.: Braces and symmetric groups with special conditions. J. Pure Appl. Algebra 222(12), 3877–3890 (2018). https://doi.org/10.1016/j.jpaa.2018.02.012
Cedó, F., Jespers, E., Okniński, J.: Braces and the Yang–Baxter equation. Commun. Math. Phys. 327(1), 101–116 (2014). https://doi.org/10.1007/s00220-014-1935-y
Clifford, A.H., Preston, G.B.: The Algebraic Theory of Semigroups. Mathematical Surveys, No. 7, vol. I. American Mathematical Society, Providence (1961)
Cvetko-Vah, K., Verwimp, C.: Skew lattices and set-theoretic solutions of the Yang–Baxter equation. J. Algebra 542, 65–92 (2020). https://doi.org/10.1016/j.jalgebra.2019.10.007
Drinfel’d, V.G.: On some unsolved problems in quantum group theory. Quantum Groups (Leningrad, 1990). Lecture Notes in Mathematics, vol. 1510, pp. 1–8. Springer, Berlin (1992). https://doi.org/10.1007/BFb0101175
Etingof, P., Schedler, T., Soloviev, A.: Set-theoretical solutions to the quantum Yang–Baxter equation. Duke Math. J. 100(2), 169–209 (1999). https://doi.org/10.1215/S0012-7094-99-10007-X
Gateva-Ivanova, T.: Set-theoretic solutions of the Yang–Baxter equation, braces and symmetric groups. Adv. Math. 338, 649–701 (2018). https://doi.org/10.1016/j.aim.2018.09.005
Gateva-Ivanova, T., Van den Bergh, M.: Semigroups of \(I\)-type. J. Algebra 206(1), 97–112 (1998). https://doi.org/10.1006/jabr.1997.7399
Gomes, G.M.S.: On left quasinormal orthodox semigroups. Proc. R. Soc. Edinb. Sect. A 95(1–2), 59–71 (1983). https://doi.org/10.1017/S0308210500015791
Guarnieri, L., Vendramin, L.: Skew braces and the Yang–Baxter equation. Math. Comput. 86(307), 2519–2534 (2017). https://doi.org/10.1090/mcom/3161
Howie, J.M.: Fundamentals of Semigroup Theory. London Mathematical Society Monographs, vol. 12. Oxford University Press, New York (1995)
Jespers, E., Van Antwerpen, A.: Left semi-braces and solutions of the Yang–Baxter equation. Forum Math. 31(1), 241–263 (2019). https://doi.org/10.1515/forum-2018-0059
Jiang, L.-N., Liu, M.: On set-theoretical solution of the pentagon equation. Adv. Math. (China) 34(3), 331–337 (2005)
Kashaev, R.: Fully noncommutative discrete Liouville equation, in: Infinite analysis 2010–Developments in quantum integrable systems, RIMS Kôkyûroku Bessatsu, B28, Res. Inst. Math. Sci. (RIMS), Kyoto, pp. 89–98 (2011)
Kashaev, R.M., Reshetikhin, N.: Symmetrically factorizable groups and self-theoretical solutions of the Pentagon Equation. Quantum Groups. Contemporary Mathematics, vol. 433, pp. 267–279. American Mathematical Society, Providence (2007). https://doi.org/10.1090/conm/433/083
Kashaev, R.M., Sergeev, S.M.: On pentagon, ten-term, and tetrahedron relations. Commun. Math. Phys. 195(2), 309–319 (1998). https://doi.org/10.1007/s002200050391
Kunze, M.: Zappa products. Acta Math. Hungar. 41(3–4), 225–239 (1983). https://doi.org/10.1007/BF01961311
Lebed, V.: Cohomology of idempotent braidings with applications to factorizable monoids. Internat. J. Algebra Comput. 27(4), 421–454 (2017). https://doi.org/10.1142/S0218196717500229
Lebed, V., Vendramin, L.: Homology of left non-degenerate set-theoretic solutions to the Yang–Baxter equation. Adv. Math. 304, 1219–1261 (2017). https://doi.org/10.1016/j.aim.2016.09.024
Lu, J.-H., Yan, M., Zhu, Y.-C.: On the set-theoretical Yang–Baxter equation, Duke Math. J. 104(1), 1–18 (2000). https://doi.org/10.1215/S0012-7094-00-10411-5
Matsumoto, D.K., Shimizu, K.: Quiver-theoretical approach to dynamical Yang–Baxter maps. J. Algebra 507, 47–80 (2018). https://doi.org/10.1016/j.jalgebra.2018.04.003
Militaru, G.: The Hopf modules category and the Hopf equation. Commun. Algebra 26(10), 3071–3097 (1998). https://doi.org/10.1080/00927879808826329
Monzo, R.A.R.: Pre-compatible almost endomorphisms and semigroups whose cube is a band. Semigroup Forum 67(3), 355–372 (2003). https://doi.org/10.1007/s00233-001-0004-y
Rump, W.: A decomposition theorem for square-free unitary solutions of the quantum Yang–Baxter equation. Adv. Math. 193(1), 40–55 (2005). https://doi.org/10.1016/j.aim.2004.03.019
Rump, W.: Braces, radical rings, and the quantum Yang–Baxter equation. J. Algebra 307(1), 153–170 (2007). https://doi.org/10.1016/j.jalgebra.2006.03.040
Smoktunowicz, A.: On Engel groups, nilpotent groups, rings, braces and the Yang–Baxter equation. Trans. Am. Math. Soc. 370(9), 6535–6564 (2018). https://doi.org/10.1090/tran/7179
Smoktunowicz, A., Vendramin, L.: On skew braces (with an appendix by N. Byott and L. Vendramin). J. Comb. Algebra 2(1), 47–86 (2018). https://doi.org/10.4171/JCA/2-1-3
Yang, D.: The interplay between \(k\)-graphs and the Yang–Baxter equation. J. Algebra 451, 494–525 (2016). https://doi.org/10.1016/j.jalgebra.2016.01.001
Zakrzewski, S.: Poisson Lie groups and pentagonal transformations. Lett. Math. Phys. 24(1), 13–19 (1992). https://doi.org/10.1007/BF00429998
Acknowledgements
We would like to thank the referee for carefully reading our manuscript and for giving useful suggestions.
Funding
This work was partially supported by the Dipartimento di Matematica e Fisica “Ennio De Giorgi” - Università del Salento. The authors are members of GNSAGA (INdAM).
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Jan Okniński.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Catino, F., Mazzotta, M. & Stefanelli, P. Set-theoretical solutions of the Yang–Baxter and pentagon equations on semigroups. Semigroup Forum 101, 259–284 (2020). https://doi.org/10.1007/s00233-020-10100-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00233-020-10100-x