Abstract
Significant progress has been made to identify the cells and signaling molecules involved in the mechanobiological regulation of bone remodeling. It is now well accepted that osteocytes act as mechanosensory cells in bone expressing several signaling molecules such as nitric oxide (NO) and sclerostin (Scl) which are able to control bone remodeling responses. In this paper, we present a comprehensive multiscale computational model of bone remodeling which incorporates biochemical osteocyte feedback. The mechanostat theory is quantitatively incorporated into the model using mechanical feedback to control expression levels of NO and Scl. The catabolic signaling pathway RANK–RANKL–OPG is co-regulated via (continuous) PTH and NO, while the anabolic Wnt signaling pathway is described via competitive binding reactions between Wnt, Scl and the Wnt receptors LRP5/6. Using this novel model of bone remodeling, we investigate the effects of changes in the mechanical loading and hormonal environment on bone balance. Our numerical simulations show that we can calibrate the mechanostat anabolic and catabolic regulatory mechanisms so that they are mutually exclusive. This is consistent with previous models that use a Wolff-type law to regulate bone resorption and formation separately. Furthermore, mechanical feedback provides an effective mechanism to obtain physiological bone loss responses due to mechanical disuse and/or osteoporosis.











Similar content being viewed by others
Notes
For applications where it is necessary to simulate a perturbation of the \(\hbox {Ca}^{2+}\) response, a model which considers shorter time scales of \(\hbox {Ca}^{2+}\) exchange is required. This is beyond the scope to this paper.
See Sect. 2.1 for details on this ligand–receptor binding.
The effect of the co-receptor binding to Kremen could be taken into account by acknowledging the dependency of the number of LRP5/6 receptors on osteoblast precursor cells \(N_{{\mathsf {Ob}}_{\mathrm{p}}}^{{\mathsf {LRP5/6}}}\) via a decreasing function \(f_{\mathsf {Kr}}\) such that \(N_{{\mathsf {Ob}}_{\mathrm{p}}}^{{\mathsf {LRP5/6}}}=f_{\mathsf {Kr}}([{\mathsf {Kr}}])\).
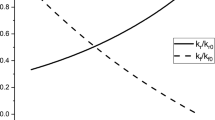
Note that although we represented the influence of mechanical loading via a Hill function, the function (\(\pi ^{{\varPsi _{\mathsf {bm}}}}_{{\mathsf {act}},\mathsf {NO}}\)) does not merely represent a chemical binding reaction but rather summarizes the highly complex phenomenon of osteocytes mechanosensation.
References
Ahlborg HG, Johnell O, Turner CH, Rannevik G, Karlsson MK (2003) Bone loss and bone size after menopause. N Engl J Med 349(4):327–334. https://doi.org/10.1056/NEJMoa022464
Ardawi MSM, Al-Kadi HA, Rouzi AA, Qari MH (2011) Determinants of serum sclerostin in healthy pre- and postmenopausal women. J Bone Miner Res 26(12):2812–2822. https://doi.org/10.1002/jbmr.479
Beaupré GS, Orr TE, Carter DR (1990) An approach for time-dependent bone modeling and remodeling-theoretical development. J Orthop Res 8(5):651–661. https://doi.org/10.1002/jor.1100080506
Bodine PVN, Komm BS (2007) Wnt signaling and osteoblastogenesis. Rev Endocr Metab Disord 7(1–2):33–39. https://doi.org/10.1007/s11154-006-9002-4
Bonewald LF (2011) The amazing osteocyte. J Bone Miner Res 26(2):229–238. https://doi.org/10.1002/jbmr.320
Bonewald LF, Johnson ML (2008) Osteocytes, mechanosensing and Wnt signaling. Bone 42(4):606–615. https://doi.org/10.1016/j.bone.2007.12.224
Bourhis E, Tam C, Franke Y, Bazan JF, Ernst J, Hwang J, Costa M, Cochran AG, Hannoush RN (2010) Reconstitution of a Frizzled8-Wnt3a-LRP6 signaling complex reveals multiple Wnt and Dkk1 binding sites on LRP6. J Biol Chem 285(12):9172–9179. https://doi.org/10.1074/jbc.M109.092130
Buenzli PR, Thomas CDL, Clement JG, Pivonka P (2013) Endocortical bone loss in osteoporosis: the role of bone surface availability. Int J Numer Method Biomed Eng. https://doi.org/10.1002/cnm.2567
Chow JWM, Fox SW, Lean JM, Chambers TJ (1998) Role of nitric oxide and prostaglandins in mechanically induced bone formation. J Bone Miner Res 13(6):1039–1044. https://doi.org/10.1359/jbmr.1998.13.6.1039
Doblaré M, García JM (2001) Application of an anisotropic bone-remodelling model based on a damage-repair theory to the analysis of the proximal femur before and after total hip replacement. J Biomech 34(9):1157–1170. https://doi.org/10.1016/S0021-9290(01)00069-0
Duncan RL, Turner CH (1995) Mechanotransduction and the functional response of bone to mechanical strain. Calcif Tissue Int 57:344–358. https://doi.org/10.1007/BF00302070
Fan X, Roy E, Zhu L, Murphy TC, Ackert-Bicknell C, Hart CM, Rosen C, Nanes MS, Rubin J (2004) Nitric oxide regulates receptor activator of nuclear factor-\(\kappa\)B ligand and osteoprotegerin expression in bone marrow stromal cells. Endocrinology 145(2):751–759. https://doi.org/10.1210/en.2003-0726
Folkesson J, Goldenstein J, Carballido-Gamio J, Kazakia G, Burghardt AJ, Rodriguez A, Krug R, de Papp AE, Link TM, Majumdar S (2011) Longitudinal evaluation of the effects of alendronate on MRI bone microarchitecture in postmenopausal osteopenic women. Bone 48(3):611–621. https://doi.org/10.1016/j.bone.2010.10.179
Frost HM (1987) Bone “mass” and the “mechanostat”: a proposal. Anat Rec 219(1):1–9. https://doi.org/10.1002/ar.1092190104
Frost HM (1996) Perspectives: a proposed general model of the “Mechanostat” (Suggestions from a new skeletal-biologic paradigm). Anat Rec 244(2):139–147. https://doi.org/10.1002/ar.1092190104
Galea GL, Lanyon LE, Price JS (2017) Sclerostin’s role in bone’s adaptive response to mechanical loading. Bone 96:38–44. https://doi.org/10.1016/j.bone.2016.10.008
Gaudio A, Pennisi P, Bratengeier C, Torrisi V, Lindner B, Mangiafico RA, Pulvirenti I, Hawa G, Tringali G, Fiore CE (2010) Increased sclerostin serum levels associated with bone formation and resorption markers in patients with immobilization-induced bone loss. J Clin Endocrinol Metab 95(5):2248–2253. https://doi.org/10.1210/jc.2010-0067
Genc K, Gopalakrishnan R, Kuklis MM, Maender CC, Rice AJ, Cavanagh P (2009) Using the enhanced daily load stimulus model to quantify the mechanical load and bone mineral density changes experienced by crew members on the international space station. In: Orthopaedic Research Society 56th annual meeting, New Orleans, LA, USA, p 1
Guthrie JR, Ebeling PR, Hopper JL, Barrett-Connor E, Dennerstein L, Dudley EC, Burger HG, Wark JD (1998) A prospective study of bone loss in menopausal Australian-born women. Osteoporos Int 8(3):282–290. https://doi.org/10.1007/s001980050066
Harris S, Dawson-Hughes B (1992) Rates of change in bone mineral density of the spine, heel, femoral neck and radius in healthy postmenopausal women. Bone Miner 17(1):87–95. https://doi.org/10.1016/0169-6009(92)90713-N
Holdsworth G, Slocombe P, Doyle C, Sweeney B, Veverka V, Le Riche K, Franklin RJ, Compson J, Brookings D, Turner J, Kennedy J, Garlish R, Shi J, Newnham L, McMillan D, Muzylak M, Carr MD, Henry AJ, Ceska T, Robinson MK (2012) Characterization of the interaction of sclerostin with the low density lipoprotein receptor-related protein (LRP) family of wnt co-receptors. J Biol Chem 287(32):26,464–26,477. https://doi.org/10.1074/jbc.M112.350108
Huiskes R, Weinans H, Grootenboer HJ, Dalstra M, Fudala B, Slooff TJ (1987) Adaptive bone-remodeling theory applied to prosthetic-design analysis. J Biomech 20(11–12):1135–1150. https://doi.org/10.1016/0021-9290(87)90030-3
Huiskes R, Ruimerman R, van Lenthe GH, Janssen JD (2000) Effects of mechanical forces on maintenance and adaptation of form in trabecular bone. Nature 405(6787):704–706. https://doi.org/10.1038/35015116
Jabbar S, Drury J, Fordham JN, Datta HK, Francis RM, Tuck SP (2011) Osteoprotegerin, RANKL and bone turnover in postmenopausal osteoporosis. J Clin Pathol 64(4):354–357. https://doi.org/10.1136/jcp.2010.086595
Jastrzebski S, Kalinowski J, Stolina M, Mirza F, Torreggiani E, Kalajzic I, Won HY, Lee SK, Lorenzo J (2013) Changes in bone sclerostin levels in mice after ovariectomy vary independently of changes in serum sclerostin levels. J Bone Miner Res 28(3):618–626. https://doi.org/10.1002/jbmr.1773
Jee WS, Tian XY (2005) The benefit of combining non-mechanical agents with mechanical loading: A perspective based on the Utah paradigm of skeletal physiology. J Musculoskelet Neuronal Interact 5(2):110–118
Keaveny TM, Crittenden DB, Bolognese MA, Genant HK, Engelke K, Oliveri B, Brown JP, Langdahl BL, Yan C, Grauer A, Libanati C (2017) Greater gains in spine and hip strength for romosozumab compared with teriparatide in postmenopausal women with low bone mass. J Bone Miner Res 32(9):1956–1962. https://doi.org/10.1002/jbmr.3176
Komarova SV, Smith RJ, Dixon SJ, Sims SM, Wahl LM (2003) Mathematical model predicts a critical role for osteoclast autocrine regulation in the control of bone remodeling. Bone. https://doi.org/10.1016/S8756-3282(03)00157-1
Langdahl B, Ferrari S, Dempster DW (2016) Bone modeling and remodeling: potential as therapeutic targets for the treatment of osteoporosis. Ther Adv Musculoskelet Dis 8(6):225–235. https://doi.org/10.1177/1759720X16670154
Langdahl BL, Libanati C, Crittenden DB, Bolognese MA, Brown JP, Daizadeh NS, Dokoupilova E, Engelke K, Finkelstein JS, Genant HK, Goemaere S, Hyldstrup L, Jodar-Gimeno E, Keaveny TM, Kendler D, Lakatos P, Maddox J, Malouf J, Massari FE, Molina JF, Ulla MR, Grauer A (2017) Romosozumab (sclerostin monoclonal antibody) versus teriparatide in postmenopausal women with osteoporosis transitioning from oral bisphosphonate therapy: a randomised, open-label, phase 3 trial. Lancet 390(10102):1585–1594. https://doi.org/10.1016/S0140-6736(17)31613-6
LeBlanc AD, Spector ER, Evans HJ, Sibonga JD (2007) Skeletal responses to space flight and the bed rest analog: a review. J Musculoskelet Neuronal Interact 7(1):33–47
Legrand E, Chappard D, Pascaretti C, Duquenne M, Krebs S, Rohmer V, Basle MF, Audran M (2000) Trabecular bone microarchitecture, bone mineral density, and vertebral fractures in male osteoporosis. J Bone Miner Res 15(1):13–19. https://doi.org/10.1359/jbmr.2000.15.1.13
Lemaire V, Tobin FL, Greller LD, Cho CR, Suva LJ (2004) Modeling the interactions between osteoblast and osteoclast activities in bone remodeling. J Theor Biol 229(3):293–309. https://doi.org/10.1016/j.jtbi.2004.03.023
Lerner U (2006) Bone remodeling in post-menopausal osteoporosis. J Dent Res 85(7):584–595. https://doi.org/10.1177/154405910608500703
Li X, Zhang Y, Kang H, Liu W, Liu P, Zhang J, Harris SE, Wu D (2005) Sclerostin binds to LRP5/6 and antagonizes canonical Wnt signaling. J Biol Chem 280(20):19,883–19,887. https://doi.org/10.1074/jbc.M413274200
Maldonado S, Borchers S, Findeisen R, Allgöwer F (2006) Mathematical modeling and analysis of force induced bone growth. In: International conference of the IEEE engineering in medicine and biology society, vol 1, pp 3154–3157. https://doi.org/10.1109/IEMBS.2006.260532
McClung M (2007) Role of RANKL inhibition in osteoporosis. Arthritis Res Ther 9(SUPPL.1):1–6. https://doi.org/10.1186/ar2167
Metz LN, Martin RB, Turner AS (2003) Histomorphometric analysis of the effects of osteocyte density on osteonal morphology and remodeling. Bone 33(5):753–759. https://doi.org/10.1016/S8756-3282(03)00245-X
Moustafa A, Sugiyama T, Prasad J, Zaman G, Gross TS, Lanyon LE, Price JS (2012) Mechanical loading-related changes in osteocyte sclerostin expression in mice are more closely associated with the subsequent osteogenic response than the peak strains engendered. Osteoporos Int 23(4):1225–1234. https://doi.org/10.1007/s00198-011-1656-4
Nakashima T, Hayashi M, Fukunaga T, Kurata K, Oh-Hora M, Feng J, Bonewald L, Kodama T, Wutz A, Wagner E, M Penninger J, Takayanagi H (2011) Evidence for osteocyte regulation of bone homeostasis through rankl expression. Nat Med 17:1231–4. https://doi.org/10.1038/nm.2452
Nazarian A, Muller J, Zurakowski D, Müller R, Snyder BD (2007) Densitometric, morphometric and mechanical distributions in the human proximal femur. J Biomech 40(11):2573–2579. https://doi.org/10.1016/j.jbiomech.2006.11.022
Nguyen J, Tang SY, Nguyen D, Alliston T (2013) Load regulates bone formation and Sclerostin expression through a TGF\(\beta\)-dependent mechanism. PLoS ONE. https://doi.org/10.1371/journal.pone.0053813
Nordin BEC, Need AG, Chatterton BE, Horowitz M, Morris HA (1990) The relative contributions of age and years since menopause to postmenopausal bone loss. J Clin Endocrinol Metab 70(1):83–88. https://doi.org/10.1210/jcem-70-1-83
Padhi D, Allison M, Kivitz AJ, Gutierrez MJ, Stouch B, Wang C, Jang G (2014) Multiple doses of sclerostin antibody romosozumab in healthy men and postmenopausal women with low bone mass: a randomized, double-blind, placebo-controlled study. J Clin Pharmacol 54(2):168–178. https://doi.org/10.1002/jcph.239
Pastrama MI, Scheiner S, Pivonka P, Hellmich C (2018) A mathematical multiscale model of bone remodeling, accounting for pore space-specific mechanosensation. Bone 107:208–221. https://doi.org/10.1016/j.bone.2017.11.009
Peterson MC, Riggs MM (2010) A physiologically based mathematical model of integrated calcium homeostasis and bone remodeling. Bone 46(1):49–63. https://doi.org/10.1016/j.bone.2009.08.053
Pivonka P, Komarova SV (2010) Mathematical modeling in bone biology: from intracellular signaling to tissue mechanics. Bone. https://doi.org/10.1016/j.bone.2010.04.601
Pivonka P, Zimak J, Smith DW, Gardiner BS, Dunstan CR, Sims NA, John Martin T, Mundy GR (2008) Model structure and control of bone remodeling: a theoretical study. Bone 43(2):249–263. https://doi.org/10.1016/j.bone.2008.03.025
Pivonka P, Buenzli P, Dunst C (2012) A systems approach to understanding bone cell interactions in health and disease. In: Cell interaction. InTech. https://doi.org/10.5772/51149
Pivonka P, Buenzli PR, Scheiner S, Hellmich C, Dunstan CR (2013) The influence of bone surface availability in bone remodelling—a mathematical model including coupled geometrical and biomechanical regulations of bone cells. Eng Struct. https://doi.org/10.1016/j.engstruct.2012.09.006
Pouilles J, Tremollieres F, Ribot C (2009) Effect of menopause on femoral and vertebral bone loss. J Bone Miner Res 10(10):1531–1536. https://doi.org/10.1002/jbmr.5650101014
Rahnert J, Fan X, Case N, Murphy TC, Grassi F, Sen B, Rubin J (2008) The role of nitric oxide in the mechanical repression of RANKL in bone stromal cells. Bone 43(1):48–54. https://doi.org/10.1016/j.bone.2008.03.006
Rawlinson SCF, El-haj AJ, Minter SL, Tavares IA, Bennett A, Lanyon LE (1991) Loading-related increases in prostaglandin production in cores of adult canine cancellous bone in vitro: a role for prostacyclin in adaptive bone remodeling? J Bone Miner Res 6(12):1345–1351. https://doi.org/10.1002/jbmr.5650061212
Robling AG, Niziolek PJ, Baldridge LA, Condon KW, Allen MR, Alam I, Mantila SM, Gluhak-Heinrich J, Bellido TM, Harris SE, Turner CH (2008) Mechanical stimulation of bone in vivo reduces osteocyte expression of Sost/sclerostin. J Biol Chem 283(9):5866–5875. https://doi.org/10.1074/jbc.M705092200
Rosa N, Simoes R, Magalhães FD, Marques AT (2015) From mechanical stimulus to bone formation: a review. Med Eng Phys 37(8):719–728. https://doi.org/10.1016/j.medengphy.2015.05.015
Scheiner S, Pivonka P, Hellmich C (2013) Coupling systems biology with multiscale mechanics, for computer simulations of bone remodeling. Comput Methods Appl Mech Eng 254:181–196. https://doi.org/10.1016/j.cma.2012.10.015
Scheiner S, Pivonka P, Smith DW, Dunstan CR, Hellmich C (2014) Mathematical modeling of postmenopausal osteoporosis and its treatment by the anti-catabolic drug denosumab. Int J Numer Method Biomed Eng 30(1):1–27. https://doi.org/10.1002/cnm.2584
Scheiner S, Pivonka P, Hellmich C (2016) Poromicromechanics reveals that physiological bone strains induce osteocyte-stimulating lacunar pressure. Biomech Model Mechanobiol 15:9–28. https://doi.org/10.1007/s10237-015-0704-y
Spatz JM, Wein MN, Gooi JH, Qu Y, Garr JL, Liu S, Barry KJ, Uda Y, Lai F, Dedic C, Balcells-Camps M, Kronenberg HM, Babij P, Pajevic PD (2015) The Wnt inhibitor sclerostin is up-regulated by mechanical unloading in osteocytes in vitro. J Biol Chem 290(27):16,744–16,758. https://doi.org/10.1074/jbc.M114.628313
Van Oers RFM, Van Rietbergen B, Ito K, Hilbers PAJ, Huiskes R (2011) A sclerostin-based theory for strain-induced bone formation. Biomech Model Mechanobiol 10(5):663–670. https://doi.org/10.1007/s10237-010-0264-0
Van Rietbergen B, Huiskes R, Weinans H, Sumner DR, Turner TM, Galante JO (1993) The mechanism of bone remodeling and resorption around press-fitted THA stems. J Biomech 26(4):369–382. https://doi.org/10.1016/0021-9290(93)90001-U
Wang Y, Pivonka P, Buenzli PR, Smith DW, Dunstan CR (2011) Computational modeling of interactions between multiple myeloma and the bone microenvironment. PLoS ONE 6(11):14–16. https://doi.org/10.1371/journal.pone.0027494
Xiong J, Piemontese M, Onal M, Campbell J, Goellner JJ, Dusevich V, Bonewald L, Manolagas SC, O’Brien CA (2015) Osteocytes, not osteoblasts or lining cells, are the main source of the RANKL required for osteoclast formation in remodeling bone. PLoS ONE 10(9):1–19. https://doi.org/10.1371/journal.pone.0138189
Zaman G, Pitsillides aa, Rawlinson SCF, Suswillo RFL, Mosley JR, Cheng MZ, Platts LaM, Hukkanen M, Polak JM, Lanyon LE (1999) Mechanical strain stimulates nitric oxide production by rapid activation of endothelial nitric oxide synthase in osteocytes. J Bone Miner Res 14(7):1123–1131. https://doi.org/10.1359/jbmr.1999.14.7.1123
Zaman G, Saxon LK, Sunters A, Hilton H, Underhill P, Williams D, Price JS, Lanyon LE (2010) Loading-related regulation of gene expression in bone in the contexts of estrogen deficiency, lack of estrogen receptor \(\alpha\) and disuse. Bone 46(3):628–642. https://doi.org/10.1016/j.bone.2009.10.021
Acknowledgements
Miss Madge Martin received a 12-month QUT School of Chemistry, Physics and Mechanical Engineering (CPME) Scholarship.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix 1: Formulation of the evolution of the osteocytes population
As written in Eq. (4), we chose here to represent the change in osteocytes concentration \({\mathsf {Ot}}\) as a function of bone matrix fraction \(f_{\mathsf {bm}}\). It is well accepted that osteocytes are differentiated osteoblastic cells buried in the bone matrix. As a result, one could argue that the evolution of the osteocytes population could be written as follows:
where one can note that the sink term is proportional to the concentration of osteoclasts \({\mathsf {Oc}}_{\mathrm{a}}\). Osteoclasts resorb bone and trigger osteocyte apoptosis by means of a local acidification. This action is quantified through the factor \(R^{{\mathsf {Oc}}_{\mathrm{a}}}_{{\mathsf {Ot}}}\) in Eq. (29). If we set the osteoblasts differentiation coefficient to \(D_{{\mathsf {Ob}}_{\mathrm{a}}} =\eta k_{\mathsf {form}}\) and the resorption coefficient to \(R^{{\mathsf {Oc}}_{\mathrm{a}}}_{{\mathsf {Ot}}}=\eta k_{\mathsf {res}}\), we obtain readily the formulation given in Eq. (4) in Sect. 2.1.
Appendix 2: Formulation of the RANKL production
We made the assumption that RANKL is expressed by osteocytes and osteoblasts precursors, following experimental evidence (Nakashima et al. 2011; Xiong et al. 2015). Therefore, the body production term for RANKL reads as follows:
where the total concentration of RANKL (bound and free) \([{\mathsf {RANKL}}]_{\mathsf {tot}}\) is defined as follows:
The parameters used in Eqs. (30), (31) are displayed in Table 3. When knocking out numerically the expression of RANKL by osteocytes (\(\beta _{{\mathsf {RANKL}},{\mathsf {Ot}}}=0\)), we obtain the osteopetrotic phenotype observed experimentally (Nakashima et al. 2011). This result is illustrated in Fig. 12, where the steady state (dashed line) is compared to the RANKL-deficient state (solid line).
Appendix 3: Properties of matrix and fluid in the micro-mechanical model
The stiffness tensor of the bone matrix reads as follows (Kelvin notation):
The stiffness tensor of the saturating fluid is:
where the bulk modulus and the shear modulus are, respectively, \(k_{\mathsf {H_2 O}}=2.3\ \mathrm {GPa}\) and \(\mu _{\mathsf {H_2 O}}=0\ \mathrm {GPa}\), and \(\mathbb {J}\) is the volumetric part of the fourth-order unit tensor \(\mathbb {I}\), and \(\mathbb {K}\) is its deviatoric part, \(\mathbb {K}=\mathbb {I}-\mathbb {J}\).
Appendix 4: Derivation of free sclerostin concentration
The following equation describes sclerostin balance:
with
After inserting Eqs. (18) and (35) into Eq. (34), we uncover a second-order polynomial equation on the concentration of free sclerostin \([{\mathsf {Scl}}]\):
where
The term A is a sum of positive quantities, one of them being strictly positive, and the term C is the opposite of the product of strictly positive quantities except for the concentration of osteocytes that we can assume to be non-null. From linear algebra, we know the product of the solutions is equal to \(\frac{C}{A}<0\). Thus, provided solutions are in the real space, there are exactly two roots of opposed signs satisfying this equation. Hence, as the solution of the equation is a concentration, the only acceptable root is:
Appendix 5: Nomenclature
The abbreviations used in the present paper are summarized in Table 5.
Rights and permissions
About this article
Cite this article
Martin, M., Sansalone, V., Cooper, D.M.L. et al. Mechanobiological osteocyte feedback drives mechanostat regulation of bone in a multiscale computational model. Biomech Model Mechanobiol 18, 1475–1496 (2019). https://doi.org/10.1007/s10237-019-01158-w
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10237-019-01158-w