Abstract
This paper examines the competition between organic and non-organic firms, their incentives to undertake a horizontal merger, and the effect of mergers on firms’ market shares. We also consider an alternative setting where one firm can acquire its rival. For generality, we allow for product differentiation, demand, and cost asymmetries. Our results show that both organic and non-organic firms, despite their cost asymmetries and demand differentials, have incentives to merge under large conditions. When demand and cost differentials are significant, we identify settings under which a firm (either organic or non-organic) purchases its rival, to subsequently shut it down, and yet increase its profits. We then study under which conditions the merger can be welfare improving, which is more likely when goods are highly differentiated and their production costs are relatively symmetric.






Similar content being viewed by others
Notes
Some of the largest operations include Danone purchasing WhiteWave in July 2016 for US$12.5 billion, TreeHouse Foods acquiring Ralcorp (from ConAgra) in November 2015 for US$2.7 billion, Hormel Foods Corporation purchasing Applegate Farms in May 2015 for US$775 million, or Coca-Cola company acquiring AdeS from Unilever in June 2016 for US$ 575 million.
Other examples include Amplify Snack Brands purchasing Boundless Nutrition (an allergen-free, non-GMO, snack manufacturer) in May 2016; Preferred Popcorn acquiring K&W Popcorn (a producer of organic popcorn) in April 2016; ConAgra Foods purchasing Blake’s All Natural Food (organic frozen meals) in May 2015; General Meals acquiring Annie’s (organic foods and snacks) in September 2014; J.M. Smucker purchasing Sahale Snacks (gluten free and non-GMO snacks) in August 2014; the Millstone Coffee Company (organic coffee manufacturer) in November 2008; and WhiteWave Foods acquiring So Delicious (organic and dairy-free foods and beverages) in September 2014.
Other examples include SunOpta (a Canadian organic and specialty food company) acquiring Sunrise Growers (a leading producer of organic foods) and Niagara Natural Food Snacks (healthy fruit snacks) in October 2015; Natural American Foods purchasing Sweet Harvest Foods (a producer of organic peanut butter, honey, and syrups) in December 2016; and Fresca Foods acquiring Wonderfully Raw and Open Road Snacks (both firms produce organic, gluten-free and vegan snacks) in October 2015 and February 2017, respectively.
Krissoff (1998) summarizes studies on consumer demand for organic food, indicating that a large proportion of consumers prefer organic foods because of taste, appearance, or personal health reasons. For other articles evaluating consumer perceptions of organic product quality, see Grunert (2007) and Agyekum et al. (2015).
Our paper therefore connects with the literature on mergers between firms with asymmetric costs, such as Fauli-Oller (2002), which finds that the merger chooses to close the plant exhibiting a significant cost inefficiency, thus exclusively producing in the efficient plant. Our paper finds a similar result in equilibrium, but allowing for product differentiation and demand differentials, evaluating afterwards its welfare implications.
Butler (2002) analyzes dairy production in California, reporting a 10–20% cost differential (on $U.S. per cow); Klonsky (2012) examines products such as field corn, broccoli, almonds, and walnuts, finding cost differentials between $51 and $312 per acre; Taylor and Granatstein (2013) studies Washington State apples, reporting a cost differential of 5–10% per acre; and McBride et al. (2015), which examines corn, soybeans, and wheat, finding cost differentials between $55 and $125. Some empirical studies analyzing other products, however, find a negative cost differential, thus reflecting that organic goods are cheaper to produce than their non-organic varieties, such as corn in Indiana, Clark (2009), alfalfa, and lettuce in California, Klonsky (2012), and Idaho and Washington State potatoes, Ecotrust (2016).
Hershey Co. acquired the organic non-GMO companies Dagoba in October 2006, Krave Pure Foods in February 2015, and barkTHINS in April 2016. In addition, Hershey has been replacing sugar from sugar beets for non-GMO cane sugar, accounting for more than 75% of its sugar use in February 2016. A similar argument applies to Flower Foods, Inc. (a firm mainly selling non-organic products before 2015), which acquired two organic producers, Dave’s Killer Bread in August 2015 and Alpine Valley Bread in September 2015. After these acquisitions, the acquirer, Flower Foods, Inc., significantly reduced its non-organic output while increasing the production of Dave’s Killer Bread by four times relative to pre-acquisition levels (see Howard (2009), Gutierrez (2016), and MarketLine (2017).
The J.M. Smucker Company (seller of non-organic products in 2008) acquired the organic firm Millstone Coffee in November 2008. The acquirer, however, discontinued Millstone Coffee in September 2016, citing lack of sustainable demand. Their organic coffee brand, therefore, disappeared since the company did not acquire another organic coffee brand, nor developed its own, as reported by The Vending Times in August 25, 2016
Gelves (2014) considers an oligopoly setting with N firms similar to that in Salant et al. (1983), but allowing for cost asymmetries and product differentiation. While Salant et al. (1983) show that mergers can only be profitable if merging firms represent a large percentage of companies in the industry (i.e., the “80% rule”), Gelves (2014) demonstrates that cost asymmetry increases firms’ incentives to merge even if they sustain a relatively small percentage of industry sales.
This demand specification is, thus, similar to that of Singh and Vives (1984) for the analysis of firms’ incentives to compete in either quantities or prices when they produce differentiated products. These demand functions assume, for simplicity, that price sensitivities are symmetric between goods. Assuming asymmetric price sensitivities, however, leads to highly intractable results, which do not allow for a clear economic interpretation.
Furthermore, if demands satisfy ak = aj, and both products are equally costly, ck = cj, this output level reduces to the standard result in duopoly markets with symmetric firms and homogeneous products, i.e., \(\frac { a_{k}-c_{k}}{3}\).
Note that both cutoffs \(c_{k}^{\text {NM}}\) and \(c_{j}^{\text {NM}}\) are less demanding than all the cutoffs we identify in subsequent sections of the paper, which implies that conditions \(c_{k}<c_{k}^{\text {NM}}\) and \(c_{j}<c_{j}^{\text {NM}}\) hold throughout our analysis.
In addition, we solve for ck in this inequality to obtain \( c_{k}>{c_{j}^{M}}\equiv \left (a_{k}-\frac {a_{j}}{\lambda } \right ) +\frac {c_{j}} {\lambda } \) . Graphically, cutoff \({c_{j}^{M}}\) is easier to plot in the (cj,ck)-quadrant, and to compare against other cutoffs found above.
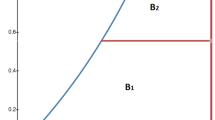
Comparing cutoff \({c_{O}^{M}}\) against that under no mergers, \(c_{O}^{\text {NM}}\), we find that \(c_{O}^{\text {NM}}\equiv \left (a_{O}-\frac {\lambda } {2}a_{\text {NO}}\right ) + \frac {\lambda } {2}c_{\text {NO}}\) originates above the vertical intercept of cutoff \( {c_{O}^{M}}\), aO − λaNO. In addition, cutoff \(c_{O}^{\text {NM}}\) crosses \({c_{O}^{M}}\) at cNO = aNO and a height of cO = aO. Therefore, cutoff \(c_{O}^{\text {NM}}\) divides Region III into two areas: (1) if \( {c_{O}^{M}}\leq c_{O}<c_{O}^{\text {NM}}\), the organic firm shuts down under the merger, but would produce a positive output if no merger occurs; and (2) if \( c_{O}\geq c_{O}^{\text {NM}}\), the organic firm shuts down both when the merger occurs and when it does not. Since we have showed that a merger can be sustained in Region III, the discussion about whether the organic firm would have been active had the merger not occurred is inconsequential. A similar argument applies to cutoff \(c_{\text {NO}}^{\text {NM}}\), which splits Region I into two areas.
Note that cutoff \({c_{O}^{M}}\) originates in the positive quadrant if aO − λaNO ≥ 0, or aO ≥ λaNO; while cutoff \( c_{\text {NO}}^{M}\) does when \(a_{O}-\frac {a_{\text {NO}}}{\lambda } \geq 0\), or \(a_{O}\geq \frac {a_{\text {NO}}}{\lambda } \) . That is, when aO is low, aO < λaNO, both cutoffs originate in the negative quadrant; when aO takes intermediate values, \(\lambda a_{\text {NO}}\leq a_{O}<\frac {a_{\text {NO}}}{\lambda } \) , only cutoff \({c_{O}^{M}}\) originates in the positive quadrant; and when aO is relatively high, \(a_{O}\geq \frac {a_{\text {NO}}}{\lambda } \), both cutoffs start at the positive quadrant.
If, in addition, firms face the same demand, i.e., aO = aNO = a, both cutoffs originate at zero, thus coinciding with the 45-degree line. In this context, when the non-organic firm enjoys even a minor cost advantage, the merged firm chooses only this plant to be active.
An alternative sharing rule could assign every firm k a larger share of profits when it produces a larger share of output, as follows: \(\frac {\pi _{k}^{M,k}}{q_{k}^{M,k}/Q^{M,k}}\) in Region I, \(\frac {\pi ^{M,\text {Both}}}{ q_{k}^{M,\text {Both}}/Q^{M,\text {Both}}}\) in Region II, and \(\frac {\pi _{j}^{M,j}}{ q_{j}^{M,j}/Q^{M,j}}\) in Region III, where QM,x denotes aggregate output in setting x = {O,Both,NO}. However, this profit sharing rule entails that the non-organic (organic) firm would not receive any profits from the organic (non-organic) plant, which is the only firm remaining active after the merger in Region I (III, respectively).
However, since λ = 1, cutoff \(c_{O}^{\text {SO}}\) originates at a low vertical intercept, aO − aNO, which lies in Region I. Therefore, insufficient output can only arise if the organic firm’s cost advantage is extremely strong.
References
Agyekum CK, Haifeng H, Agyeiwaa A (2015) Consumer perception of product quality. Microecon Macroecon 3(2):25–29
Butler L (2002) Survey quantifies cost of organic milk production in California. Calif Agric 56(5):157–162
Clark SF (2009) The profitability of transitioning to organic grain crops in Indiana. Amer J Agric Econ 91(5):1497–1504
Ecotrust (2016) Differentiated cost of production in the Northwest: an analysis of six food categories. Cascadia Foodshed Financing Project. Storage crops. https://ecotrust.org/media/CFFP_beef_8_16_16.pdf
Escrihuela-Villar M (2011) On welfare effects of horizontal mergers with product differentiation. J Econ Financ Admin Sci 16(30):7–12
Farrell J, Shapiro C (1990) Horizontal mergers: an equilibrium analysis. Amer Econ Rev 80:107–26
Fauli-Oller R (2002) Mergers between asymmetric firms: profitability and welfare. Manchester Sch 70:77–87
Gelves JA (2014) Differentiation and cost asymmetry: solving the merger paradox. Int J Econ Bus 21(3):321–340
Grunert KG (2007) How Consumers perceive food quality. In: Frewer L, Van Trijp H (eds) Understanding Consumers of Food Products. Woodhead Publishing Series in Food Science, Technology and Nutrition. Woodhead Publishing, pp 181–99
Gutierrez D (2016) Hershey introduces GMO-free chocolate after dropping transgenic sugar beets from ingredients, Natural News
Häckner J (2000) A note on price and quantity competition in differentiated oligopolies. J Econ Theory 93:233–239
Howard PH (2009) Consolidation in the North American organic food processing sector, 1997 to 2007. Int J Sociol Agric Food 16(1):13–30
Kao T, Menezes F (2010) Welfare-Enhancing Mergers under product differentiation. Manchester Sch 78(4):290–301
Klonsky K (2012) Comparison of production costs and resource use for organic and conventional production systems. Amer J Agric Econ 94(2):314–21
Krissoff B (1998) Emergence of U.S. Organic agriculture: can we compete? discussion. Amer J Agric Econ 80((5), Proceedings Issue):1130–1133
MarketLine (2017) Flowers Foods, Inc. - Mergers & Acquisitions (M&A), Partnerships & Alliances and Investment Report. Report code: 3F7F2D82-F8DA-4C94-BD9F-41E340E851EC. Available at: https://store.marketline.com/report/3f7f2d82-f8da-4c94-bd9f-41e340e851ec--flowers-foods-inc-mergers-acquisitions-ma-partnerships-alliances-and-investment-report/
McBride WD, Greene C, Foreman L, Ali M (2015) The profit potential of certified organic field crop production. United States Department of Agriculture, Economic Research Service, Economic Research Report No. 188
Norman G, Pepall L (2000) Profitable mergers in a Cournot model of spatial competition. South Econ J 66(3):667–681
Norman G, Pepall L, Richards D (2005) Product differentiation, cost-reducing mergers, and consumer welfare. Can J Econ 38(4):1204–1223
Salant SW, Switzer S, Reynolds RJ (1983) Losses from horizontal merger: the effects of an exogenous change in industry structure on Cournot-Nash equilibrium. Q J Econ 98:185–99
Savorelli L (2012) Asymmetric cross-price effects and collusion. Res Econ 66 (4):375–382
Singh N, Vives X (1984) Price and quantity competition in a differentiated duopoly. RAND J Econ 15(4):546–554
Taylor M, Granatstein D (2013) A cost comparison of organic and conventional apple production in the state of Washington. Crop Management. Available online at https://dl.sciencesocieties.org/publications/cm/pdfs/12/1/2013-0429-05-RS
Zanchettin P (2006) Differentiated duopoly with asymmetric costs. J Econ Manag Strateg 15(4):999–1015
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix
Appendix 1 - Numerical Example
We evaluate cutoffs c1 through c6 at M= N = 5 firms throughout this Appendix. A similar analysis applies to settings with different numbers of firms.
Benchmark Case
Applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.79 \ \)and \(c_{\text {NO}}^{M}=0.16\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I cannot be sustained in equilibrium; Region II can be supported for all costs satisfying 0.25 ≤ cO < 0.79; and Region III for all cO ≥ 0.79. From Proposition 1, we obtain cutoffs c1 through c6 (see first row of Table 4). We can then conclude that: (1) the range of parameters [c3,c4] = [0.67, 0.99] is compatible with Region II as long as cO ∈ [0.67, 0.79], which entails that this region can be supported in equilibrium when costs are relatively high and (2) the range of parameters [c5,c6] = [0.68, 0.97] is compatible for all values of cO in Region III, implying that this region can be sustained for all admissible cO.
We next examine the previous cutoffs on rows 2–5 of Table 1.
Lower Cost
cNO = 1/10. Applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.71\) and \(c_{\text {NO}}^{M}=-0.13\). Since cO > 0 and cO ≥ cNO by definition, Region I cannot be sustained in equilibrium. Region II can be sustained for all cost satisfying 0.1 ≤ cO < 0.71; and Region III for all cO ≥ 0.71. Proposition 1 implies that: (1) the range of parameters [c3,c4] = [0.55, 0.99] is compatible with Region II as long as cO ∈ [0.55, 0.71], which entails that this region can be supported in equilibrium when costs are moderately high and (2) the range of parameters [c5,c6] = [0.56, 0.96] is compatible for all values of cO in Region III, entailing that this region can be sustained for all admissible cO.
Higher Demand
aNO = 1 . Applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.62\) and \(c_{\text {NO}}^{M}=-0.5\). Since cutoff \( c_{\text {NO}}^{M}<0\) and costs must be positive by definition, Region I cannot be sustained in equilibrium. Region II can be sustained for all costs satisfying 0.25 ≤ cO < 0.62 and Region III for all remaining costs cO ≥ 0.62. Proposition 1 implies that: (1) the range of parameters [c3,c4] = [0.41, 0.98] is compatible with Region II as long as cO ∈ [0.42, 0.95] and (2) the range of parameters [c5,c6] = [0.42, 0.95] is compatible for all values of cO in Region III, implying that this region can be sustained for all admissible cO.
Homogeneous Goods
λ = 1. Applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=c_{\text {NO}}^{M}=0.58\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I can be sustained for all costs satisfying 0.25 ≤ cO < 0.58. Region II cannot be sustained since cutoffs coincide \(c_{\text {NO}}^{M}=c_{O}={c_{O}^{M}}\) and Region III can be sustained for all cO ≥ 0.58. Proposition 1 implies that: (1) the range of parameters [c1,c2] = [0.60, 0.69] is not compatible with Region I, implying that this region cannot be sustained in equilibrium; and (2) the range of parameters [c5,c6] = [0.59, 0.70] is compatible for all values of cO in Region III, implying that this region can be sustained for all admissible cO.
λ = 1 and aNO = 1
Applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=c_{\text {NO}}^{M}=0.25\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I cannot be sustained in equilibrium. Region II cannot be sustained since cutoffs \( {c_{O}^{M}}\) and \(c_{\text {NO}}^{M}\) coincide and Region III can be sustained for all cO ≥ 0.25. Proposition 1 implies that the range of parameters [c5,c6] = [0.27, 0.47] is compatible for all values of cO in Region III, entailing that this region can be sustained for all admissible cO.
Appendix 2 - Extension to N Organic and M Non-Organic Firms
Second-Stage Output
Case 1, No Merger
If one or more firms chose to not merge during the first period, a merger does not occur, leading each firm to simultaneously and independently set its own output. In particular, every organic firm chooses its output level qk to solve the following:
where \(q_{-k}={\sum }_{i\neq j}^{N}q_{i}\) denotes the aggregate output by the other N − 1 organic firms, and \(Q_{j}={\sum }_{r=1}^{M}q_{r}\) represents the aggregate output by all non-organic firms. Differentiating with respect to qk, we obtain a best response function as follows:
Invoking symmetry (qk = qi for every pair of organic firms k and i ), we find the following:
Solving a similar problem for every non-organic firm j, we have as follows:
which yields a symmetric best response function to qk(q−k,Qj), that is,
Invoking symmetry (qj = qr for every pair of non-organic firms j and r), we find the following:
In a symmetric equilibrium, aggregate output from organic firms is Qk = Nqk while that from non-organic firms is Qj = Mqj. Inserting these two properties in expressions (4) and (5), and simultaneously solving, yields the following:
Last, equilibrium profits under no merger for the organic and non-organic firms are as follows:
and
Firm k’s output under no merger is positive if its cost satisfies \(c_{k} <c_{N,k}^{\text {NM}} \equiv \left (a_{k}-\frac {M \lambda a_{j}}{M+1}\right ) + \frac { M \lambda c_{j}}{M+1}\). Similarly firm j produces a positive output if only if \(c_{j}<c_{M,j}^{\text {NM}} \equiv \left (a_{j}-\frac {N\lambda a_{k}}{N+1} \right ) +\frac {N\lambda c_{k}}{N+1}\).
Case 2, Merger
As in the model with only one firm of each type, we next examine each case separately.
Both Firms Are Active
When all types of firm are active, they maximize their joint profits as follows:
Differentiating with respect to qk and qj, and invoking symmetry for organic firms, q−k = (N − 1)qk, and for non-organic firms, q−j = (M − 1)qj, we obtain the following:
yielding equilibrium profits of the following:
and
Firm k’s output under the merger is positive if its cost satisfies \(c_{k} < c_{N,k}^{M} \equiv \left (a_{k}-\frac {(M+N) \lambda a_{j}}{2M}\right ) + \frac {(M+N) \lambda c_{j}}{2M}\).
Only Firm k Is Active
If the merged firm shuts down all non-organic firms j, its profit-maximization problem becomes the following:
Differentiating with respect to qk and invoking symmetry, q−k = (N − 1)qk, yields output as follows:
with associated profits as follow:
Comparing the profits that the merged firm obtains from keeping producing both goods, πM,Both, against those where only firm k remains active, \(\pi _{k}^{M,k}\), we obtain the following lemma. For presentation purposes, recall that firm j’s output under the merger is positive if its cost cj satisfies \(c_{j}<a_{j}-\frac {(M+N)\lambda } {2N}(a_{k}-c_{k})\) which is equivalent to \(c_{k}<c_{M,j}^{M}\equiv \left (a_{k}-\frac {2Na_{j}}{ (M+N)\lambda } \right ) +\frac {2Nc_{j}}{(M+N)\lambda } \).
As in the main body of the paper, our subsequent analysis considers firm k as the organic producer, firm O, and its rival j as the non-organic firm, NO.
Lemma 3
The following three regions can arise in the(cNO,cO)-quadrant:
- 1.
Region I. Only firm Oproduces positive output if\(c_{O}<c_{M,NO}^{M}\).
- 2.
Region II. Both firms produce positive outputif\( c_{M,NO}^{M}\leq c_{O}<c_{N,O}^{M}\).
- 3.
Region III. Only firm NOproduces positive outputif\(c_{O}\geq c_{N,O}^{M}\).
Proof of Lemma 3.
The profit difference \(\pi ^{M,\text {Both}}-\pi _{O}^{M,O}\) yields a U-shaped curve, which becomes zero at exactly \( c_{O}=c_{M,NO}^{M}\equiv \left (a_{O}-\frac {2Na_{NO}}{(M+N)\lambda } \right ) + \frac {2Nc_{NO}}{(M+N)\lambda } \) . As a consequence, \(\pi ^{M,\text {Both}}\geq \pi _{O}^{M,O}\) holds for all parameter values. For the non-organic firm, the profit difference \(\pi ^{M,\text {Both}}-\pi _{\text {NO}}^{M,\text {NO}}\) exhibits a similar shape, becoming zero at \(c_{O}=c_{N,O}^{M}\), thus implying that \(\pi ^{M,\text {Both}}\geq \pi _{\text {NO}}^{M,\text {NO}}\) also holds for all parameter values. Summarizing, it is profitable to maintain both firms active, rather than shutting one of them down.
We can now compare cutoffs \(c_{N,O}^{M}\) and \(c_{M,\text {NO}}^{M}\). First, cutoff \( c_{N,O}^{M}\) originates above \(c_{M,\text {NO}}^{M}\) since their vertical intercepts satisfy \(a_{O}-\frac {(M+N)\lambda a_{\text {NO}}}{2M}>a_{O}-\frac {2Na_{\text {NO}}}{ (M+N)\lambda } \) , which holds given that λ ∈ [0, 1] by definition. Second, the positive slope of cutoff \(c_{N,O}^{M} \)is \(\frac { (M+N)\lambda } {2M}\), whereas that of cutoff \(c_{M,\text {NO}}^{M}\) is \(\frac {2N}{ (M+N)\lambda } \) , thus indicating that cutoff \(c_{M,\text {NO}}^{M}\) grows faster than \(c_{N,O}^{M}\) does. In addition, cutoffs \(c_{N,O}^{M}\) and \( c_{M,\text {NO}}^{M} \) cross each other at cNO = aNO and a height of cO = aO. Recalling that aO > cO for every firm O, only three regions can be sustained in the (cNO,cO)-quadrant: (1) when \( c_{O}<c_{M,NO}^{M}\), only firm O is active; (2) when \(c_{M,NO}^{M}\leq c_{O}<c_{N,O}^{M}\), both firms are active; and (3) when \(c_{O}\geq c_{N,O}^{M}\), only firm NO is active. □
We next examine how the results in Lemma 3 differ relative to its analogous result in the main body of the paper, Lemma 1.
Corollary 2
When the number of organic and non-organic firms coincide,M = N,cutoff\(c_{N,O}^{M}\)becomes\( {c_{O}^{M}}\)andcutoff\(c_{M,\text {NO}}^{M}\)becomes\( c_{\text {NO}}^{M}\).When the differenceM − Nincreases,both cutoffs\(c_{M,\text {NO}}^{M}\)and\(c_{N,O}^{M}\)shiftupwards, shrinking Regions II and III while expanding Region I.
Therefore, our results in Lemma 1 (which assumes one firm of each type) coincide with those where M firms of each type exist, regardless of how large the number of firms is, i.e., for all M = N ≥ 1. The findings depicted in Fig. 1 readily apply in this context.
However, as the difference in the number of non-organic and organic firms (as captured by M − N) increases, organic firms produce a positive output under more restrictive cost conditions. As a result, when the costs of organic firms are extremely high, as in Region III (see Fig. 1 in the main body of the paper), the merged firm produces non-organic goods alone. When the costs of both firms are relatively similar and high, as in Region II, they both remain active after the merger. In the rest of the cases, the merged firm produces organic goods alone as in Region I.
When products are homogeneous, λ = 1, our results in the main body of the paper still apply when the number of organic and non-organic firms coincides, M = N, as depicted in Fig. 2a. However, when M > N, cutoff \( c_{N,O}^{M}\) originates below \(c_{M,O}^{M}\), giving rise to Region II, where both firms are active; a result that could not be sustained when products are homogeneous and M = N. In contrast, when products are completely differentiated, λ = 0, all cutoffs identified in Lemma 3 simplify to aO (i.e., they all become a horizontal line originating at aO), yielding only one possible outcome in equilibrium: Region I, where the organic firm is the only active plant.
First Stage
For each (cNO,cO)-pair, every firm O anticipates the output profile that will emerge in the second stage of the game, i.e., Regions I–III. For completeness, we consider that during the first stage firms choose whether to merge, and subsequently examine how the results would change if, instead, one firm is allowed to acquire its rival.
In the first stage, every firm chooses whether to merge or not. In particular, for each regions I–III, the firm compares the profits that it currently obtains as an independent firm, \(\pi _{N,O}^{\text {NM}}\), against the profits it would obtain under the merger: HCode \(\frac {\pi _{N,O}^{M,O}}{M+N}\) in Region I, \(\frac {\pi _{N,M}^{M,\text {Both}}}{M+N}\) in Region II, and \(\frac {\pi _{M,NO}^{M,NO}}{M+N}\) in Region III. For simplicity, we assume that firms evenly share merger profits.
Proposition 3
During the first stage, every firm O chooses to merge as follows:
- 1.
If (cNO,cO)-pairslie in Region I, firm O merges if and only ifcO ∈ [c1,c2].
- 2.
If (cNO,cO)-pairslie in Region II, firm O merges if and only ifcO ∈ [c3,c4].
- 3.
If (cNO,cO)-pairslie in Region III, firm O merges if and only ifcO ∈ [c5,c6].
For compactness, cutoffsc1 − c6aredefined in the proof below.
Proof of Proposition 3.
First, consider (cNO,cO)-pairs in Region I, i.e., \(c_{O}<c_{M,\text {NO}}^{M}\). In this region, only firm O operates under a merger in the second stage. Therefore, every firm O chooses to merge in the first stage if and only if its share of profits under the merger, \(\frac {\pi _{N,O}^{M,O}}{M+N}\), exceeds the profits it would obtain as an independent firm, \(\pi _{N,O}^{\text {NM}}\). Setting \(\frac {\pi _{N,O}^{M,O}}{ M+N}\geq \pi _{N,O}^{\text {NM}}\), and solving for cost cO, we find that \(\frac { \pi _{N,O}^{M,O}}{M+N}\geq \pi _{N,O}^{\text {NM}}\) holds for all cO ∈ [c1,c2], where,
and
Second, consider (cNO,cO)-pairs in Region II, i.e., \( c_{M,\text {NO}}^{M}\leq c_{O}<c_{N,O}^{M}\). In this region, both firms are active under a merger in the second stage. Therefore, every firm O chooses to merge in the first stage if and only if its share of profits under the merger, \(\frac {\pi _{N,M}^{M,\text {Both}}}{M+N}\), exceeds the profits it would obtain as an independent firm, \(\pi _{N,O}^{\text {NM}}\). Setting \(\frac { \pi _{N,M}^{M,\text {Both}}}{M+N}\geq \pi _{N,O}^{\text {NM}}\), and solving for cost cO, we find that \(\frac {\pi _{N,M}^{M,\text {Both}}}{M+N}\geq \pi _{N,O}^{\text {NM}}\) holds for all cO ∈ [c3,c4], where,
and
Third, consider (cNO,cO)-pairs in Region III, i.e., \(c_{O}\geq c_{N,O}^{M}\). In this region, only firm NO operates under a merger in the second stage. Therefore, every firm O chooses to merge in the first stage if and only if its share of profits under the merger, \(\frac {\pi _{M,\text {NO}}^{M,\text {NO}}}{M+N}\), exceeds the profits, it would obtain as an independent firm, \(\pi _{N,O}^{\text {NM}}\). Setting \(\frac {\pi _{M,\text {NO}}^{M,\text {NO}}}{M+N}\geq \pi _{N,O}^{\text {NM}}\), and solving for cost cO, we find that this inequality holds for all cO ∈ [c5,c6], where,
and
□
Numerical Example
Benchmark Case
We first consider the case when the number of organic and non-organic firms are equal. Applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.79 \ \) and \(c_{\text {NO}}^{M}=0.16\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I cannot be sustained in equilibrium; Region II can be supported for all 0.25 ≤ cO < 0.79; and Region III for all cO ≥ 0.79. From Proposition 1, we obtain cutoffs c1 through c6 (see first row of Table 1). We can then conclude that (1) the range of parameters [c3,c4] = [0.67, 0.99] is compatible with Region II as long as cO ∈ [0.67, 0.79], which entails that this region can be supported in equilibrium when costs are relatively high; and (2) the range of parameters [c5,c6] = [0.68, 0.97] is compatible for all values of cO in Region III, implying that this region can be sustained for all admissible cO.
Then, for the case when the number of non-organic firms are greater than that of organic firms, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.84 \) and \(c_{\text {NO}}^{M}=0.44\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I can only be sustained for all 0.25 ≤ cO < 0.44; Region II can be supported for all 0.44 ≤ cO < 0.84; and Region III for all cO ≥ 0.84. We can then conclude that (1) the range of parameters [c1,c2] = [0.73, 0.85] is incompatible with Region I, and thus this region cannot be sustained in equilibrium; (2) the range of parameters [c3,c4] = [0.71, 0.89] is compatible with Region II as long as cO ∈ [0.71, 0.84], which entails that this region can be supported in equilibrium when costs are relatively high; and (3) the range of parameters [c5,c6] = [0.72, 0.89] is compatible for all values of cO in Region III , implying that this region can be sustained for all admissible cO.
We next examine the previous cutoffs on rows 2–5 of Table 1.
Lower Cost
cNO = 1/10. For the case when the number of organic and non-organic firms are equal, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.71\) and \(c_{\text {NO}}^{M}=-0.13\). Since cO > 0 and cO ≥ cNO by definition, Region I cannot be sustained in equilibrium. Region II can be sustained for all costs satisfying 0.1 ≤ cO < 0.71 and Region III for all cO ≥ 0.71. Proposition 1 implies that (1) the range of parameters [c3,c4] = [0.55, 0.99] is compatible with Region II for all costs cO ∈ [0.55, 0.71], which entails that this region can be supported in equilibrium when costs are moderately high and (2) the range of parameters [c5,c6] = [0.56, 0.96] is compatible for all values of cO in Region III, entailing that this region can be sustained for all admissible cO.
For the second case when the number of non-organic firms are greater than that of organic firms, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.78\) and \(c_{\text {NO}}^{M}=0.24\). Since cO ≥ cNO by definition, cO ≥ 0.1. As a consequence, Region I can be sustained for all costs satisfying 0.1 ≤ cO < 0.24; Region II can be supported for all 0.24 ≤ cO < 0.78; and Region III for all cO ≥ 0.78. Proposition 1 implies that (1) the range of parameters [c1,c2] = [0.64, 0.79] is incompatible with Region I, and thus this region cannot be sustained in equilibrium; (2) the range of parameters [c3,c4] = [0.61, 0.857] is compatible with Region II as long as cO ∈ [0.61, 0.78], which entails that this region can be supported in equilibrium when costs are relatively high; and (3) the range of parameters [c5,c6] = [0.62, 0.854] is compatible for all values of cO in Region III, entailing that this region can be sustained for all admissible cO.
Higher Demand
aNO = 1 . For the case when the number of organic and non-organic firms are equal, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.62\) and \(c_{\text {NO}}^{M}=-0.5\). Since cutoff \( c_{\text {NO}}^{M}<0\) and costs must be positive by definition, Region I cannot be sustained in equilibrium. Region II can be sustained for all costs satisfying 0.25 ≤ cO < 0.62 and Region III for all remaining costs cO ≥ 0.62. Proposition 1 implies that (1) the range of parameters [c3,c4] = [0.41, 0.98] is compatible with Region II as long as cO ∈ [0.41, 0.62] and (2) the range of parameters [c5,c6] = [0.42, 0.95] is compatible for all values of cO in Region III, implying that this region can be sustained for all admissible cO.
For the second case when the number of non-organic firms are greater than that of organic firms, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.71\) and \(c_{\text {NO}}^{M}=0\). Since costs must be positive by definition, Region I cannot be sustained in equilibrium. Region II can be sustained for all costs satisfying 0.25 ≤ cO < 0.71 and Region III for all remaining costs cO ≥ 0.71. Proposition 1 implies that (1) the range of parameters [c3,c4] = [0.49, 0.81] is compatible with Region II as long as cO ∈ [0.49, 0.71] and (2) the range of parameters [c5,c6] = [0.51, 0.80] is compatible for all values of cO in Region III, implying that this region can be sustained for all admissible cO.
Homogeneous Goods
λ = 1. For the case when the number of organic and non-organic firms are equal, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=c_{\text {NO}}^{M}=0.58\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I can be sustained for all costs satisfying 0.25 ≤ cO < 0.58. Region II cannot be sustained since cutoffs coincide \(c_{\text {NO}}^{M}=c_{O}={c_{O}^{M}}\) and Region III can be sustained for all cO ≥ 0.58. Proposition 1 implies that (1) the range of parameters [c1,c2] = [0.60, 0.69] is not compatible in Region I, implying that this region cannot be sustained in equilibrium and (2) the range of parameters [c5,c6] = [0.59, 0.70] is compatible for all values of cO in Region III, implying that this region can be sustained for all admissible cO.
For the second case when the number of non-organic firms are greater than that of organic firms, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.68\) and \(c_{\text {NO}}^{M}=0.72\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I can be sustained for all cost satisfying 0.25 ≤ cO < 0.72. Region II can be supported for all costs satisfying 0.68 ≤ cO < 0.72 and Region III can be sustained for all cO ≥ 0.68. Proposition 1 implies that (1) the range of parameters [c1,c2] = [0.58, 0.65] is compatible in Region I as long as cO ∈ [0.58, 0.72], implying that this region can be sustained in equilibrium; (2) the range of parameters [c3,c4] = [0.60, 0.64] is not compatible with Region II; and (3) the range of parameters [c5,c6] = [0.59, 0.64] is not compatible in Region III, implying that this region cannot be sustained in equilibrium.
λ = 1 and aNO = 1
For the case when the number of organic and non-organic firms are equal, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=c_{\text {NO}}^{M}=0.25\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I cannot be sustained in equilibrium, Region II cannot be sustained since cutoffs \( {c_{O}^{M}}\) and \(c_{\text {NO}}^{M}\) coincide, and Region III can be sustained for all cO ≥ 0.25. Proposition 1 implies that the range of parameters [c5,c6] = [0.27, 0.47] is compatible for all values of cO in Region III, entailing that this region can be sustained for all admissible cO.
For the second case when the number of non-organic firms are greater than that of organic firms, applying Lemma 1 to this parametric example yields cutoffs \({c_{O}^{M}}=0.43\) and \(c_{\text {NO}}^{M}=0.5\). Since cO ≥ cNO by definition, cO ≥ 0.25. As a consequence, Region I can only be sustained for costs satisfying 0.25 ≤ cO < 0.5. Region II can be supported for all costs satisfying 0.43 ≤ cO < 0.5 and Region III can be sustained for all cO ≥ 0.5. Proposition 1 implies that (1) the range of parameters [c1,c2] = [0.25, 0.37] is compatible in Region I, implying that this region can be sustained in equilibrium; (2) the range of parameters [c3,c4] = [0.29, 0.35] is not compatible with Region II; and (3) the range of parameters [c5,c6] = [0.27, 0.36] is not compatible in Region III, implying that this region cannot be sustained in equilibrium.
Proof of Lemma 1
First, note that the profit difference \(\pi ^{M,\text {Both}}-\pi _{O}^{M,O}\) yields a U-shaped curve, which becomes zero at exactly \(c_{O}=a_{O}-\frac { a_{\text {NO}}-c_{\text {NO}}}{\lambda } \). As a consequence, \(\pi ^{M,\text {Both}}\geq \pi _{O}^{M,O}\) holds for all parameter values. For the non-organic firm, the profit difference \(\pi ^{M,\text {Both}}-\pi _{\text {NO}}^{M,\text {NO}}\) exhibits a similar shape, becoming zero at \(c_{O}={c_{O}^{M}}\), thus implying that \(\pi ^{M,\text {Both}}\geq \pi _{\text {NO}}^{M,\text {NO}}\) also holds for all parameter values. Summarizing, it is profitable to maintain both firms active, rather than shutting one of them down. However, conditions \(c_{O}<{c_{O}^{M}}\) and \(c_{\text {NO}}<c_{\text {NO}}^{M}\) still apply yielding different regions in the (cNO,cO)-quadrant.
We can now compare cutoffs \({c_{O}^{M}}\) and \(c_{\text {NO}}^{M}\). First, cutoff \( {c_{O}^{M}}\) originates above \(c_{\text {NO}}^{M}\) since their vertical intercepts satisfy \(a_{O}-\lambda a_{\text {NO}}>a_{O}-\frac {a_{\text {NO}}}{\lambda } \), which holds given that λ ∈ [0, 1] by definition. Second, the positive slope of cutoff \({c_{O}^{M}} \)is λ, whereas that of cutoff \( c_{\text {NO}}^{M}\) is 1/λ, thus indicating that cutoff \(c_{\text {NO}}^{M}\) grows faster than \({c_{O}^{M}}\) does. In addition, cutoffs \({c_{O}^{M}}\) and \( c_{\text {NO}}^{M}\) cross each other at cNO = aNO and a height of cO = aO. Recalling that aO > cO for the organic firm, only three regions can be sustained in the (cNO,cO)-quadrant: (1) when \( c_{O}<c_{\text {NO}}^{M}\), only the organic firm is active; (2) when \(c_{\text {NO}}^{M}\leq c_{O}<{c_{O}^{M}}\), both firms are active; and (3) when \(c_{O}\geq {c_{O}^{M}}\), only the non-organic firm is active.
Proof of Proposition 1
First, consider (cNO,cO)-pairs in Region I, i.e., \(c_{O}<c_{\text {NO}}^{M}\) . In this region, only the organic firm operates under a merger in the second stage. Therefore, the organic firm chooses to merge in the first stage if and only if its share of profits under the merger, \(\frac {\pi _{O}^{M,O}}{2}\), exceeds the profits, it would obtain as an independent firm, \(\pi _{O}^{\text {NM}}\). Setting \(\frac {\pi _{O}^{M,O}}{2}\geq \pi _{O}^{\text {NM}}\), and solving for cost cO, we find that \(\frac {\pi _{O}^{M,O}}{2}\geq \pi _{O}^{\text {NM}}\) holds for all cO ∈ [c1,c2], where,
and
Second, consider (cNO,cO)-pairs in Region II, i.e., \(c_{\text {NO}}^{M}\leq c_{O}<{c_{O}^{M}}\). In this region, both firms are active under a merger in the second stage. Therefore, the organic firm chooses to merge in the first stage if and only if its share of profits under the merger, \(\frac {\pi ^{M,\text {Both}}}{2}\), exceeds the profits, it would obtain as an independent firm, \( \pi _{O}^{\text {NM}}\). Setting \(\frac {\pi ^{M,\text {Both}}}{2}\geq \pi _{O}^{\text {NM}}\), and solving for cost cO, we find that \(\frac {\pi ^{M,\text {Both}}}{2}\geq \pi _{O}^{\text {NM}}\) holds for all cO ∈ [c3,c4], where,
and
Third, consider (cNO,cO)-pairs in Region III, i.e., \(c_{O}\geq {c_{O}^{M}}\). In this region, only the non-organic firm operates under a merger in the second stage. Therefore, the organic firm chooses to merge in the first stage if and only if its share of profits under the merger, \(\frac { \pi _{NO}^{M,\text {NO}}}{2}\), exceeds the profits it would obtain as an independent firm, \(\pi _{O}^{\text {NM}}\). Setting \(\frac {\pi _{\text {NO}}^{M,\text {NO}}}{2}\geq \pi _{O}^{\text {NM}}\) , and solving for cost cO, we find that \(\frac {\pi _{\text {NO}}^{M,\text {NO}}}{2}\geq \pi _{O}^{\text {NM}}\) holds for all cO ∈ [c5,c6], where,
and
Proof of Lemma 2
As discussed in the main body of the paper, social welfare is given by the sum of consumer and producer surplus, W = CS + PS, where \(\text {CS}\equiv \frac {1}{2} ({q_{O}^{2}}+q_{\text {NO}}^{2}+2\lambda q_{O}q_{\text {NO}})\) and PS ≡ [p(qO,qNO)qO − cOqO] + [p(qNO,qO)qNO − cNOqNO]. Using the inverse demands p(qO,qNO) and p(qNO,qO), the expression of producer surplus, PS, collapses to PS = aOqO + qNO(aNO − cNO − qNO) − qO(qO + cO + 2λqNO). Differentiating welfare W with respect to qO, we obtain the following:
and a symmetric expression when we differentiate W with respect to qNO , aNO − cNO − qNO − λqO = 0. Simultaneously solving for qO and qNO, yields \(q_{O}^{\text {SO}}=\frac {a_{O}-c_{O}-\lambda (a_{\text {NO}}-c_{\text {NO}})}{ 1-\lambda ^{2}}\) and \(q_{\text {NO}}^{\text {SO}}=\frac {a_{\text {NO}}-c_{\text {NO}}-\lambda (a_{O}-c_{O})}{ 1-\lambda ^{2}}\).
Comparing \(q_{O}^{\text {SO}}\) against \(q_{O}^{M,\text {Both}}\), we obtain that \( q_{O}^{\text {SO}}\geq q_{O}^{M,\text {Both}}\) for all \(c_{O}\leq {c_{O}^{A}}\equiv \left (a_{O}-\lambda a_{\text {NO}}\right ) +\lambda c_{\text {NO}}\), where the term in parenthesis indicates the vertical intercept of cutoff \({c_{O}^{A}}\) in the (cNO,cO)-quadrant (see Fig. 1 for a reference), while λ represents its positive slope. Similarly, comparing \(q_{O}^{\text {SO}}\) against \( q_{O}^{M,O}\), we obtain that \(q_{O}^{\text {SO}}\geq q_{O}^{M,O}\) for all \(c_{O}\leq {c_{O}^{B}}\equiv \left (a_{O}-\frac {2\lambda a_{\text {NO}}}{1+\lambda ^{2}}\right ) + \frac {2\lambda } {1+\lambda ^{2}}c_{\text {NO}}\), where the term in parenthesis indicates the vertical intercept of cutoff \({c_{O}^{B}}\) in the (cNO,cO)-quadrant (such as that in Fig. 1), while \(\frac {2\lambda } { 1+\lambda ^{2}}\) represents its positive slope. A similar argument for the output levels of the non-organic firm yields that \(q_{\text {NO}}^{\text {SO}}\geq q_{\text {NO}}^{M,\text {NO}}\) if and only if \(c_{O}\leq \left (a_{O}-\frac {\left (1+\lambda ^{2}\right ) a_{\text {NO}}}{2\lambda } \right ) +\frac {\left (1+\lambda ^{2}\right ) c_{\text {NO}}}{2\lambda } \equiv c_{\text {NO}}^{B}\).
Proof of Proposition 2
Let us now analyze each of the regions in Fig. 1. Although we are simultaneously plotting several cutoffs for cO, their ranking does not depend on λ.
As depicted in Fig. 7, cutoffs \({c_{O}^{M}},{c_{O}^{A}}\), and \(c_{O}^{\text {SO}}\) coincide and originate above cutoff \({c_{O}^{B}}\) since \(a_{O}-\lambda a_{\text {NO}}>a_{O}-\frac {2\lambda a_{\text {NO}}}{1+\lambda ^{2}}\), cutoff \({c_{O}^{B}}\) originates above \(c_{\text {NO}}^{B}\) since \(a_{O}-\frac {2\lambda a_{\text {NO}}}{1+\lambda ^{2}}>a_{O}-\frac {\left (1+\lambda ^{2}\right ) a_{\text {NO}}}{2\lambda } \), and cutoff \(c_{\text {NO}}^{B}\) originates above \(c_{\text {NO}}^{M}\) since \(a_{O}-\frac {\left (1+\lambda ^{2}\right ) a_{\text {NO}}}{2\lambda } >a_{O}-\frac {a_{\text {NO}}}{\lambda } \) . Furthermore, cutoffs \({c_{O}^{M}}\) and \(c_{O}^{\text {SO}}\) coincide and originate above cutoffs \(c_{\text {NO}}^{M}\) and \(c_{\text {NO}}^{\text {SO}}\) since \(a_{O}-\lambda a_{\text {NO}}>a_{O}-\frac {a_{\text {NO}}}{\lambda } \).
(in the Appendix)
Starting at Region I, where \(c_{O}<c_{\text {NO}}^{M}\), only the organic firm is active in equilibrium. The social planner would also have only the organic firm being active since in this region cO satisfies \(c_{O}<c_{O}^{\text {SO}}\) and \(c_{O}<c_{\text {NO}}^{\text {SO}}\). We can then compare equilibrium and socially optimal output, \(q_{O}^{M,O}\) and \(q_{O}^{\text {SO}}\), obtaining that \( q_{O}^{\text {SO}}\geq q_{O}^{M,O}\) since Region I lies entirely below cutoff \( {c_{O}^{B}}\). Therefore, a socially insufficient output emerges in Region I, relative to the social optimum.
In Region II, where \(c_{\text {NO}}^{M}\leq c_{O}< {c_{O}^{M}}\), both firms are active in equilibrium where \(q_{O}^{M,\text {Both}},q_{\text {NO}}^{M,\text {Both}}>0\). The social planner would also recommend that both firms are active since \(c_{\text {NO}}^{\text {SO}}\leq c_{O}< c_{O}^{\text {SO}}\). Comparing equilibrium and optimal output, we obtain that \(q_{O}^{\text {SO}}\geq q_{O}^{M,\text {Both}}\) since \(c_{O}\leq {c_{O}^{A}}\). Therefore, a socially insufficient output emerges in Region II.
In Region III, only the non-organic firm is active in equilibrium, which coincides with the social optimum since \(c_{O}\geq c_{O}^{\text {SO}}\) and \( c_{O}\geq c_{\text {NO}}^{\text {SO}}\). Comparing output levels, we find that \( q_{\text {NO}}^{\text {SO}}<q_{\text {NO}}^{M,\text {NO}}\) since Region III lies entirely above cutoff \( c_{\text {NO}}^{B}\). Therefore, a socially excessive output emerges for all costs in Region III.
In summary, socially insufficient production occurs in two regions: (a) Region I, where \(c_{O}<c_{\text {NO}}^{M}=c_{\text {NO}}^{\text {SO}}\); (b) Region II, where \( c_{\text {NO}}^{M}\leq c_{O}<{c_{O}^{M}}=c_{O}^{\text {SO}}\). Furthermore, we can collapse the conditions to just \(c_{O}<c_{O}^{\text {SO}}\). Socially excessive production occurs in Region III, where \(c_{O}\geq {c_{O}^{M}}=c_{O}^{\text {SO}}\).
Proof of Corollary 1
Undifferentiated Products
λ = 1. In this context, all cutoffs collapse to the same line (i.e., they are all originating at aO − aNO), dividing into two regions, Region I and Region III, as depicted in Fig. 8.
(in the Appendix): Cutoffs when λ = 1
Starting at Region I, where \(c_{O}<c_{\text {NO}}^{M}\), only the organic firm is active in equilibrium. The social planner would also have only the organic firm being active since in this region cO satisfies \(c_{O}<c_{O}^{\text {SO}}\) and \(c_{O}<c_{\text {NO}}^{\text {SO}}\). Comparing equilibrium and socially optimal output, we obtain that \(q_{O}^{\text {SO}}\geq q_{O}^{M,O}\) since Region I lies entirely below cutoff \({c_{O}^{B}}\). As a result, a socially insufficient output emerges in Region I.
In Region III, only the non-organic firm is active in equilibrium. The social planner would also have only the non-organic firm being active since cO satisfies \(c_{O}\geq c_{\text {NO}}^{\text {SO}}\). Comparing output levels, we find that \(q_{\text {NO}}^{\text {SO}}<q_{\text {NO}}^{M,\text {NO}}\) since Region III lies entirely above \( c_{\text {NO}}^{B}\). Therefore, a socially excessive output emerges in Region III.
Completely Differentiated Products
λ = 0. As depicted in Fig. 9, in this setting, all cutoffs collapse to the same line (i.e., they are all horizontal line originating at aO), yielding only one possible outcome: Region I, where the organic firm is the only active plant if \(c_{O}<c_{\text {NO}}^{M}\).
(in the Appendix): Cutoffs when λ = 0
In Region I, where \(c_{O}<c_{\text {NO}}^{M}\), only the organic firm is active in equilibrium. The social planner would also have only the organic firm being active since in this region cO satisfies \(c_{O}<c_{O}^{\text {SO}}\) and \( c_{O}<c_{\text {NO}}^{\text {SO}}\). We can then compare equilibrium and socially optimal output, \(q_{O}^{M,O}\) and \(q_{O}^{\text {SO}}\), obtaining that \(q_{O}^{\text {SO}}\geq q_{O}^{M,O}\) since Region I lies entirely below cutoff \({c_{O}^{B}}\). Therefore, a socially insufficient output emerges in Region I, relative to the social optimum.
Rights and permissions
About this article
Cite this article
Espínola-Arredondo, A., Munoz-Garcia, F. & Jung, A.R. Organic Mergers and Acquisitions. J Ind Compet Trade 20, 59–91 (2020). https://doi.org/10.1007/s10842-019-00300-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10842-019-00300-9