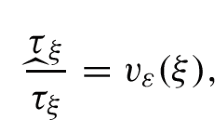Skip to main content
SpringerLink
Log in

Detection and construction of an elliptic solution of the complex cubic-quintic Ginzburg-Landau equation

  • Published:
Theoretical and Mathematical Physics Aims and scope Submit manuscript

Abstract

In evolution equations for a complex amplitude, the equation for the phase is much more intricate than for the amplitude. Nevertheless, general methods should be applicable to both variables. In the example of the traveling-wave reduction of the complex cubic-quintic Ginzburg-Landau (CGL5) equation, we explain how to overcome the difficulties arising in two methods: (1) the criterion that the sum of residues of an elliptic solution is zero and (2) the construction of a first-order differential equation admitting a given equation as a differential consequence (subequation method).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. I. S. Aranson and L. Kramer, Rev. Modern Phys., 74, 99–143 (2002); arXiv:cond-mat/0106115v1 (2001).

    Article  MathSciNet  ADS  MATH  Google Scholar 

  2. W. van Saarloos, Phys. Rep., 386, 29–222 (2003); arXiv:cond-mat/0308540v2 (2003).

    Article  ADS  MATH  Google Scholar 

  3. A. V. Klyachkin, “Modulational instability and autowaves in the active media described by the nonlinear equations of Ginzburg-Landau type,” Preprint No. 1338, Ioffe Phys. Tech. Inst., Leningrad (1989).

    Google Scholar 

  4. M. Musette and R. Conte, Phys. D, 181, 70–79 (2003); arXiv:nlin.PS/0302051v1 (2003).

    Article  MathSciNet  MATH  Google Scholar 

  5. R. Conte and M. Musette, The Painlevé Handbook, Springer, Berlin (2008).

    MATH  Google Scholar 

  6. R. Conte and M. Musette, Stud. Appl. Math., 123, 63–81 (2009); arXiv:0903.2009v1 [math.CA] (2009).

    Article  MathSciNet  MATH  Google Scholar 

  7. J. Chazy, Acta Math., 34, 317–385 (1911).

    Article  MathSciNet  MATH  Google Scholar 

  8. P. Marcq, H. Chaté, and R. Conte, Phys. D, 73, 305–317 (1994); arXiv:patt-sol/9310004v1 (1993).

    Article  MathSciNet  MATH  Google Scholar 

  9. A. N. W. Hone, Phys. D, 205, 292–306 (2005).

    Article  MathSciNet  MATH  Google Scholar 

  10. S. Yu. Vernov, J. Phys. A, 40, 9833–9844 (2007); arXiv:nlin/0602060v2 (2006).

    Article  MathSciNet  ADS  MATH  Google Scholar 

  11. C. Briot and J.-C. Bouquet, Théorie des fonctions elliptiques (1st ed.), Mallet-Bachelier, Paris (1859); Gauthier-Villars, Paris (1875).

    Google Scholar 

  12. R. Conte and T. W. Ng, “Meromorphic traveling wave solutions of the complex cubic-quintic Ginzburg-Landau equation,” Acta Appl. Math. (in press 2012).

  13. G.-H. Halphen, Traité des fonctions elliptiques et de leurs applications (http://gallica.bnf.fr/document?O=N007348), Gauthier-Villars, Paris (1890).

    MATH  Google Scholar 

  14. S. Popp, O. Stiller, I. Aranson, and L. Kramer, Phys. D, 84, 398–423 (1995).

    Article  MathSciNet  Google Scholar 

  15. H. Chaté, Nonlinearity, 7, 185–204 (1994).

    Article  MathSciNet  ADS  MATH  Google Scholar 

  16. J. Swift and P. C. Hohenberg, Phys. Rev. A, 15, 319–328 (1977).

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. LRC MESO, Centre de mathématiques et de leurs applications et CEA-DAM, École normale supérieure de Cachan, Cachan, France

    R. Conte & Tuen-Wai Ng

  2. Department of Mathematics, Faculty of Science, The University of Hong Kong, Hong Kong, China

    R. Conte

Authors
  1. R. Conte
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tuen-Wai Ng
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to R. Conte.

Additional information

Prepared from an English manuscript submitted by the authors; for the Russian version, see Teoreticheskaya i Matematicheskaya Fizika, Vol. 172, No. 2, pp. 224–235, August, 2012.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Conte, R., Ng, TW. Detection and construction of an elliptic solution of the complex cubic-quintic Ginzburg-Landau equation. Theor Math Phys 172, 1073–1084 (2012). https://doi.org/10.1007/s11232-012-0096-4

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11232-012-0096-4

Keywords

Search

Navigation