Abstract
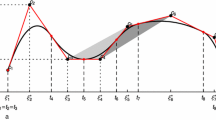
This begins the study of a Riemannian generalization of a special case of algorithm I of Lane and Riesenfeld (1980), closely related to the de Casteljau algorithm (Goldman, 1989) for generating cubic polynomial curves. In our version, as in Shoemake's (1985), straight lines are replaced by geodesic segments. Our construction differs from Shoemake's in that it is a kind of stationary subdivision algorithm, defined by a recursive procedure, and it is not at all clear from the construction that a limiting curve q ∞ exists, much less that it is differentiable. Indeed, the aim of the present paper is to prove that q ∞ is differentiable and that the derivative is Lipschitz. The result is nontrivial: it is well‐known that stationary subdivision typically defines non‐differentiable curves (Cavaretta et al., 1991). On the other hand Shoemake's algorithm is non‐recursive and evidently defines a C ∞ curve. Other approaches to splines on curved spaces are considered in (Barr et al., 1992; Chapman and Noakes, 1991; Duff, 1985; Gabriel and Kajiya, 1985; Noakes et al., 1989).
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
A.H. Barr, B. Currin, S. Gabriel and J.F. Hughes, Smooth interpolation of orientations with angular velocity constraints using quaternions, Computer Graphics 26(2) (1992) 313–320.
A.S. Cavaretta, W. Dahmen and C.A. Micchelli, Stationary subdivision, Mem. Amer. Math. Soc. 93(453) (September 1991).
P.B. Chapman and J.L. Noakes, Singular perturbations and interpolation – a problem in robotics, Nonlinear Anal. 16 (1991) 849–859.
G. de Rham, Sur quelques fonctions différentiables dont toutes les valeurs sont des valeurs critiques, in: Celebrazioni Archimedee del Secolo XX, Siracusa, II (11–16 April 1961) pp. 61–65.
G. de Rham, Un peu mathématiques á propos d'une courbe plane, Revue de Mathématiques Élémentaires, II (1947).
G. de Rham, Sur certaines équations fonctionelles, in: Ouvrage Publié á L'Occasion de son Centenaire par l'École Polytechnique de l'Université de Lausanne (1953) pp. 95–97.
G. de Rham, Sur une courbe plane, J. Math. Pures Appl. 35 (1956) 25–42.
G. de Rham, Sur les courbes limites de polygones obtenus par trisection, L'Enseignement Mathématique 5 (1959) 29–43.
T. Duff, Quaternion splines for animating orientations, in: Second Computer Graphics Workshop, Monterey, CA, USA, 12–13 December, 1985 (Usenix Association, 1985) pp. 54–62.
S.A. Gabriel and J.T. Kajiya, Spline interpolation in curved manifolds (1985, unpublished).
S. Gallot, D. Hulin and J. Lafontaine, Riemannian Geometry, Universitext (Springer, Berlin, 2nd ed., 1990).
R.N. Goldman, Recursive triangles, in: Computation of Curves and Surfaces, eds. W. Dahmen, M. Gasca and C.A. Micchelli, NATO Adv. Sci. Inst. Ser. C Math. Phys. Sci. 307 (Kluwer, Dordrecht, 1989) pp. 27–72.
J.M. Lane and R.F. Riesenfeld, A theoretical development for the computer generation and display of piecewise polynomial curves and surfaces, IEEE Trans. PAMI 2(1) (1980) 35–46.
J.W. Milnor, Morse Theory, Ann. of Math. Stud. 51 (Princeton University Press, Princeton, NJ, 1963).
L. Noakes, H. Greg and B. Paden, Cubic splines on curved spaces, IMA J. Math. Control Inform. 6 (1989) 465–473.
K. Shoemake, Animating rotation with quaternion curves, SIGGRAPH 19(3) (1985) 245–254.
H. von Koch, Sur une courbe continue sans tangente obtenue par une construction géometrique élémentaire, Arkiv. Mat., Astronomik och Fysik 1 (1904) 681–702.
J.H.C. Whitehead, Convex regions in the geometry of paths, Quart. J. Math. Oxford Ser. (2) 3 (1932) 33–42.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Noakes, L. Nonlinear corner‐cutting. Advances in Computational Mathematics 8, 165–177 (1998). https://doi.org/10.1023/A:1018940112654
Issue Date:
DOI: https://doi.org/10.1023/A:1018940112654